Question Number 98623 by hardylanes last updated on 15/Jun/20
$${evaluate}\: \\ $$$$\int_{\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}} ^{\mathrm{2}} \frac{\mathrm{1}}{{x}^{\mathrm{2}} \sqrt{\mathrm{4}+{x}^{\mathrm{2}} }}{dx}\:{using}\:{the}\:{substitution}\:{x}=\mathrm{2tan}\theta \\ $$$$ \\ $$
Answered by Kunal12588 last updated on 15/Jun/20
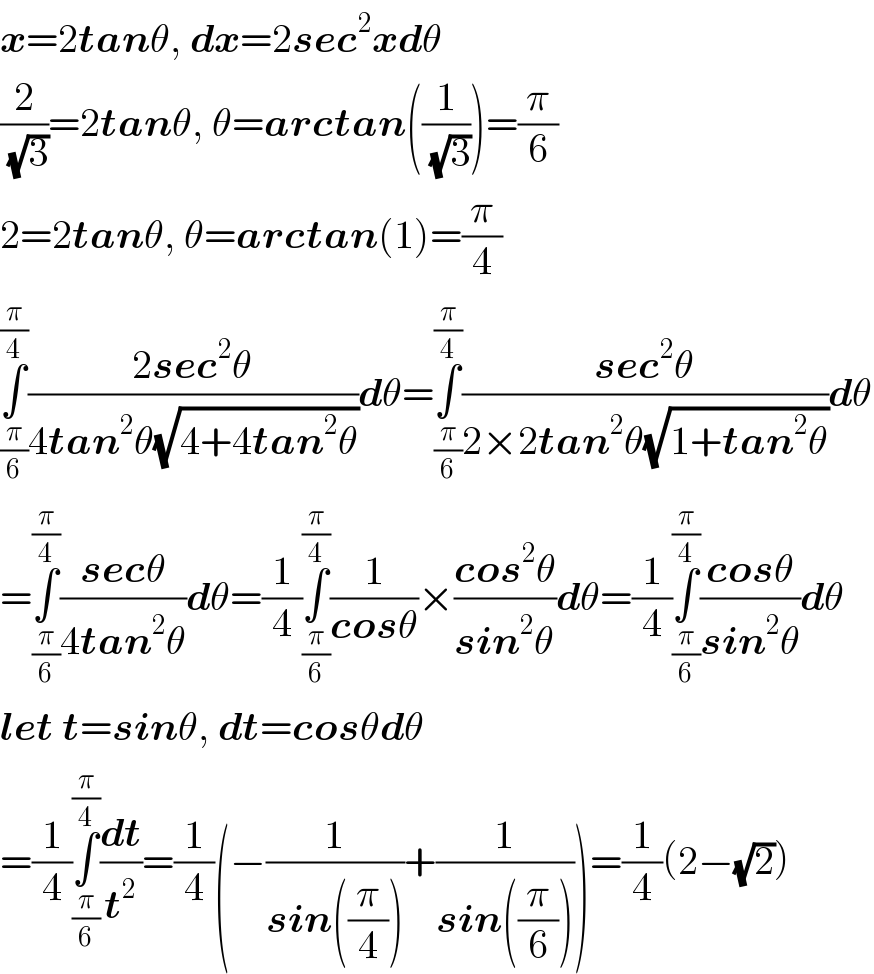
![x=2tanθ ⇒dx=2sec^2 θ dθ θ=tan^(−1) ((x/2)); ∫_( π/6) ^( π/4) ((2sec^2 θ)/(4tan^2 θ(√(4+4tan^2 θ))))dθ ∫_( π/6) ^( π/4) ((secθ)/(4tan^2 θ))dθ (1/4)∫_( π/6) ^( π/4) ((cosθ)/(sin^2 θ))dθ (1/4)∫_( π/6) ^( π/4) ((d(sinθ))/(sin^2 θ)) (1/4)[−(1/(sin θ))]_(π/6) ^(π/4) =(1/4)(−(√2)+2)=((2−(√2))/4)](https://www.tinkutara.com/question/Q98627.png)
$${x}=\mathrm{2}{tan}\theta \\ $$$$\Rightarrow{dx}=\mathrm{2}{sec}^{\mathrm{2}} \theta\:{d}\theta \\ $$$$\theta={tan}^{−\mathrm{1}} \left(\frac{{x}}{\mathrm{2}}\right);\: \\ $$$$\int_{\:\pi/\mathrm{6}} ^{\:\pi/\mathrm{4}} \frac{\mathrm{2}{sec}^{\mathrm{2}} \theta}{\mathrm{4}{tan}^{\mathrm{2}} \theta\sqrt{\mathrm{4}+\mathrm{4}{tan}^{\mathrm{2}} \theta}}{d}\theta \\ $$$$\int_{\:\pi/\mathrm{6}} ^{\:\pi/\mathrm{4}} \frac{{sec}\theta}{\mathrm{4}{tan}^{\mathrm{2}} \theta}{d}\theta \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\int_{\:\pi/\mathrm{6}} ^{\:\pi/\mathrm{4}} \frac{{cos}\theta}{{sin}^{\mathrm{2}} \theta}{d}\theta \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\int_{\:\pi/\mathrm{6}} ^{\:\pi/\mathrm{4}} \frac{{d}\left({sin}\theta\right)}{{sin}^{\mathrm{2}} \theta} \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\left[−\frac{\mathrm{1}}{{sin}\:\theta}\right]_{\pi/\mathrm{6}} ^{\pi/\mathrm{4}} =\frac{\mathrm{1}}{\mathrm{4}}\left(−\sqrt{\mathrm{2}}+\mathrm{2}\right)=\frac{\mathrm{2}−\sqrt{\mathrm{2}}}{\mathrm{4}} \\ $$
Commented by hardylanes last updated on 15/Jun/20
thanks
Commented by abdomathmax last updated on 15/Jun/20
$$\mathrm{your}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{correct}\:\mathrm{miss}\:\mathrm{kunal} \\ $$
Commented by Kunal12588 last updated on 16/Jun/20
$${it}'{s}\:{mister}! \\ $$
Answered by MWSuSon last updated on 15/Jun/20
$$\boldsymbol{{x}}=\mathrm{2}\boldsymbol{{tan}}\theta,\:\boldsymbol{{dx}}=\mathrm{2}\boldsymbol{{sec}}^{\mathrm{2}} \boldsymbol{{xd}}\theta \\ $$$$\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}=\mathrm{2}\boldsymbol{{tan}}\theta,\:\theta=\boldsymbol{{arctan}}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right)=\frac{\pi}{\mathrm{6}} \\ $$$$\mathrm{2}=\mathrm{2}\boldsymbol{{tan}}\theta,\:\theta=\boldsymbol{{arctan}}\left(\mathrm{1}\right)=\frac{\pi}{\mathrm{4}} \\ $$$$\underset{\frac{\pi}{\mathrm{6}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\frac{\mathrm{2}\boldsymbol{{sec}}^{\mathrm{2}} \theta}{\mathrm{4}\boldsymbol{{tan}}^{\mathrm{2}} \theta\sqrt{\mathrm{4}+\mathrm{4}\boldsymbol{{tan}}^{\mathrm{2}} \theta}}\boldsymbol{{d}}\theta=\underset{\frac{\pi}{\mathrm{6}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\frac{\boldsymbol{{sec}}^{\mathrm{2}} \theta}{\mathrm{2}×\mathrm{2}\boldsymbol{{tan}}^{\mathrm{2}} \theta\sqrt{\mathrm{1}+\boldsymbol{{tan}}^{\mathrm{2}} \theta}}\boldsymbol{{d}}\theta \\ $$$$=\underset{\frac{\pi}{\mathrm{6}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\frac{\boldsymbol{{sec}}\theta}{\mathrm{4}\boldsymbol{{tan}}^{\mathrm{2}} \theta}\boldsymbol{{d}}\theta=\frac{\mathrm{1}}{\mathrm{4}}\underset{\frac{\pi}{\mathrm{6}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\frac{\mathrm{1}}{\boldsymbol{{cos}}\theta}×\frac{\boldsymbol{{cos}}^{\mathrm{2}} \theta}{\boldsymbol{{sin}}^{\mathrm{2}} \theta}\boldsymbol{{d}}\theta=\frac{\mathrm{1}}{\mathrm{4}}\underset{\frac{\pi}{\mathrm{6}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\frac{\boldsymbol{{cos}}\theta}{\boldsymbol{{sin}}^{\mathrm{2}} \theta}\boldsymbol{{d}}\theta \\ $$$$\boldsymbol{{let}}\:\boldsymbol{{t}}=\boldsymbol{{sin}}\theta,\:\boldsymbol{{dt}}=\boldsymbol{{cos}}\theta\boldsymbol{{d}}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\underset{\frac{\pi}{\mathrm{6}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\frac{\boldsymbol{{dt}}}{\boldsymbol{{t}}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{4}}\left(−\frac{\mathrm{1}}{\boldsymbol{{sin}}\left(\frac{\pi}{\mathrm{4}}\right)}+\frac{\mathrm{1}}{\boldsymbol{{sin}}\left(\frac{\pi}{\mathrm{6}}\right)}\right)=\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{2}−\sqrt{\mathrm{2}}\right) \\ $$
Commented by hardylanes last updated on 15/Jun/20
thankiu
Answered by abdomathmax last updated on 15/Jun/20
![let I =∫_(2/( (√3))) ^2 (dx/(x^2 (√(4+x^2 )))) let do the changement x =2sht ⇒t =argsh((x/2)) ⇒ I = ∫_(ln((1/( (√3)))+(√(1+(1/3))))) ^(ln(1+(√2))) ((2ch(t)dt)/(4sh^2 t(2cht))) = ∫_(ln((√3))) ^(ln(1+(√2))) (dt/(4(((ch(2t)−1)/2)))) =(1/2) ∫_(ln((√3))) ^(ln(1+(√2))) (dt/(((e^(2t) +e^(−2t) )/2)−1)) =∫_(ln((√3))) ^(ln(1+(√2))) (dt/(e^(2t) +e^(−2t) −2)) =_(e^(2t) =u) ∫_3 ^((1+(√2))^2 ) (du/(2u(u +u^(−1) −2))) =∫_3 ^(3+2(√2)) (du/(2(u^2 +1−2u))) =(1/2) ∫_3 ^(3+2(√2)) (du/((u−1)^2 )) =(1/2)[−(1/(u−1))]_3 ^(3+2(√2)) =(1/2){(1/2)−(1/(2+2(√2)))} =(1/4){1−(1/(1+(√2)))} =(1/4){((√2)/(1+(√2)))} =(1/4)×(((√2)((√2)−1))/1) =(1/4)(2−(√2)) =(1/2)−((√2)/4)](https://www.tinkutara.com/question/Q98662.png)
$$\mathrm{let}\:\mathrm{I}\:=\int_{\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}} ^{\mathrm{2}} \:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} \sqrt{\mathrm{4}+\mathrm{x}^{\mathrm{2}} }}\:\:\mathrm{let}\:\mathrm{do}\:\mathrm{the}\:\mathrm{changement}\: \\ $$$$\mathrm{x}\:=\mathrm{2sht}\:\Rightarrow\mathrm{t}\:=\mathrm{argsh}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)\:\Rightarrow \\ $$$$\mathrm{I}\:=\:\int_{\mathrm{ln}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}+\sqrt{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}}\right)} ^{\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)} \:\frac{\mathrm{2ch}\left(\mathrm{t}\right)\mathrm{dt}}{\mathrm{4sh}^{\mathrm{2}} \mathrm{t}\left(\mathrm{2cht}\right)}\:\: \\ $$$$=\:\int_{\mathrm{ln}\left(\sqrt{\mathrm{3}}\right)} ^{\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)} \:\:\frac{\mathrm{dt}}{\mathrm{4}\left(\frac{\mathrm{ch}\left(\mathrm{2t}\right)−\mathrm{1}}{\mathrm{2}}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{ln}\left(\sqrt{\mathrm{3}}\right)} ^{\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)} \:\:\frac{\mathrm{dt}}{\frac{\mathrm{e}^{\mathrm{2t}} +\mathrm{e}^{−\mathrm{2t}} }{\mathrm{2}}−\mathrm{1}} \\ $$$$=\int_{\mathrm{ln}\left(\sqrt{\mathrm{3}}\right)} ^{\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)} \:\:\:\frac{\mathrm{dt}}{\mathrm{e}^{\mathrm{2t}} \:+\mathrm{e}^{−\mathrm{2t}} −\mathrm{2}} \\ $$$$=_{\mathrm{e}^{\mathrm{2t}} \:=\mathrm{u}} \:\:\:\:\int_{\mathrm{3}} ^{\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{2}} } \:\:\:\:\frac{\mathrm{du}}{\mathrm{2u}\left(\mathrm{u}\:+\mathrm{u}^{−\mathrm{1}} −\mathrm{2}\right)} \\ $$$$=\int_{\mathrm{3}} ^{\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}} \:\:\:\:\:\frac{\mathrm{du}}{\mathrm{2}\left(\mathrm{u}^{\mathrm{2}} +\mathrm{1}−\mathrm{2u}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{3}} ^{\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}} \:\:\frac{\mathrm{du}}{\left(\mathrm{u}−\mathrm{1}\right)^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{2}}\left[−\frac{\mathrm{1}}{\mathrm{u}−\mathrm{1}}\right]_{\mathrm{3}} ^{\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left\{\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}+\mathrm{2}\sqrt{\mathrm{2}}}\right\}\:=\frac{\mathrm{1}}{\mathrm{4}}\left\{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{1}+\sqrt{\mathrm{2}}}\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\left\{\frac{\sqrt{\mathrm{2}}}{\mathrm{1}+\sqrt{\mathrm{2}}}\right\}\:=\frac{\mathrm{1}}{\mathrm{4}}×\frac{\sqrt{\mathrm{2}}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)}{\mathrm{1}}\:=\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{2}−\sqrt{\mathrm{2}}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}−\frac{\sqrt{\mathrm{2}}}{\mathrm{4}} \\ $$