evaluate-lim-n-1-e-x-n-ln-x-dx-

Question Number 100215 by Rio Michael last updated on 25/Jun/20

evaluate \lim_{n \to \infty} \int_{1}^{e} x^{n} \ln x d x

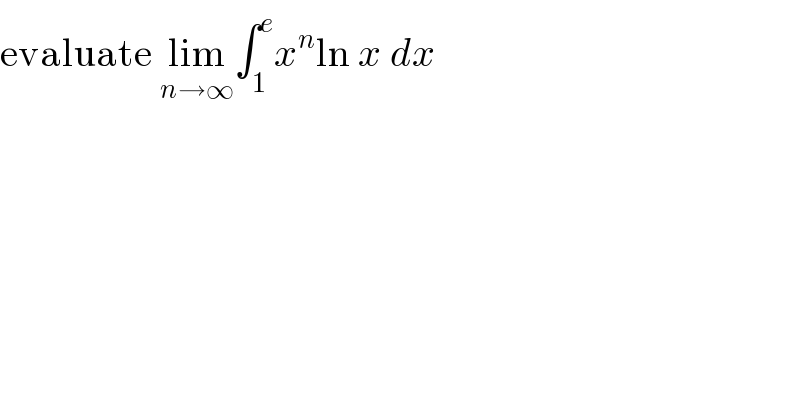
Commented by Dwaipayan Shikari last updated on 25/Jun/20

\infty

Answered by Ar Brandon last updated on 25/Jun/20

Let I = \int_{1}^{e} x^{n} lnxdx

let {\begin{cases} u = lnx \\ dv = x^{n} dx \end{cases} \Rightarrow {\begin{cases} du = \frac{1}{x} dx \\ v = \frac{x^{n + 1}}{n + 1} \end{cases}

\Rightarrow I = {[\frac{x^{n + 1}}{n + 1} \cdot lnx]}_{1}^{e} - \frac{1}{n + 1} \int_{1}^{e} x^{n} dx = \frac{e^{n + 1}}{n + 1} - {[\frac{x^{n + 1}}{{(n + 1)}^{2}}]}_{1}^{e}

\Rightarrow I = \frac{e^{n + 1}}{n + 1} - \frac{e^{n + 1}}{{(n + 1)}^{2}} + \frac{1}{{(n + 1)}^{2}}

\lim_{n \to \infty} (I) = \lim_{n \to \infty} {\frac{e^{n + 1}}{1} - \frac{e^{n + 1}}{2 (n + 1)}} = \lim_{n \to \infty} {e^{n + 1} - \frac{e^{n + 1}}{2}}

= \lim_{n \to \infty} {\frac{e^{n + 1}}{2}} = + \infty

Commented by DGmichael last updated on 25/Jun/20

�� LIATE

Commented by Ar Brandon last updated on 25/Jun/20

Ouais�� c'est ça.

Answered by mathmax by abdo last updated on 25/Jun/20

A_{n} = \int_{1}^{e} x^{n} lnx dx changement lnx = t give A_{n} = \int_{0}^{1} e^{nt} t e^{t} dt

= \int_{0}^{1} t e^{(n + 1) t} dt = {[\frac{1}{n + 1} t e^{(n + 1) t}]}_{0}^{1} - \int_{0}^{1} \frac{1}{n + 1} e^{(n + 1) t} dt

= \frac{e^{n + 1}}{n + 1} - \frac{1}{{(n + 1)}^{2}} {[e^{) n + 1) t}]}_{0}^{1} = \frac{e^{n + 1}}{n + 1} - \frac{1}{{(n + 1)}^{2}} (e^{n + 1} - 1)

= \frac{e^{n + 1}}{n + 1} - \frac{e^{n + 1}}{{(n + 1)}^{2}} + \frac{1}{{(n + 1)}^{2}} = \frac{(n + 1) e^{n + 1} - e^{n + 1}}{{(n + 1)}^{2}} + \frac{1}{{(n + 1)}^{2}} = \frac{{ne}^{n + 1}}{{(n + 1)}^{2}} + \frac{1}{{(n + 1)}^{2}} \Rightarrow

\lim_{n \to + \infty} A_{n} = \lim_{n \to + \infty} \frac{{ne}^{n + 1}}{{(n + 1)}^{2}} = + \infty