Question Number 92805 by niroj last updated on 09/May/20
$$\:\mathrm{Evaluate}: \\ $$$$\:\int_{\boldsymbol{\mathrm{R}}} \int\:\frac{\boldsymbol{\mathrm{xy}}}{\:\sqrt{\mathrm{1}−\boldsymbol{\mathrm{y}}^{\mathrm{2}} }}\:\boldsymbol{\mathrm{dx}}\:\boldsymbol{\mathrm{dy}}\:\mathrm{where}\:\mathrm{the}\:\mathrm{region}\:\mathrm{of}\:\mathrm{integration}\:\mathrm{is}\:\mathrm{the} \\ $$$$\:\mathrm{positive}\:\mathrm{quadrant}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circle}\:\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\boldsymbol{\mathrm{y}}^{\mathrm{2}} =\mathrm{1}. \\ $$$$ \\ $$
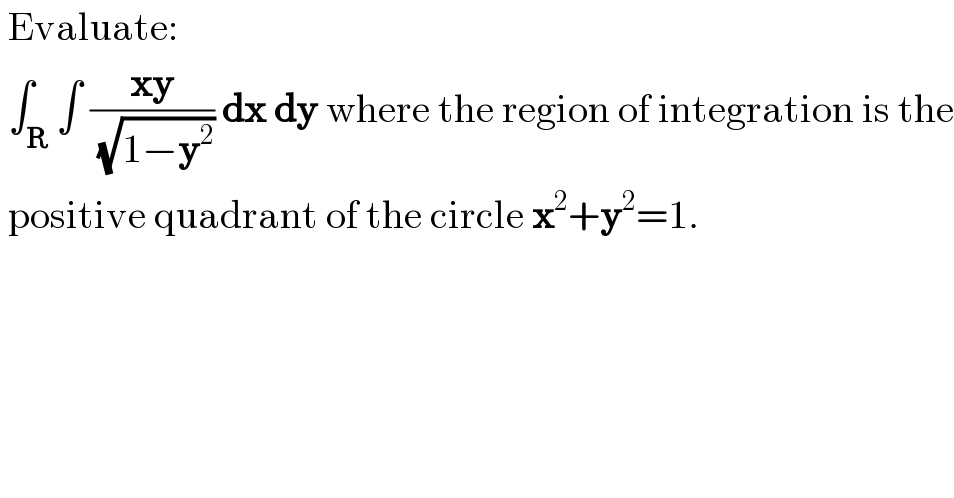
Answered by mr W last updated on 09/May/20
![=∫_0 ^(π/2) ∫_0 ^1 ((r^2 cos θ sin θ)/( (√(1−r^2 sin^2 θ))))rdrdθ =(1/2)∫_0 ^(π/2) cos θ sin θ(∫_0 ^1 (r^2 /( (√(1−r^2 sin^2 θ))))dr^2 )dθ =(1/2)∫_0 ^(π/2) cos θ sin θ(∫_0 ^1 (u/( (√(1−u sin^2 θ))))du)dθ =(1/2)∫_0 ^(π/2) cos θ sin θ(1/(sin^2 θ)){∫_0 ^1 [−(√(1−u sin^2 θ))+(1/( (√(1−u sin^2 θ))))]du}dθ =(1/2)∫_0 ^(π/2) ((cos θ)/(sin θ)){[(2/(3 sin^2 θ))(1−u sin^2 θ)^(3/2) −(2/(sin^2 θ))(√(1−u sin^2 θ))]_0 ^1 }dθ =(1/2)∫_0 ^(π/2) ((cos θ)/(sin θ)){[(2/(3 sin^2 θ))(1−sin^2 θ)^(3/2) −(2/(3 sin^2 θ))−(2/(sin^2 θ))(√(1−sin^2 θ))+(2/(sin^2 θ))]}dθ =(1/3)∫_0 ^(π/2) ((cos θ)/(sin θ))[((cos^3 θ−3cos θ+2)/(sin^2 θ))]dθ =(1/3)∫_(π/2) ^0 (((cos^3 θ−3cos θ+2)cos θ)/((1−cos^2 θ)^2 ))d(cos θ) =(1/3)∫_0 ^1 (((t^3 −3t+2)t)/((1−t^2 )^2 ))dt =(1/3)∫_0 ^1 (((t+2)t)/((t+1)^2 ))dt =(1/3)∫_0 ^1 [1−(1/((t+1)^2 ))]dt =(1/3)[t+(1/(t+1))]_0 ^1 =(1/3)(1+(1/2)−1) =(1/6)](https://www.tinkutara.com/question/Q92811.png)
$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \int_{\mathrm{0}} ^{\mathrm{1}} \frac{{r}^{\mathrm{2}} \mathrm{cos}\:\theta\:\mathrm{sin}\:\theta}{\:\sqrt{\mathrm{1}−{r}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta}}{rdrd}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{cos}\:\theta\:\mathrm{sin}\:\theta\left(\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{r}^{\mathrm{2}} }{\:\sqrt{\mathrm{1}−{r}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta}}{dr}^{\mathrm{2}} \right){d}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{cos}\:\theta\:\mathrm{sin}\:\theta\left(\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{u}}{\:\sqrt{\mathrm{1}−{u}\:\mathrm{sin}^{\mathrm{2}} \:\theta}}{du}\right){d}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{cos}\:\theta\:\mathrm{sin}\:\theta\frac{\mathrm{1}}{\mathrm{sin}^{\mathrm{2}} \:\theta}\left\{\int_{\mathrm{0}} ^{\mathrm{1}} \left[−\sqrt{\mathrm{1}−{u}\:\mathrm{sin}^{\mathrm{2}} \:\theta}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{u}\:\mathrm{sin}^{\mathrm{2}} \:\theta}}\right]{du}\right\}{d}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\left\{\left[\frac{\mathrm{2}}{\mathrm{3}\:\mathrm{sin}^{\mathrm{2}} \:\theta}\left(\mathrm{1}−{u}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right)^{\frac{\mathrm{3}}{\mathrm{2}}} −\frac{\mathrm{2}}{\mathrm{sin}^{\mathrm{2}} \:\theta}\sqrt{\mathrm{1}−{u}\:\mathrm{sin}^{\mathrm{2}} \:\theta}\right]_{\mathrm{0}} ^{\mathrm{1}} \right\}{d}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\left\{\left[\frac{\mathrm{2}}{\mathrm{3}\:\mathrm{sin}^{\mathrm{2}} \:\theta}\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:\theta\right)^{\frac{\mathrm{3}}{\mathrm{2}}} −\frac{\mathrm{2}}{\mathrm{3}\:\mathrm{sin}^{\mathrm{2}} \:\theta}−\frac{\mathrm{2}}{\mathrm{sin}^{\mathrm{2}} \:\theta}\sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:\theta}+\frac{\mathrm{2}}{\mathrm{sin}^{\mathrm{2}} \:\theta}\right]\right\}{d}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\left[\frac{\mathrm{cos}^{\mathrm{3}} \:\theta−\mathrm{3cos}\:\theta+\mathrm{2}}{\mathrm{sin}^{\mathrm{2}} \:\theta}\right]{d}\theta \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{0}} \frac{\left(\mathrm{cos}^{\mathrm{3}} \:\theta−\mathrm{3cos}\:\theta+\mathrm{2}\right)\mathrm{cos}\:\theta}{\left(\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \:\theta\right)^{\mathrm{2}} }{d}\left(\mathrm{cos}\:\theta\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left({t}^{\mathrm{3}} −\mathrm{3}{t}+\mathrm{2}\right){t}}{\left(\mathrm{1}−{t}^{\mathrm{2}} \right)^{\mathrm{2}} }{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left({t}+\mathrm{2}\right){t}}{\left({t}+\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \left[\mathrm{1}−\frac{\mathrm{1}}{\left({t}+\mathrm{1}\right)^{\mathrm{2}} }\right]{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\left[{t}+\frac{\mathrm{1}}{{t}+\mathrm{1}}\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{6}} \\ $$
Commented by niroj last updated on 09/May/20
Thank you Mr. W It is aspected result ��������
Commented by Ar Brandon last updated on 09/May/20
��