Question Number 185665 by mathlove last updated on 25/Jan/23

$${f}\left(\frac{\mathrm{2}{x}−\mathrm{1}}{\mathrm{3}{x}+\mathrm{2}}\right)=\frac{{x}−\mathrm{1}}{{x}−\mathrm{2}}\:\:\:\:\:\:{faind}\:\:\:{f}^{−\mathrm{1}} \left(\mathrm{2}\right)=? \\ $$
Answered by cortano1 last updated on 25/Jan/23
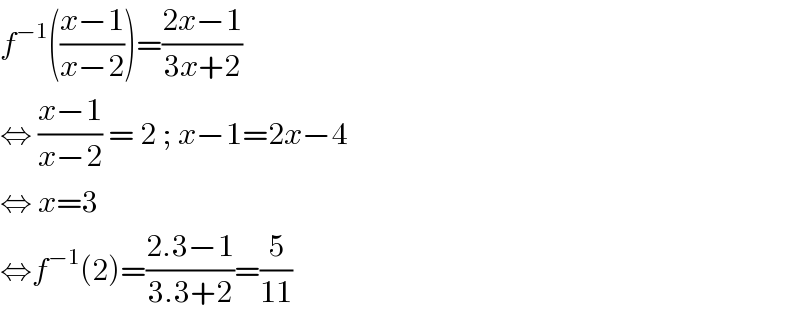
$${f}^{−\mathrm{1}} \left(\frac{{x}−\mathrm{1}}{{x}−\mathrm{2}}\right)=\frac{\mathrm{2}{x}−\mathrm{1}}{\mathrm{3}{x}+\mathrm{2}}\: \\ $$$$\Leftrightarrow\:\frac{{x}−\mathrm{1}}{{x}−\mathrm{2}}\:=\:\mathrm{2}\:;\:{x}−\mathrm{1}=\mathrm{2}{x}−\mathrm{4} \\ $$$$\Leftrightarrow\:{x}=\mathrm{3}\: \\ $$$$\Leftrightarrow{f}^{−\mathrm{1}} \left(\mathrm{2}\right)=\frac{\mathrm{2}.\mathrm{3}−\mathrm{1}}{\mathrm{3}.\mathrm{3}+\mathrm{2}}=\frac{\mathrm{5}}{\mathrm{11}} \\ $$
