f-x-1-1-sin-x-1-cos-x-find-Min-f-x-

Question Number 149667 by mnjuly1970 last updated on 06/Aug/21

f (x) = \frac{1}{\sqrt{1 + s i n (x)} + \sqrt{1 + c o s (x)}}

f i n d :

Min (f (x)) = ?

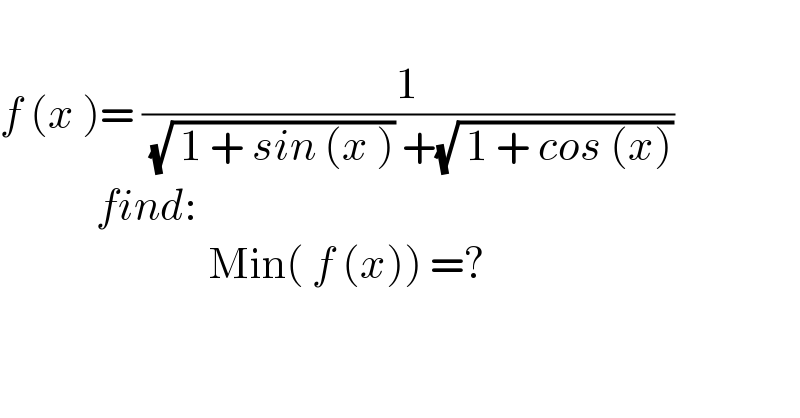
Answered by iloveisrael last updated on 07/Aug/21

f (x) = \frac{1}{∣ \cos \frac{1}{2} x + \sin \frac{1}{2} x ∣ + \sqrt{2} ∣ \cos \frac{1}{2} x ∣}

f (x) = \frac{1}{\sqrt{2} ∣ \sin (\frac{1}{2} x + \frac{π}{4}) ∣ + \sqrt{2} ∣ \cos \frac{1}{2} x ∣}

when \cos \frac{1}{2} x = 1

or \frac{1}{2} x = 0

f_{1} = \frac{1}{\sqrt{2} \sin (\frac{π}{4}) + \sqrt{2}} = \frac{1}{\sqrt{2} + 1} = \sqrt{2} - 1

when \sin (\frac{1}{2} x + \frac{π}{4}) = 1

or \frac{1}{2} x = \frac{π}{4}

f_{2} = \frac{1}{\sqrt{2} + \sqrt{2} (\frac{1}{\sqrt{2}})} = \frac{1}{\sqrt{2} + 1} = \sqrt{2} - 1

f (x) = \sqrt{2} - 1

when \frac{1}{2} x = \frac{π}{8} we get

f_{3} = \frac{1}{\sqrt{2} {∣ \sin \frac{3 π}{8} ∣ + ∣ \cos \frac{π}{8} ∣}}

\sin \frac{3 π}{8} = \sqrt{\frac{1 - \cos \frac{3 π}{4}}{2}} = \sqrt{\frac{1 + \frac{\sqrt{2}}{2}}{2}} = \sqrt{\frac{2 + \sqrt{2}}{4}} = \frac{\sqrt{2 + \sqrt{2}}}{2}

\cos \frac{π}{8} = \sqrt{\frac{1 + \cos \frac{π}{4}}{2}} = \sqrt{\frac{2 + \sqrt{2}}{4}} = \frac{\sqrt{2 + \sqrt{2}}}{2}

f {(x)}_{\min} = f_{3} = \frac{1}{\sqrt{2} {\frac{\sqrt{2 + \sqrt{2}}}{2} + \frac{\sqrt{2 + \sqrt{2}}}{2}}}

= \frac{1}{\sqrt{2} (\sqrt{2 + \sqrt{2}})}

Commented by mnjuly1970 last updated on 06/Aug/21

t h x m a s t e r \dots

Commented by mnjuly1970 last updated on 06/Aug/21

m i n ? \frac{1}{\sqrt{2} (\sqrt{2 + \sqrt{2}})}

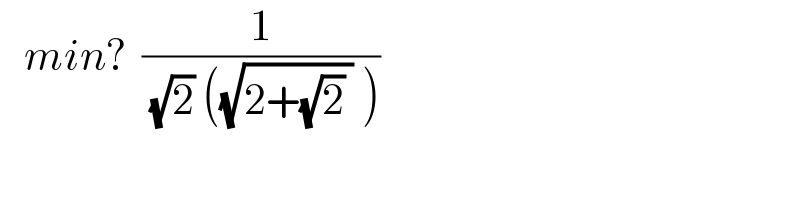
Answered by EDWIN88 last updated on 07/Aug/21

f (x) = \frac{1}{\sqrt{1 + \sin x} + \sqrt{1 + \cos x}}

let g (x) = \sqrt{1 + \sin x} + \sqrt{1 + \cos x}

t h e n f (x) = \frac{1}{g (x)}, f {(x)}_{m i n} i t

m u s t b e g {(x)}_{m a x}

\Rightarrow t a k e g^{'} (x) = \frac{\cos x}{2 \sqrt{1 + \sin x}} - \frac{\sin x}{2 \sqrt{1 + \cos x}} = 0

\Rightarrow \cos x \sqrt{1 + \cos x} = \sin x \sqrt{1 + \sin x}

\Rightarrow \cos^{2} x + \cos^{3} x = \sin^{2} x + \sin^{3} x

\Rightarrow \cos^{2} x - \sin^{2} x = \sin^{3} x - \cos^{3} x

\Rightarrow (\cos x - \sin x) (\cos x + \sin x) = - (\cos x - \sin x) (1 + \sin x \cos x)

\Rightarrow (\cos x - \sin x) {\cos x + \sin x + 1 + \sin x \cos x) = 0

w h e n \cos x = \sin x \Rightarrow x = \frac{π}{4}

g {(x)}_{m a x} = \sqrt{1 + \sin \frac{π}{4}} + \sqrt{1 + \cos \frac{π}{4}}

g {(x)}_{m a x} = \sqrt{\frac{2 + \sqrt{2}}{2}} + \sqrt{\frac{2 + \sqrt{2}}{2}}

g {(x)}_{m a x} = 2 \sqrt{\frac{2 + \sqrt{2}}{2}} = \sqrt{2} (\sqrt{2 + \sqrt{2}})

t h e r e f o r e f {(x)}_{m i n} = \frac{1}{\sqrt{2} (\sqrt{2 + \sqrt{2}})}

Facebook Tweet Pin