Menu Close

f-x-f-x-1-x-2-2x-f-6-33-faind-volue-of-f-50-

Tinku Tara 0 Comments
Pin




Question Number 177146 by mathlove last updated on 01/Oct/22
f(x)=f(x−1)+x^2 +2x f(6)=33 faind volue of f(50)=?
$${f}\left({x}\right)={f}\left({x}−\mathrm{1}\right)+{x}^{\mathrm{2}} +\mathrm{2}{x} \\ $$$${f}\left(\mathrm{6}\right)=\mathrm{33}\:\:{faind}\:{volue}\:{of}\:\:{f}\left(\mathrm{50}\right)=? \\ $$
Answered by cortano1 last updated on 01/Oct/22
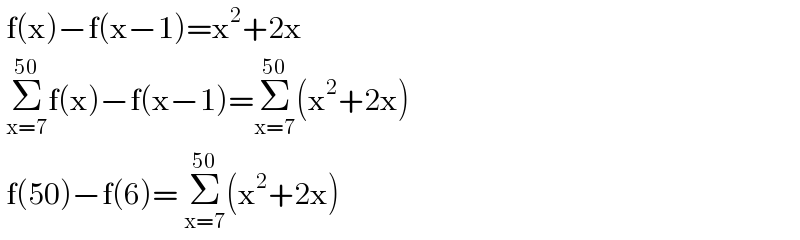
f(x)−f(x−1)=x^2 +2x Σ_(x=7) ^(50) f(x)−f(x−1)=Σ_(x=7) ^(50) (x^2 +2x) f(50)−f(6)= Σ_(x=7) ^(50) (x^2 +2x)
$$\:\mathrm{f}\left(\mathrm{x}\right)−\mathrm{f}\left(\mathrm{x}−\mathrm{1}\right)=\mathrm{x}^{\mathrm{2}} +\mathrm{2x} \\ $$$$\:\underset{\mathrm{x}=\mathrm{7}} {\overset{\mathrm{50}} {\sum}}\mathrm{f}\left(\mathrm{x}\right)−\mathrm{f}\left(\mathrm{x}−\mathrm{1}\right)=\underset{\mathrm{x}=\mathrm{7}} {\overset{\mathrm{50}} {\sum}}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{2x}\right) \\ $$$$\:\mathrm{f}\left(\mathrm{50}\right)−\mathrm{f}\left(\mathrm{6}\right)=\:\underset{\mathrm{x}=\mathrm{7}} {\overset{\mathrm{50}} {\sum}}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{2x}\right) \\ $$
Answered by mr W last updated on 01/Oct/22
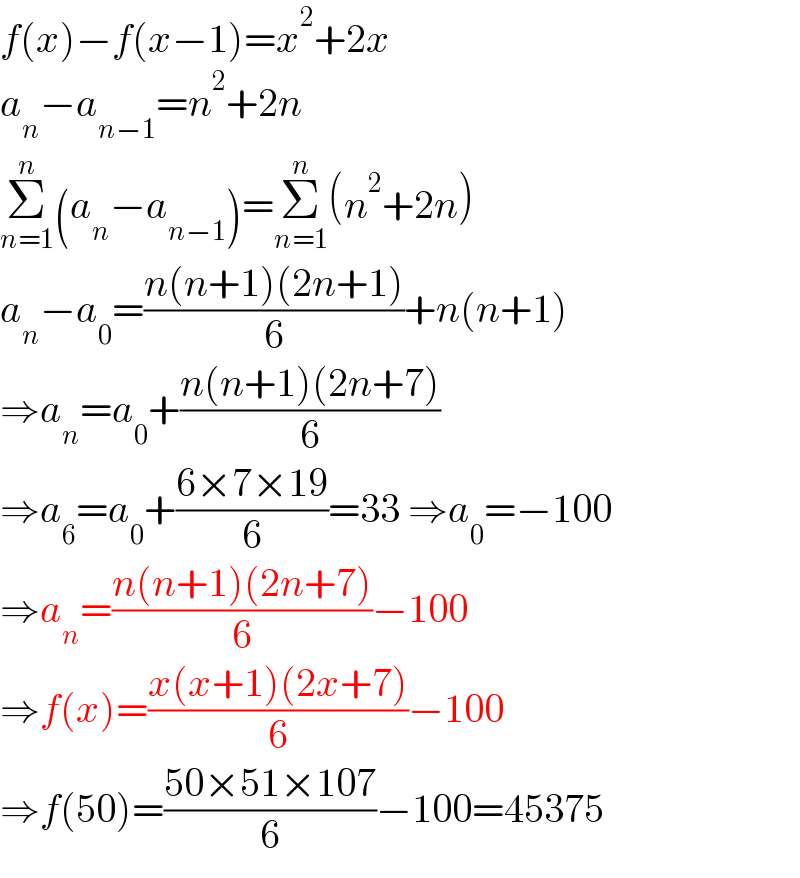
f(x)−f(x−1)=x^2 +2x a_n −a_(n−1) =n^2 +2n Σ_(n=1) ^n (a_n −a_(n−1) )=Σ_(n=1) ^n (n^2 +2n) a_n −a_0 =((n(n+1)(2n+1))/6)+n(n+1) ⇒a_n =a_0 +((n(n+1)(2n+7))/6) ⇒a_6 =a_0 +((6×7×19)/6)=33 ⇒a_0 =−100 ⇒a_n =((n(n+1)(2n+7))/6)−100 ⇒f(x)=((x(x+1)(2x+7))/6)−100 ⇒f(50)=((50×51×107)/6)−100=45375
$${f}\left({x}\right)−{f}\left({x}−\mathrm{1}\right)={x}^{\mathrm{2}} +\mathrm{2}{x} \\ $$$${a}_{{n}} −{a}_{{n}−\mathrm{1}} ={n}^{\mathrm{2}} +\mathrm{2}{n} \\ $$$$\underset{{n}=\mathrm{1}} {\overset{{n}} {\sum}}\left({a}_{{n}} −{a}_{{n}−\mathrm{1}} \right)=\underset{{n}=\mathrm{1}} {\overset{{n}} {\sum}}\left({n}^{\mathrm{2}} +\mathrm{2}{n}\right) \\ $$$${a}_{{n}} −{a}_{\mathrm{0}} =\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{6}}+{n}\left({n}+\mathrm{1}\right) \\ $$$$\Rightarrow{a}_{{n}} ={a}_{\mathrm{0}} +\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{7}\right)}{\mathrm{6}} \\ $$$$\Rightarrow{a}_{\mathrm{6}} ={a}_{\mathrm{0}} +\frac{\mathrm{6}×\mathrm{7}×\mathrm{19}}{\mathrm{6}}=\mathrm{33}\:\Rightarrow{a}_{\mathrm{0}} =−\mathrm{100} \\ $$$$\Rightarrow{a}_{{n}} =\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{7}\right)}{\mathrm{6}}−\mathrm{100} \\ $$$$\Rightarrow{f}\left({x}\right)=\frac{{x}\left({x}+\mathrm{1}\right)\left(\mathrm{2}{x}+\mathrm{7}\right)}{\mathrm{6}}−\mathrm{100} \\ $$$$\Rightarrow{f}\left(\mathrm{50}\right)=\frac{\mathrm{50}×\mathrm{51}×\mathrm{107}}{\mathrm{6}}−\mathrm{100}=\mathrm{45375} \\ $$
Commented by mathlove last updated on 02/Oct/22
thanks mr
$${thanks}\:{mr} \\ $$
Commented by Tawa11 last updated on 02/Oct/22
Great sir
$$\mathrm{Great}\:\mathrm{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *