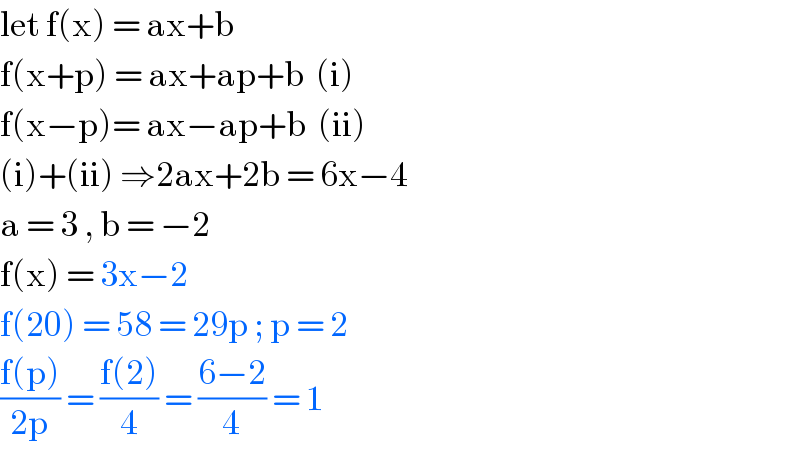Question Number 95662 by john santu last updated on 26/May/20
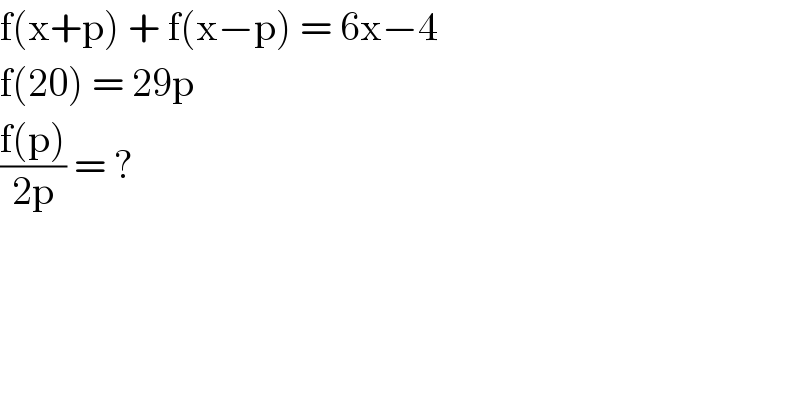
Commented by i jagooll last updated on 26/May/20
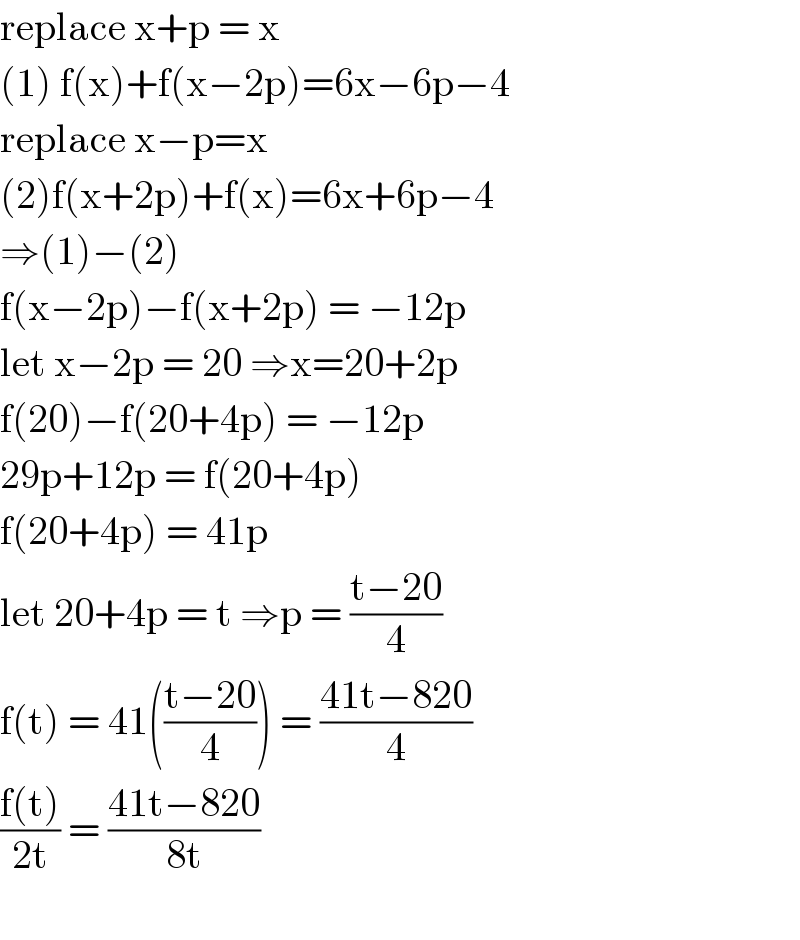
Commented by PRITHWISH SEN 2 last updated on 26/May/20
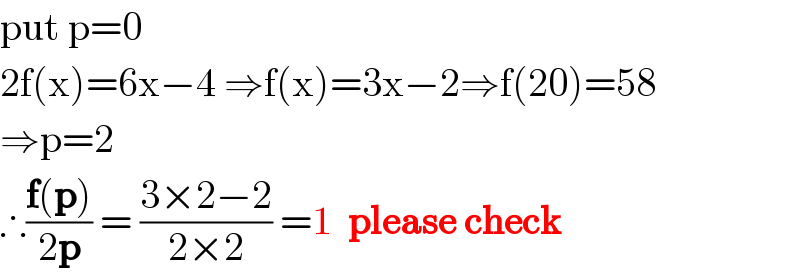
Commented by i jagooll last updated on 27/May/20
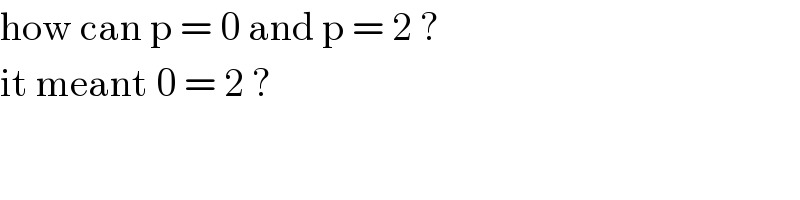
Commented by PRITHWISH SEN 2 last updated on 27/May/20
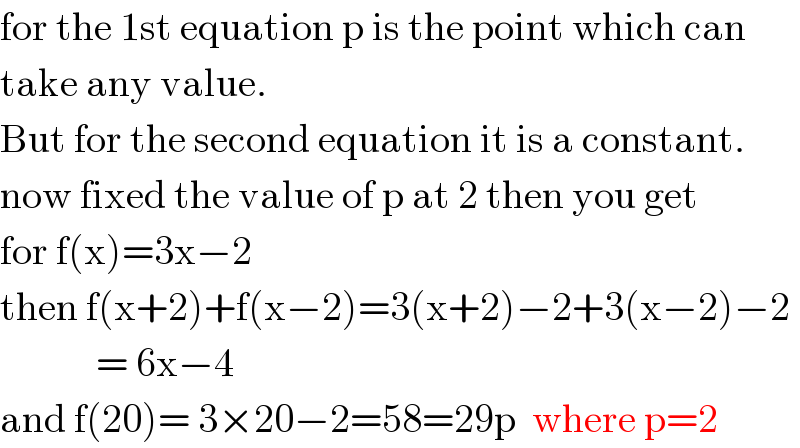
Commented by i jagooll last updated on 27/May/20
Answered by john santu last updated on 28/May/20