f-x-sin-x-sec-x-1-xtan-x-find-f-x-

Question Number 25034 by chernoaguero@gmail.com last updated on 02/Dec/17

f (x) = \frac{\sin x + \sec x}{1 + xtan x}

find f^{'} (x)

Answered by prakash jain last updated on 02/Dec/17

\frac{d}{d x} \frac{u}{v} = \frac{u^{'} v - v^{'} u}{v^{2}} =

\frac{d}{d x} \frac{\sin x + \sec x}{1 + xtan x}

= \frac{{(\sin x + \sec x)}^{'} (1 + x \tan x) - {(1 + x \tan x)}^{'} (\sin x + \sec x)}{{(1 + x \tan x)}^{2}}

= \frac{(\cos x + \sec x \tan x) (1 + x \tan x) - (x \sec^{2} x + \tan x) (\sin x + \sec x)}{{(1 + x \tan x)}^{2}}

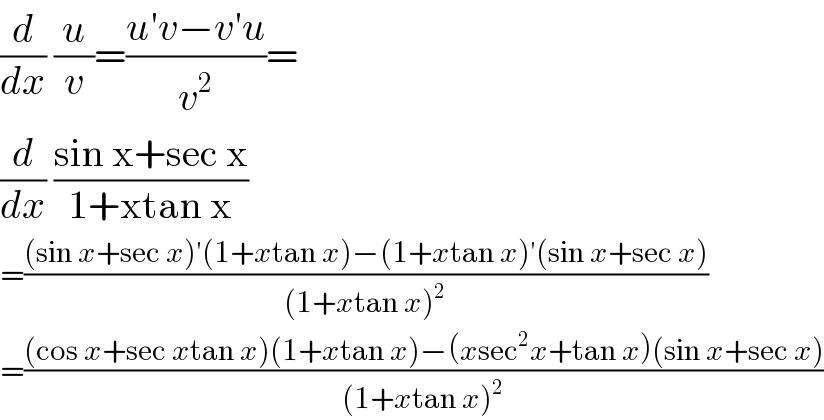
Commented by chernoaguero@gmail.com last updated on 02/Dec/17

Sir is there any trigonometric identity between them

Commented by prakash jain last updated on 02/Dec/17

I {didn}^{'} t simplify it further but will do

it later today .

Commented by chernoaguero@gmail.com last updated on 02/Dec/17

Ok sir

Answered by ajfour last updated on 02/Dec/17

y (1 + x \tan x) = \sin x + \frac{1}{\cos x}

\Rightarrow y (\cot x + x) = \cos x + cosec x

f^{'} (x) (\cot x + x) - y \cot^{2} x =

- \sin x - cosec x \cot x

f^{'} (x) (\cot x + x) = \frac{(\cos x + cosec x) \cot^{2} x}{(\cot x + x)}

- (\sin x + cosec x \cot x)

f^{'} (x) = \frac{\cos x \cot^{2} x - \cos x - x \sin x - x cosec x \cot x}{{(\cot x + x)}^{2}} .

Commented by chernoaguero@gmail.com last updated on 02/Dec/17

Thank u vry much sir