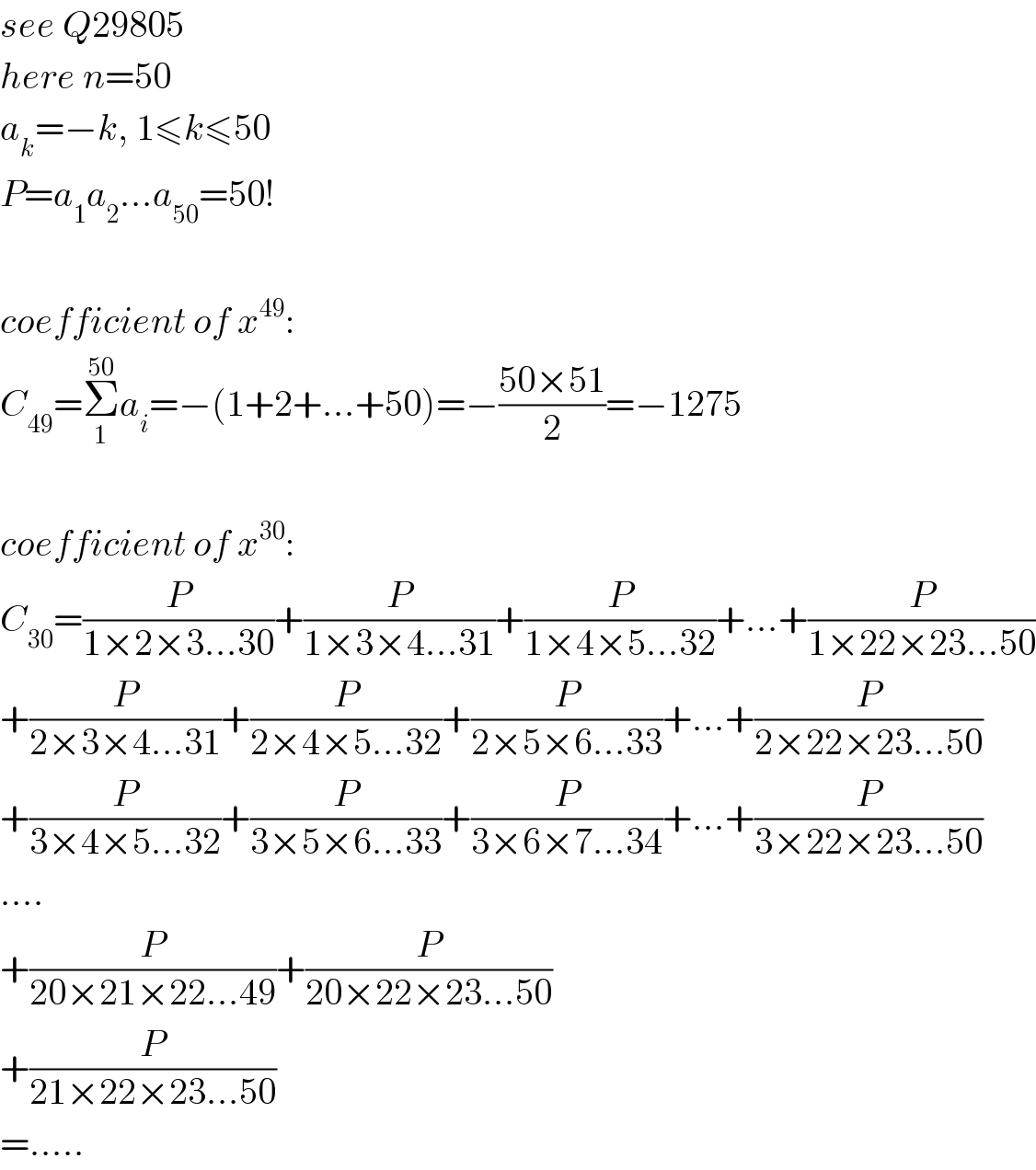
Question Number 29777 by Joel578 last updated on 12/Feb/18
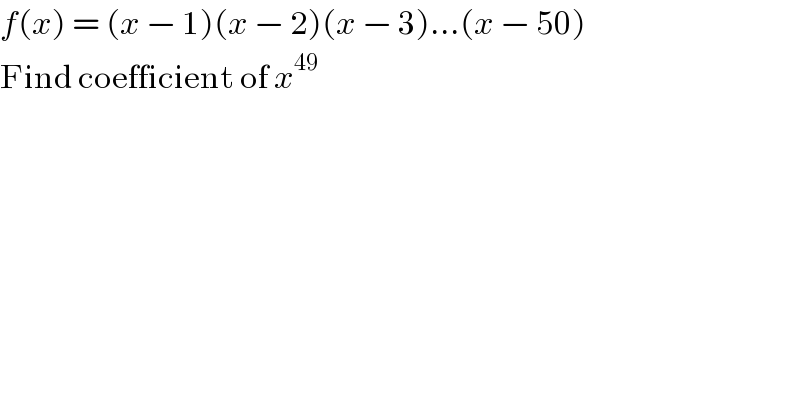
Commented by mrW2 last updated on 12/Feb/18

Commented by 803jaideep@gmail.com last updated on 12/Feb/18
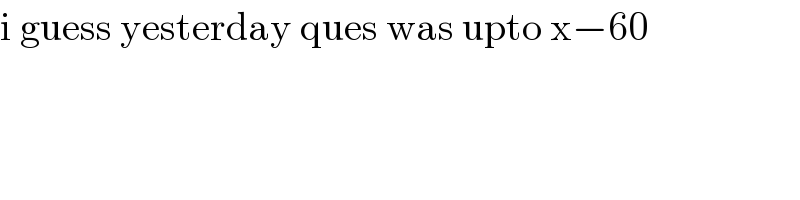
Commented by Joel578 last updated on 12/Feb/18
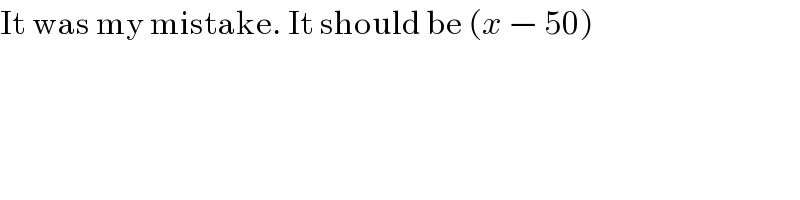
Commented by mrW2 last updated on 12/Feb/18
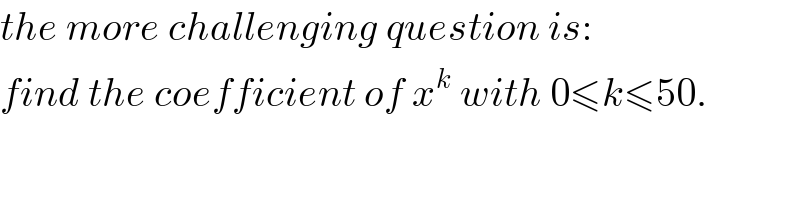
Commented by 803jaideep@gmail.com last updated on 12/Feb/18

Answered by puneet1789 last updated on 12/Feb/18
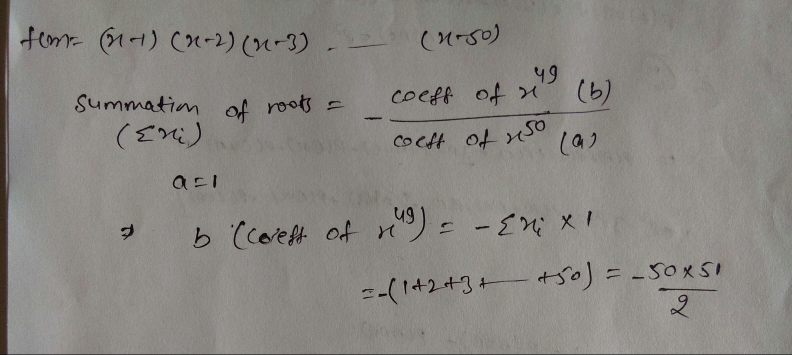
Commented by Joel578 last updated on 12/Feb/18
Answered by mrW2 last updated on 13/Feb/18