Menu Close

Find-0-x-ln-x-x-2-1-dx-

Tinku Tara 0 Comments
Pin




Question Number 155479 by mathdanisur last updated on 01/Oct/21
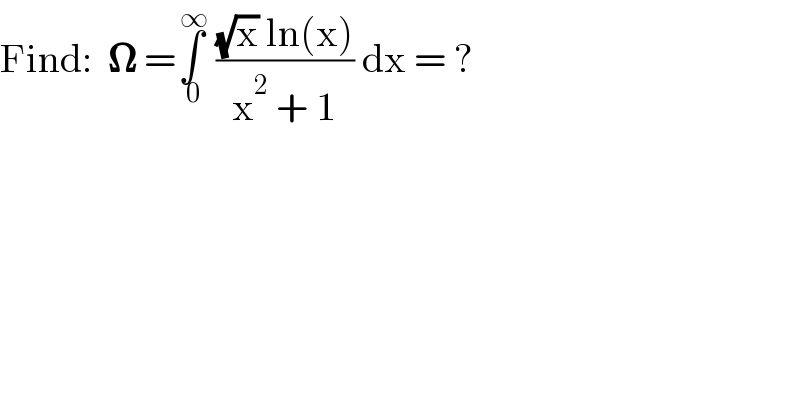
Find: 𝛀 =∫_( 0) ^( ∞) (((√x) ln(x))/(x^2 + 1)) dx = ?
$$\mathrm{Find}:\:\:\boldsymbol{\Omega}\:=\underset{\:\mathrm{0}} {\overset{\:\infty} {\int}}\:\frac{\sqrt{\mathrm{x}}\:\mathrm{ln}\left(\mathrm{x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\:\mathrm{1}}\:\mathrm{dx}\:=\:? \\ $$
Commented by mathdanisur last updated on 01/Oct/21
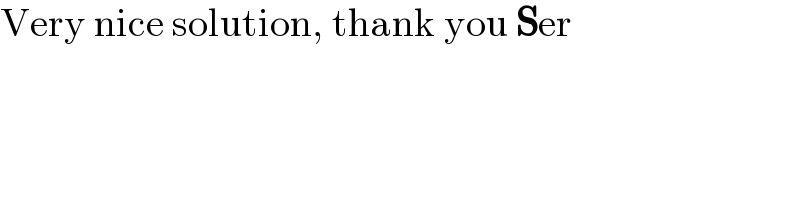
Very nice solution, thank you Ser
$$\mathrm{Very}\:\mathrm{nice}\:\mathrm{solution},\:\mathrm{thank}\:\mathrm{you}\:\boldsymbol{\mathrm{S}}\mathrm{er} \\ $$
Commented by aliyn last updated on 01/Oct/21
𝛀 = ∫_0 ^( ∞) (((√( x)) ln(x))/(x^2 +1)) dx Solution: 𝛀 = Re ( ∫_0 ^( ∞) (((√( x)) ln(x))/(x^2 +1)) dx ) x^2 +1 = 0 β‡’ x = Β± i Res(f,βˆ’i) = lim_(xβ†’βˆ’i) (x+i) Γ— (((√x) ln(x))/((xβˆ’i)(x+i))) = (((√(βˆ’i)) ln(βˆ’i))/(βˆ’2i)) Since: (√(βˆ’i)) = (βˆ’i)^(1/2) = (e^(βˆ’i(𝛑/2)) )^(1/2) = e^(βˆ’i(𝛑/4)) = cos((𝛑/4)) βˆ’ i sin((𝛑/4)) = (1/( (√2))) βˆ’ i (1/( (√2))) ln(βˆ’i) = ln ( e^(βˆ’i(𝛑/2)) ) = βˆ’ i (𝛑/2) ∴ (√(βˆ’i)) ln(βˆ’i) = βˆ’ i (𝛑/(2 (√( 2)))) βˆ’ (𝛑/(2 (√( 2)))) ∴ 𝛀 = Re ( 2𝛑i Γ—Res( f,βˆ’i) )= ((βˆ’((2𝛑^2 )/(2(√( 2)))) βˆ’ i ((2𝛑^2 )/(2 (√2))))/(βˆ’ 2 i)) = (𝛑^2 /( 2 (√( 2)))) ∴ ∴ 𝛀 = (𝛑^2 /(2 (√( 2)))) ⟨ M . T ⟩
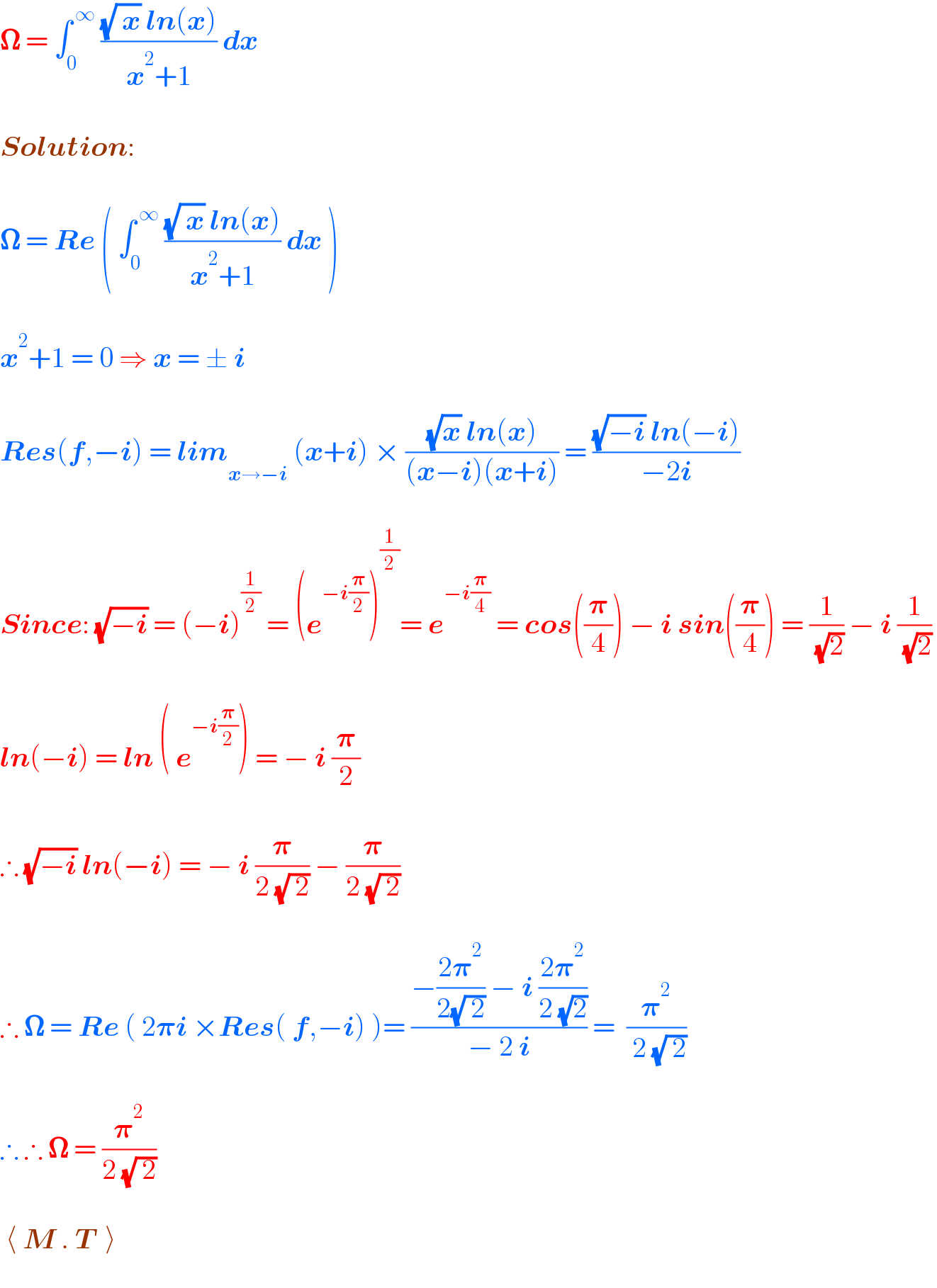
$$\boldsymbol{\Omega}\:=\:\int_{\mathrm{0}} ^{\:\infty} \:\frac{\sqrt{\:\boldsymbol{{x}}}\:\boldsymbol{{ln}}\left(\boldsymbol{{x}}\right)}{\boldsymbol{{x}}^{\mathrm{2}} +\mathrm{1}}\:\boldsymbol{{dx}} \\ $$$$ \\ $$$$\boldsymbol{{Solution}}: \\ $$$$ \\ $$$$\boldsymbol{\Omega}\:=\:\boldsymbol{{Re}}\:\left(\:\int_{\mathrm{0}} ^{\:\infty} \:\frac{\sqrt{\:\boldsymbol{{x}}}\:\boldsymbol{{ln}}\left(\boldsymbol{{x}}\right)}{\boldsymbol{{x}}^{\mathrm{2}} +\mathrm{1}}\:\boldsymbol{{dx}}\:\right) \\ $$$$ \\ $$$$\boldsymbol{{x}}^{\mathrm{2}} +\mathrm{1}\:=\:\mathrm{0}\:\Rightarrow\:\boldsymbol{{x}}\:=\:\pm\:\boldsymbol{{i}}\: \\ $$$$ \\ $$$$\boldsymbol{{Res}}\left(\boldsymbol{{f}},βˆ’\boldsymbol{{i}}\right)\:=\:\boldsymbol{{lim}}_{\boldsymbol{{x}}\rightarrowβˆ’\boldsymbol{{i}}} \:\left(\boldsymbol{{x}}+\boldsymbol{{i}}\right)\:Γ—\:\frac{\sqrt{\boldsymbol{{x}}}\:\boldsymbol{{ln}}\left(\boldsymbol{{x}}\right)}{\left(\boldsymbol{{x}}βˆ’\boldsymbol{{i}}\right)\left(\boldsymbol{{x}}+\boldsymbol{{i}}\right)}\:=\:\frac{\sqrt{βˆ’\boldsymbol{{i}}}\:\boldsymbol{{ln}}\left(βˆ’\boldsymbol{{i}}\right)}{βˆ’\mathrm{2}\boldsymbol{{i}}} \\ $$$$ \\ $$$$\boldsymbol{{Since}}:\:\sqrt{βˆ’\boldsymbol{{i}}}\:=\:\left(βˆ’\boldsymbol{{i}}\right)^{\frac{\mathrm{1}}{\mathrm{2}}} \:=\:\left(\boldsymbol{{e}}^{βˆ’\boldsymbol{{i}}\frac{\boldsymbol{\pi}}{\mathrm{2}}} \right)^{\frac{\mathrm{1}}{\mathrm{2}}} =\:\boldsymbol{{e}}^{βˆ’\boldsymbol{{i}}\frac{\boldsymbol{\pi}}{\mathrm{4}}} \:=\:\boldsymbol{{cos}}\left(\frac{\boldsymbol{\pi}}{\mathrm{4}}\right)\:βˆ’\:\boldsymbol{{i}}\:\boldsymbol{{sin}}\left(\frac{\boldsymbol{\pi}}{\mathrm{4}}\right)\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:βˆ’\:\boldsymbol{{i}}\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\: \\ $$$$ \\ $$$$\boldsymbol{{ln}}\left(βˆ’\boldsymbol{{i}}\right)\:=\:\boldsymbol{{ln}}\:\left(\:\boldsymbol{{e}}^{βˆ’\boldsymbol{{i}}\frac{\boldsymbol{\pi}}{\mathrm{2}}} \right)\:=\:βˆ’\:\boldsymbol{{i}}\:\frac{\boldsymbol{\pi}}{\mathrm{2}} \\ $$$$ \\ $$$$\therefore\:\sqrt{βˆ’\boldsymbol{{i}}}\:\boldsymbol{{ln}}\left(βˆ’\boldsymbol{{i}}\right)\:=\:βˆ’\:\boldsymbol{{i}}\:\frac{\boldsymbol{\pi}}{\mathrm{2}\:\sqrt{\:\mathrm{2}}}\:βˆ’\:\frac{\boldsymbol{\pi}}{\mathrm{2}\:\sqrt{\:\mathrm{2}}}\: \\ $$$$ \\ $$$$\therefore\:\boldsymbol{\Omega}\:=\:\boldsymbol{{Re}}\:\left(\:\mathrm{2}\boldsymbol{\pi{i}}\:Γ—\boldsymbol{{Res}}\left(\:\boldsymbol{{f}},βˆ’\boldsymbol{{i}}\right)\:\right)=\:\frac{βˆ’\frac{\mathrm{2}\boldsymbol{\pi}^{\mathrm{2}} }{\mathrm{2}\sqrt{\:\mathrm{2}}}\:βˆ’\:\boldsymbol{{i}}\:\frac{\mathrm{2}\boldsymbol{\pi}^{\mathrm{2}} }{\mathrm{2}\:\sqrt{\mathrm{2}}}}{βˆ’\:\mathrm{2}\:\boldsymbol{{i}}}\:=\:\:\frac{\boldsymbol{\pi}^{\mathrm{2}} }{\:\mathrm{2}\:\sqrt{\:\mathrm{2}}}\:\: \\ $$$$ \\ $$$$\therefore\:\therefore\:\boldsymbol{\Omega}\:=\:\frac{\boldsymbol{\pi}^{\mathrm{2}} }{\mathrm{2}\:\sqrt{\:\mathrm{2}}}\: \\ $$$$ \\ $$$$\:\langle\:\boldsymbol{{M}}\:.\:\boldsymbol{{T}}\:\:\rangle \\ $$
Commented by mindispower last updated on 01/Oct/21
Hello sir i hop all going well Nice solution By using Residu Theorem have a nice day
$${Hello}\:{sir}\:{i}\:{hop}\:{all}\:{going}\:{well} \\ $$$${Nice}\:{solution}\:{By}\:{using}\:{Residu}\:{Theorem} \\ $$$${have}\:{a}\:{nice}\:{day} \\ $$
Commented by aliyn last updated on 01/Oct/21
thank you sir !
$$\boldsymbol{{thank}}\:\boldsymbol{{you}}\:\boldsymbol{{sir}}\:! \\ $$
Commented by tabata last updated on 01/Oct/21
thank you sir
$$\boldsymbol{{thank}}\:\boldsymbol{{you}}\:\boldsymbol{{sir}} \\ $$
Answered by mindispower last updated on 01/Oct/21
Ξ©=(βˆ‚/βˆ‚a)∫_0 ^∞ (x^a /(1+x^2 ))dx∣_(a=(1/2)) ∫_0 ^∞ (x^a /(1+x^2 ))dx=∫_0 ^∞ (u^(a/2) /(1+u)).(du/(2(√u))) =(1/2)∫_0 ^∞ (u^(((a+1)/2)βˆ’1) /(1+u))du=f(a) recallΞ²(x,y)=∫_0 ^∞ (t^(xβˆ’1) /((1+t)^(x+y) ))dx f(a)=((Ξ²(((a+1)/2),((1βˆ’a)/2)))/2)=(1/2).(Ξ²(((1+a)/2),1βˆ’((1+a)/2)) =(1/2).(Ο€/(sin(((1+a)/2)Ο€)))=(Ο€/(2cos(((Ο€a)/2)))) Ξ©=(Ο€^2 /4).((sin(((Ο€a)/2)))/(cos^2 (((Ο€a)/2))))∣a=(1/2) =(Ο€^2 /4).(√2)=(Ο€^2 /(2(√2)))
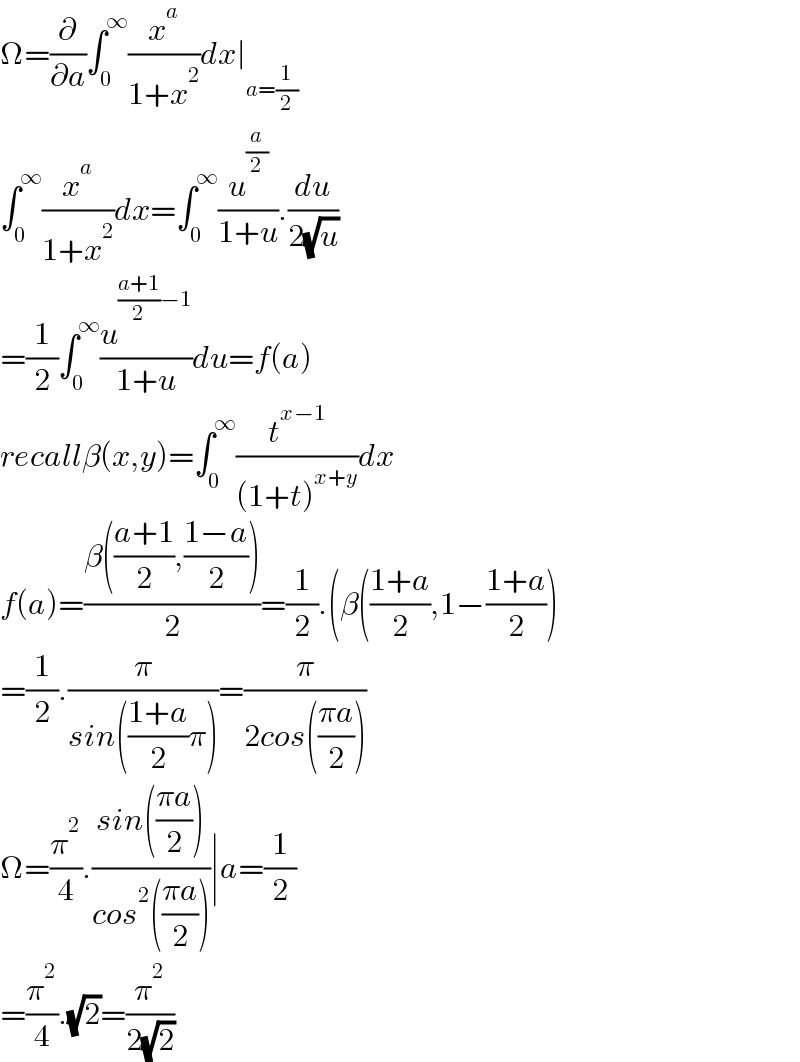
$$\Omega=\frac{\partial}{\partial{a}}\int_{\mathrm{0}} ^{\infty} \frac{{x}^{{a}} }{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\mid_{{a}=\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{{x}^{{a}} }{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\int_{\mathrm{0}} ^{\infty} \frac{{u}^{\frac{{a}}{\mathrm{2}}} }{\mathrm{1}+{u}}.\frac{{du}}{\mathrm{2}\sqrt{{u}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \frac{{u}^{\frac{{a}+\mathrm{1}}{\mathrm{2}}βˆ’\mathrm{1}} }{\mathrm{1}+{u}}{du}={f}\left({a}\right) \\ $$$${recall}\beta\left({x},{y}\right)=\int_{\mathrm{0}} ^{\infty} \frac{{t}^{{x}βˆ’\mathrm{1}} }{\left(\mathrm{1}+{t}\right)^{{x}+{y}} }{dx} \\ $$$${f}\left({a}\right)=\frac{\beta\left(\frac{{a}+\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}βˆ’{a}}{\mathrm{2}}\right)}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}}.\left(\beta\left(\frac{\mathrm{1}+{a}}{\mathrm{2}},\mathrm{1}βˆ’\frac{\mathrm{1}+{a}}{\mathrm{2}}\right)\right. \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}.\frac{\pi}{{sin}\left(\frac{\mathrm{1}+{a}}{\mathrm{2}}\pi\right)}=\frac{\pi}{\mathrm{2}{cos}\left(\frac{\pi{a}}{\mathrm{2}}\right)} \\ $$$$\Omega=\frac{\pi^{\mathrm{2}} }{\mathrm{4}}.\frac{{sin}\left(\frac{\pi{a}}{\mathrm{2}}\right)}{{cos}^{\mathrm{2}} \left(\frac{\pi{a}}{\mathrm{2}}\right)}\mid{a}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$=\frac{\pi^{\mathrm{2}} }{\mathrm{4}}.\sqrt{\mathrm{2}}=\frac{\pi^{\mathrm{2}} }{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$
Commented by mathdanisur last updated on 01/Oct/21
Very nice solution, thank you Ser
$$\mathrm{Very}\:\mathrm{nice}\:\mathrm{solution},\:\mathrm{thank}\:\mathrm{you}\:\boldsymbol{\mathrm{S}}\mathrm{er} \\ $$
Commented by mindispower last updated on 04/Oct/21
thanks Sir Withe plesur
$${thanks}\:{Sir}\:{Withe}\:{plesur} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *