Question Number 45213 by MrW3 last updated on 10/Oct/18
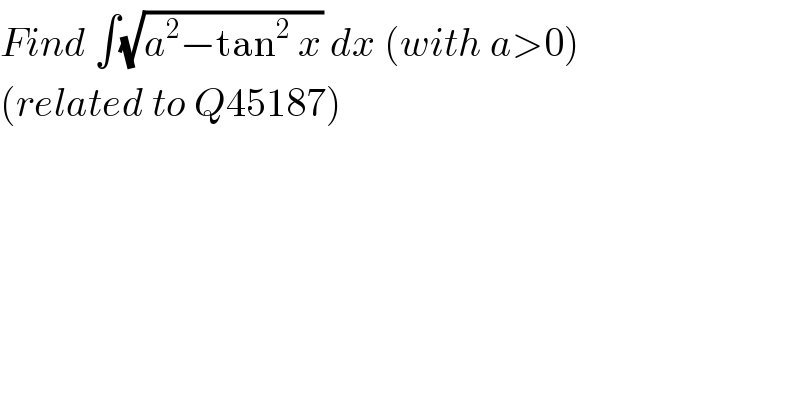
Answered by MrW3 last updated on 10/Oct/18
![I=∫(√(a^2 −tan^2 x)) dx let u=tan x du=(dx/(cos^2 x))=(1+tan^2 x)dx=(1+u^2 )dx I=∫((√(a^2 −u^2 ))/(1+u^2 ))du let u=a sin θ ⇒θ=sin^(−1) (u/a) du=a cos θ dθ I=a^2 ∫((cos^2 θ)/(1+a^2 sin^2 θ))dθ let v=tan θ dv=(dθ/(cos^2 θ))=(1+tan^2 θ)dθ=(v^2 +1)dθ I=a^2 ∫(dv/({(a^2 +1)v^2 +1}(v^2 +1))) (1/({(a^2 +1)v^2 +1}(v^2 +1)))=(A/((a^2 +1)v^2 +1))+(B/(v^2 +1)) A(v^2 +1)+B(a^2 +1)v^2 +B=1 {A+B(a^2 +1)}v^2 +(A+B)=1 ⇒A+B=1 ⇒A+B(a^2 +1)=0 ⇒B=−(1/a^2 ) ⇒A=((a^2 +1)/a^2 ) I=a^2 ∫[((a^2 +1)/(a^2 {(a^2 +1)v^2 +1}))−(1/(a^2 (v^2 +1)))]dv I=∫[((a^2 +1)/((a^2 +1)v^2 +1))−(1/(v^2 +1))]dv I=(a^2 +1)∫(1/((a^2 +1)v^2 +1))dv−∫(1/(v^2 +1))dv I=(a^2 +1)I_1 −I_2 I_1 =∫(1/((a^2 +1)v^2 +1))dv let p=(√(a^2 +1))v dp=(√(a^2 +1)) dv I_1 =(1/( (√(a^2 +1))))∫(dp/(p^2 +1))=(1/( (√(a^2 +1))))tan^(−1) p I_1 =(1/( (√(a^2 +1)))) tan^(−1) ((√(a^2 +1))v) I_1 =(1/( (√(a^2 +1)))) tan^(−1) ((√(a^2 +1)) tan θ) I_1 =(1/( (√(a^2 +1)))) tan^(−1) ((√(a^2 +1)) ((sin θ)/( (√(1−sin^2 θ))))) I_1 =(1/( (√(a^2 +1)))) tan^(−1) ((√(a^2 +1)) ((u/a)/( (√(1−(u^2 /a^2 )))))) I_1 =(1/( (√(a^2 +1)))) tan^(−1) ((√(a^2 +1)) (u/( (√(a^2 −u^2 ))))) ⇒I_1 =(1/( (√(a^2 +1)))) tan^(−1) ((((√(a^2 +1)) tan x)/( (√(a^2 −tan^2 x))))) I_2 =∫(1/(v^2 +1))dv=tan^(−1) v=θ=sin^(−1) (u/a) ⇒I_2 =sin^(−1) (u/a)=sin^(−1) (((tan x)/a)) I=(a^2 +1)I_1 −I_2 ⇒I=((√(a^2 +1))) tan^(−1) ((((√(a^2 +1)) tan x)/( (√(a^2 −tan^2 x)))))−sin^(−1) (((tan x)/a))+C](https://www.tinkutara.com/question/Q45217.png)
Commented by malwaan last updated on 10/Oct/18

Commented by ajfour last updated on 10/Oct/18

Answered by ajfour last updated on 10/Oct/18
![let tan x = asin ψ ⇒ dx= ((acos ψdψ)/(1+a^2 sin^2 ψ)) I=∫((a^2 cos^2 ψ)/(1+a^2 sin^2 ψ))dψ let tan ψ = t ⇒ dψ=(dt/(1+t^2 )) I=∫((a^2 ((1/(1+t^2 )))(dt/((1+t^2 ))))/(1+(a^2 /((1+(1/t^2 )))))) = ∫((a^2 dt)/((1+t^2 )^2 +a^2 t^2 (1+t^2 ))) ............................................... = ∫((a^2 dt)/((a^2 +1)t^4 +(a^2 +2)t^2 +1)) = (a^2 /( (√(a^2 +1))))∫(((1/t^2 ))/( (√(a^2 +1))t^2 +(1/( (√(a^2 +1))t^2 ))+((a^2 +2)/( (√(a^2 +1))))))dt let t(a^2 +1)^(1/4) =bt=z ⇒ bdt = dz ⇒ I = ((b^4 −1)/b^2 )∫(((b^2 /z^2 )((dz/b)))/((z+(1/z))^2 +((b^4 +1)/b^2 )−2)) = ((b^4 −1)/(2b))∫(((1+(1/z^2 ))−(1−(1/z^2 )))/((z+(1/z))^2 +(((b^2 −1)/b))^2 )) dz = ((b^4 −1)/(2b))∫(((1+(1/z^2 ))dz)/((z−(1/z))^2 +(((b^2 +1)/b))^2 )) −((b^4 −1)/(2b))∫(((1−(1/z^2 ))dz)/((z+(1/z))^2 +(((b^2 −1)/b))^2 )) I = ((b^4 −1)/(2b))[(b/(b^2 +1))tan^(−1) (((z−(1/z))/((b^2 +1)/b)))−(b/(b^2 −1))tan^(−1) (((z+(1/z))/((b^2 −1)/b)))]+c And since bt=z , t=tan 𝛙 asin 𝛙 = tan x ⇒ tan 𝛙 = z/b ((az)/( (√(z^2 +b^2 )))) = tan x ∴ z^2 = ((b^2 tan^2 x)/(a^2 −tan^2 x)) ⇒ z = (((a^2 +1)^(1/4) tan x)/( (√(a^2 −tan^2 x)))) ; b=(a^2 +1)^(1/4) I = ((b^4 −1)/(2b))[(b/(b^2 +1))tan^(−1) (((z−(1/z))/((b^2 +1)/b)))−(b/(b^2 −1))tan^(−1) (((z+(1/z))/((b^2 −1)/b)))]+c . I= ((b^2 −1)/2)tan^(−1) ((b(z^2 −1))/(z(b^2 +1))) −((b^2 +1)/2)tan^(−1) ((b(z^2 +1))/(z(b^2 −1))) .](https://www.tinkutara.com/question/Q45219.png)
Commented by MrW3 last updated on 10/Oct/18

Answered by tanmay.chaudhury50@gmail.com last updated on 11/Oct/18
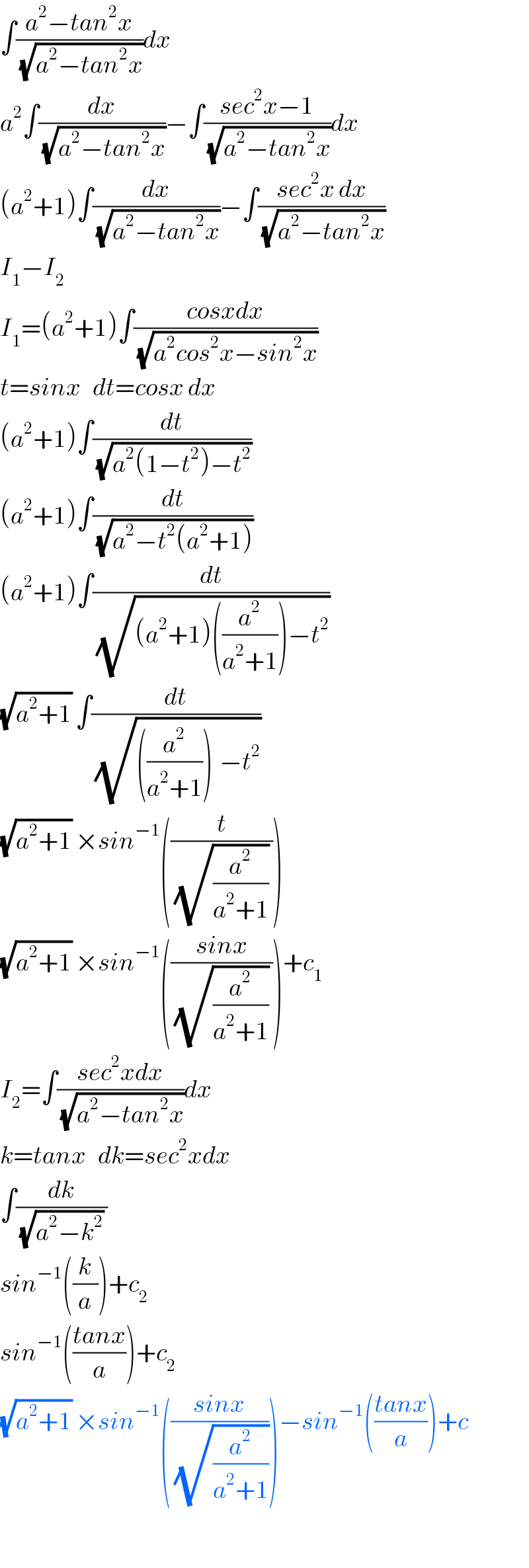
Commented by tanmay.chaudhury50@gmail.com last updated on 11/Oct/18

Commented by tanmay.chaudhury50@gmail.com last updated on 11/Oct/18

Commented by MrW3 last updated on 11/Oct/18

Commented by tanmay.chaudhury50@gmail.com last updated on 11/Oct/18
Commented by MrW3 last updated on 11/Oct/18
Commented by MrW3 last updated on 11/Oct/18

