Question Number 79095 by mathmax by abdo last updated on 22/Jan/20
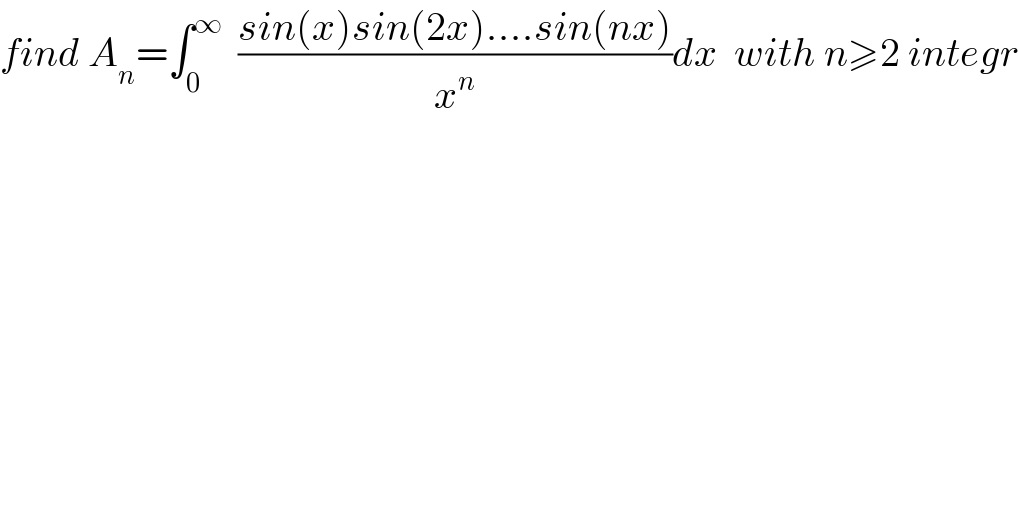
$${find}\:{A}_{{n}} =\int_{\mathrm{0}} ^{\infty} \:\:\frac{{sin}\left({x}\right){sin}\left(\mathrm{2}{x}\right)….{sin}\left({nx}\right)}{{x}^{{n}} }{dx}\:\:{with}\:{n}\geqslant\mathrm{2}\:{integr} \\ $$
Commented by mind is power last updated on 23/Jan/20
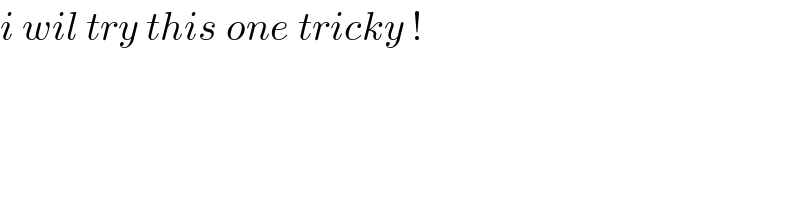
$${i}\:{wil}\:{try}\:{this}\:{one}\:{tricky}\:! \\ $$
