Question Number 97554 by 1549442205 last updated on 08/Jun/20

Commented by Rasheed.Sindhi last updated on 09/Jun/20
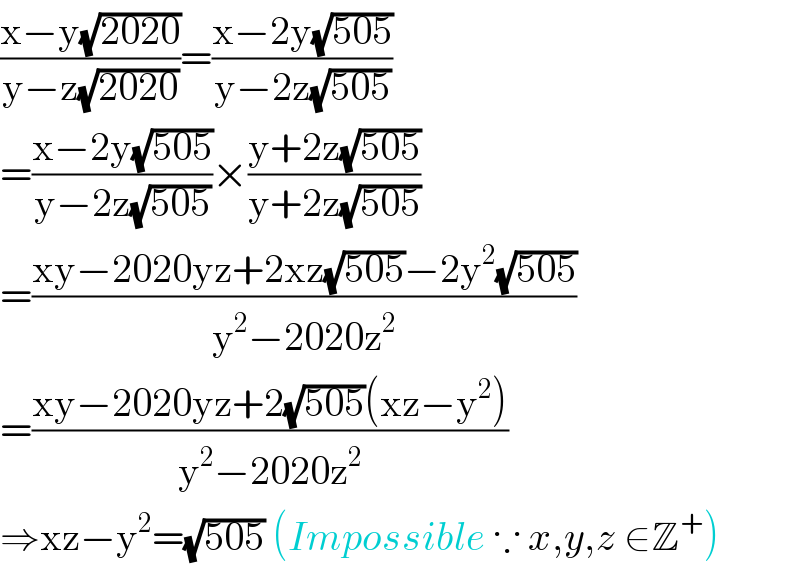
Commented by prakash jain last updated on 08/Jun/20

Commented by Rasheed.Sindhi last updated on 08/Jun/20

Commented by Rasheed.Sindhi last updated on 09/Jun/20

Commented by prakash jain last updated on 09/Jun/20
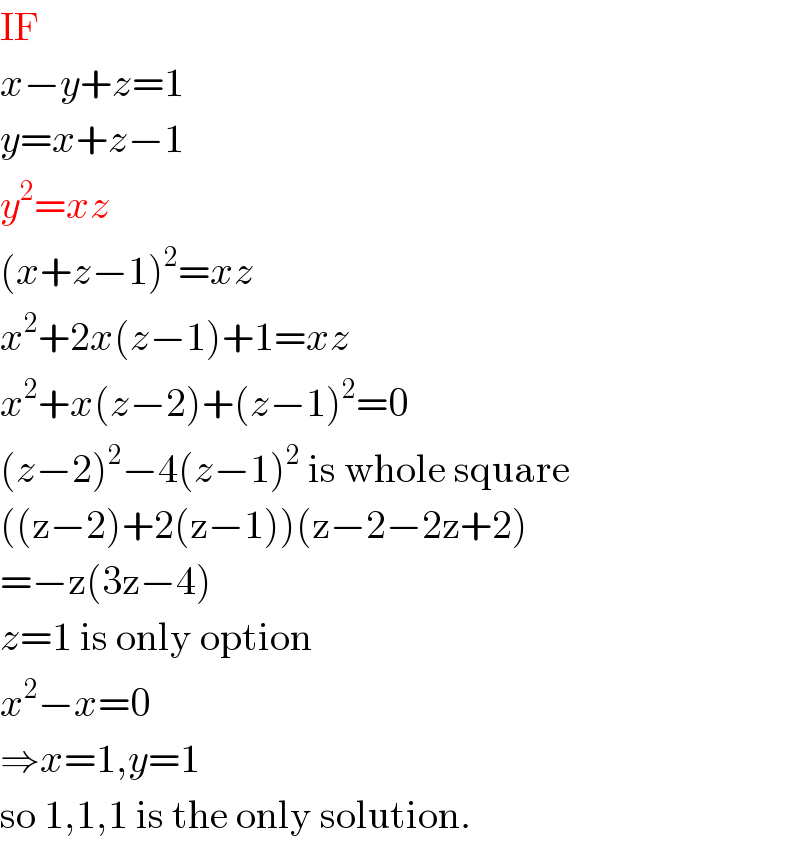
Answered by 1549442205 last updated on 09/Jun/20
![Let ((x−y(√(2020)))/(y−z(√(2020))))=(m/n),where m,n∈Z,gcd(m;n)=1 ⇔nx−2ny(√(505))=my−2mz(√(505)) ⇔(2mz−2ny)(√(505)) =my−nx if 2mz−2ny≠0 then (√(505))=((my−nx)/(2mz−2ny))∈Q(set of rational numbers) This is a contradiction because (√(505)) be irrational number Hence,we need must have { ((2mz−2ny=0)),((my−nx=0)) :} it follows that (m/n)=(x/y)=(y/z) ⇒y^2 =xz.Now we denote gcd(x,z)=d⇒ { ((x=ad)),((z=bd)) :} (1) with a,b∈N and gcd(a,b)=1 ⇒y^2 =abd^2 ⇒a=u^2 ,b=v^2 (u,v∈N^∗ )⇒y=uvd.Replace into (1) we get x=u^2 d,z=v^2 d. From this we get x^2 +y^2 +z^2 =d^2 (u^4 +u^2 v^2 +v^4 ) =d^2 [(u^2 +v^2 )^2 −u^2 v^2 ]=d^2 (u^2 −uv+v^2 )(u^2 +uv+v^2 ) From the condition x^2 +y^2 +z^2 is a prime we infer that { ((d^2 =1)),((u^2 −uv+v^2 =1)) :}.Therefore, (u−v)^2 =1−uv≥0 ⇔ ^(u,v∈N^∗ ) u=v=1⇔x=y=z=1 Thus,(x;y;z)=(1;1;1) is unique triple satisfying requirement of the problem](https://www.tinkutara.com/question/Q97639.png)
