Menu Close

find-dx-3-tan-2-x-

Tinku Tara 0 Comments
Pin




Question Number 42430 by maxmathsup by imad last updated on 25/Aug/18
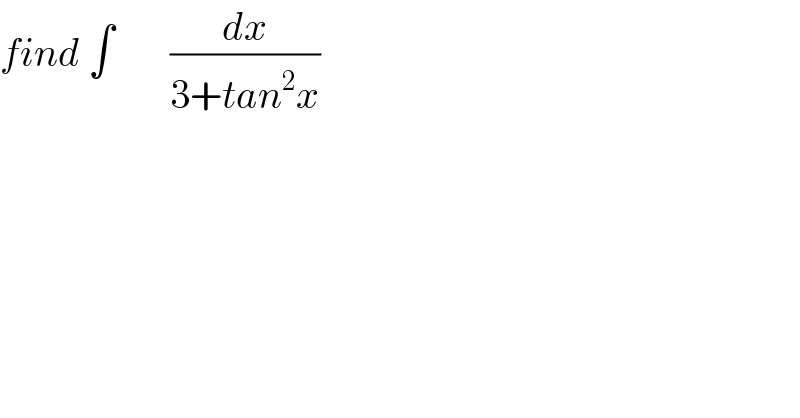
find ∫ (dx/(3+tan^2 x))
$${find}\:\int\:\:\:\:\:\:\:\frac{{dx}}{\mathrm{3}+{tan}^{\mathrm{2}} {x}} \\ $$
Commented by maxmathsup by imad last updated on 25/Aug/18
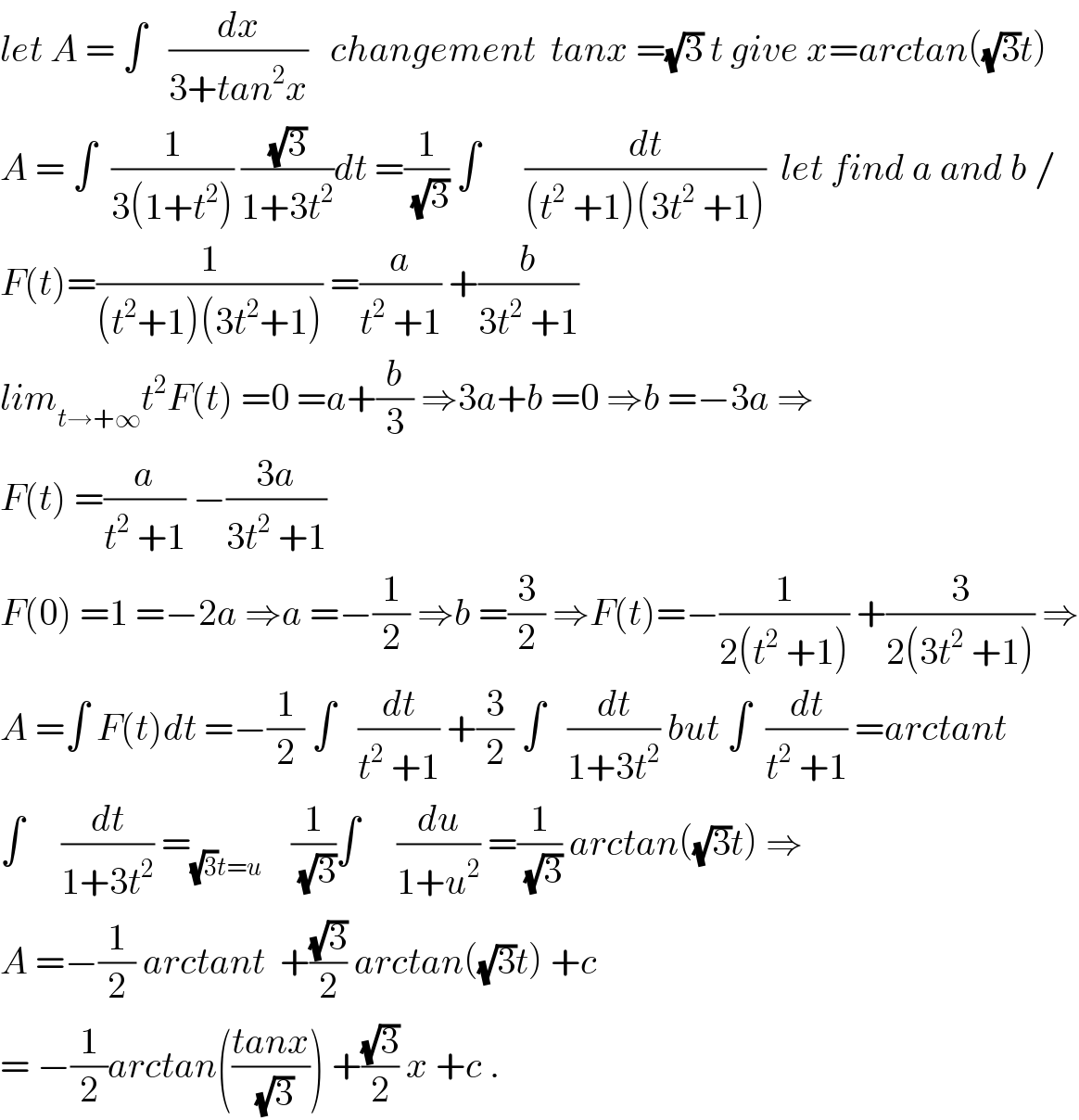
let A = ∫ (dx/(3+tan^2 x)) changement tanx =(√3) t give x=arctan((√3)t) A = ∫ (1/(3(1+t^2 ))) ((√3)/(1+3t^2 ))dt =(1/( (√3))) ∫ (dt/((t^2 +1)(3t^2 +1))) let find a and b / F(t)=(1/((t^2 +1)(3t^2 +1))) =(a/(t^2 +1)) +(b/(3t^2 +1)) lim_(t→+∞) t^2 F(t) =0 =a+(b/3) ⇒3a+b =0 ⇒b =−3a ⇒ F(t) =(a/(t^2 +1)) −((3a)/(3t^2 +1)) F(0) =1 =−2a ⇒a =−(1/2) ⇒b =(3/2) ⇒F(t)=−(1/(2(t^2 +1))) +(3/(2(3t^2 +1))) ⇒ A =∫ F(t)dt =−(1/2) ∫ (dt/(t^2 +1)) +(3/2) ∫ (dt/(1+3t^2 )) but ∫ (dt/(t^2 +1)) =arctant ∫ (dt/(1+3t^2 )) =_((√3)t=u) (1/( (√3)))∫ (du/(1+u^2 )) =(1/( (√3))) arctan((√3)t) ⇒ A =−(1/2) arctant +((√3)/2) arctan((√3)t) +c = −(1/2)arctan(((tanx)/( (√3)))) +((√3)/2) x +c .
$${let}\:{A}\:=\:\int\:\:\:\frac{{dx}}{\mathrm{3}+{tan}^{\mathrm{2}} {x}}\:\:\:{changement}\:\:{tanx}\:=\sqrt{\mathrm{3}}\:{t}\:{give}\:{x}={arctan}\left(\sqrt{\mathrm{3}}{t}\right) \\ $$$${A}\:=\:\int\:\:\frac{\mathrm{1}}{\mathrm{3}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:\frac{\sqrt{\mathrm{3}}}{\mathrm{1}+\mathrm{3}{t}^{\mathrm{2}} }{dt}\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\:\int\:\:\:\:\:\:\frac{{dt}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)\left(\mathrm{3}{t}^{\mathrm{2}} \:+\mathrm{1}\right)}\:\:{let}\:{find}\:{a}\:{and}\:{b}\:/ \\ $$$${F}\left({t}\right)=\frac{\mathrm{1}}{\left({t}^{\mathrm{2}} +\mathrm{1}\right)\left(\mathrm{3}{t}^{\mathrm{2}} +\mathrm{1}\right)}\:=\frac{{a}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{{b}}{\mathrm{3}{t}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${lim}_{{t}\rightarrow+\infty} {t}^{\mathrm{2}} {F}\left({t}\right)\:=\mathrm{0}\:={a}+\frac{{b}}{\mathrm{3}}\:\Rightarrow\mathrm{3}{a}+{b}\:=\mathrm{0}\:\Rightarrow{b}\:=−\mathrm{3}{a}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{{a}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:−\frac{\mathrm{3}{a}}{\mathrm{3}{t}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)\:=\mathrm{1}\:=−\mathrm{2}{a}\:\Rightarrow{a}\:=−\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow{b}\:=\frac{\mathrm{3}}{\mathrm{2}}\:\Rightarrow{F}\left({t}\right)=−\frac{\mathrm{1}}{\mathrm{2}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}\:+\frac{\mathrm{3}}{\mathrm{2}\left(\mathrm{3}{t}^{\mathrm{2}} \:+\mathrm{1}\right)}\:\Rightarrow \\ $$$${A}\:=\int\:{F}\left({t}\right){dt}\:=−\frac{\mathrm{1}}{\mathrm{2}}\:\int\:\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{\mathrm{3}}{\mathrm{2}}\:\int\:\:\:\frac{{dt}}{\mathrm{1}+\mathrm{3}{t}^{\mathrm{2}} }\:{but}\:\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:={arctant} \\ $$$$\int\:\:\:\:\:\frac{{dt}}{\mathrm{1}+\mathrm{3}{t}^{\mathrm{2}} }\:=_{\sqrt{\mathrm{3}}{t}={u}} \:\:\:\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\int\:\:\:\:\:\frac{{du}}{\mathrm{1}+{u}^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\:{arctan}\left(\sqrt{\mathrm{3}}{t}\right)\:\Rightarrow \\ $$$${A}\:=−\frac{\mathrm{1}}{\mathrm{2}}\:{arctant}\:\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{arctan}\left(\sqrt{\mathrm{3}}{t}\right)\:+{c} \\ $$$$=\:−\frac{\mathrm{1}}{\mathrm{2}}{arctan}\left(\frac{{tanx}}{\:\sqrt{\mathrm{3}}}\right)\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{x}\:+{c}\:. \\ $$
Commented by maxmathsup by imad last updated on 25/Aug/18
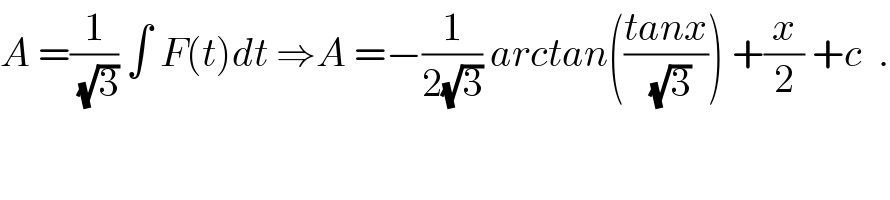
A =(1/( (√3))) ∫ F(t)dt ⇒A =−(1/(2(√3))) arctan(((tanx)/( (√3)))) +(x/2) +c .
$${A}\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\:\int\:{F}\left({t}\right){dt}\:\Rightarrow{A}\:=−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:{arctan}\left(\frac{{tanx}}{\:\sqrt{\mathrm{3}}}\right)\:+\frac{{x}}{\mathrm{2}}\:+{c}\:\:. \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 25/Aug/18
∫(dx/(3+((1−cos2x)/(1+cos2x)))) ∫((1+cos2x)/(3+3cos2x+1−cos2x))dx ∫((1+cos2x)/(4+2cos2x))dx (1/2)∫((2+cos2x−1)/(2+cos2x))dx (1/2)∫dx−(1/2)∫(dx/(2+cos2x)) (((1/2)∫dx−(1/2)∫((1+tan^2 x)/(2+2tan^2 x+1−tan^2 x))dx)/) (1/2)∫dx−(1/2)∫((sec^2 x)/(3+tan^2 x))dx (1/2)∫dx−(1/2)∫((d(tanx))/(3+tan^2 x))dx→∫(dx/(x^2 +a^2 )) formula (x/2)−(1/2)×(1/( (√3)))tan^(−1) (((tanx)/( (√3))))+c
$$\int\frac{{dx}}{\mathrm{3}+\frac{\mathrm{1}−{cos}\mathrm{2}{x}}{\mathrm{1}+{cos}\mathrm{2}{x}}} \\ $$$$\int\frac{\mathrm{1}+{cos}\mathrm{2}{x}}{\mathrm{3}+\mathrm{3}{cos}\mathrm{2}{x}+\mathrm{1}−{cos}\mathrm{2}{x}}{dx} \\ $$$$\int\frac{\mathrm{1}+{cos}\mathrm{2}{x}}{\mathrm{4}+\mathrm{2}{cos}\mathrm{2}{x}}{dx} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{2}+{cos}\mathrm{2}{x}−\mathrm{1}}{\mathrm{2}+{cos}\mathrm{2}{x}}{dx} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int{dx}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dx}}{\mathrm{2}+{cos}\mathrm{2}{x}} \\ $$$$\frac{\frac{\mathrm{1}}{\mathrm{2}}\int{dx}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}+{tan}^{\mathrm{2}} {x}}{\mathrm{2}+\mathrm{2}{tan}^{\mathrm{2}} {x}+\mathrm{1}−{tan}^{\mathrm{2}} {x}}{dx}}{} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int{dx}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{sec}^{\mathrm{2}} {x}}{\mathrm{3}+{tan}^{\mathrm{2}} {x}}{dx} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int{dx}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{d}\left({tanx}\right)}{\mathrm{3}+{tan}^{\mathrm{2}} {x}}{dx}\rightarrow\int\frac{{dx}}{{x}^{\mathrm{2}} +{a}^{\mathrm{2}} }\:\:\:{formula} \\ $$$$\frac{{x}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}{tan}^{−\mathrm{1}} \left(\frac{{tanx}}{\:\sqrt{\mathrm{3}}}\right)+{c} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *