find-f-0-1-ln-1-e-x-dx-with-0-

Question Number 51825 by Abdo msup. last updated on 30/Dec/18

f i n d f (α) = \int_{0}^{1} l n (1 + e^{- α} x) d x w i t h α ⩾ 0

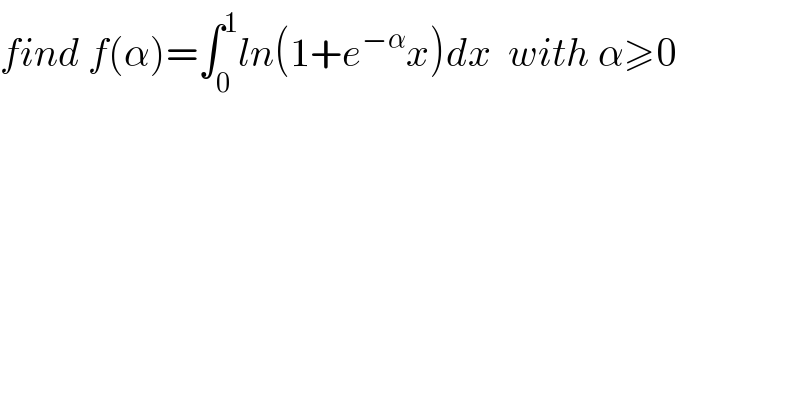
Commented by maxmathsup by imad last updated on 31/Dec/18

l e t p u t e^{- α} = λ \Rightarrow f (α) = \int_{0}^{1} l n (1 + λ x) d x a n d b y p a r t s u^{^{'}} = 1, v = l n (1 + λ x)

w e g e t f (α) = {[x l n (1 + λ x)]}_{0}^{1} - \int_{0}^{1} x \frac{λ}{1 + λ x} d x

= l n (1 + λ) - \int_{0}^{1} \frac{λ x + 1 - 1}{1 + λ x} d x = l n (1 + λ) - 1 + \int_{0}^{1} \frac{d x}{1 + λ x}

= l n (1 + λ) - 1 + \frac{1}{λ} l n ∣ 1 + λ x ∣ + c \Rightarrow

f (α) = l n (1 + e^{- α}) - 1 + e^{- α} l n ∣ 1 + e^{- α} x ∣ + c .

Commented by maxmathsup by imad last updated on 31/Dec/18

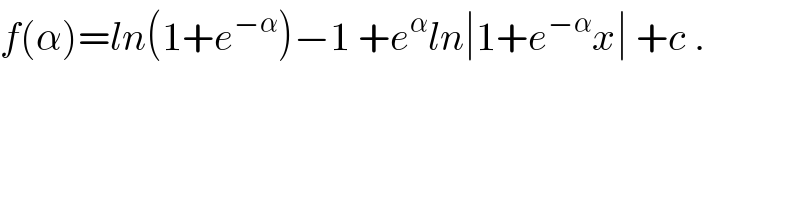
f (α) = l n (1 + e^{- α}) - 1 + e^{α} l n ∣ 1 + e^{- α} x ∣ + c .

Commented by Abdo msup. last updated on 31/Dec/18

f (α) = l n (1 + λ) - 1 + {[\frac{1}{λ} l n ∣ 1 + λ x ∣]}_{0}^{1} + c

= l n (1 + λ) - 1 + \frac{1}{λ} l n (1 + λ) + c

\Rightarrow f (α) = l n (1 + e^{- α}) - 1 + e^{α} l n (1 + e^{- α}) + c

= (1 + e^{α}) l n (1 + e^{- α}) - 1 + c .

Answered by Smail last updated on 30/Dec/18

l e t t = 1 + e^{- α} x \Rightarrow d x = e^{α} d t

f (α) = e^{α} \int_{1}^{1 + e^{- α}} l n (t) d t

b y p a r t s

u = l n (t) \Rightarrow u^{'} = \frac{1}{t}

v^{'} = 1 \Rightarrow v = t

f (α) = e^{α} {[t l n t]}_{1}^{1 + e^{- α}} - e^{α} \int_{1}^{1 + e^{- α}} d t

= e^{α} ((1 + e^{- α}) l n (1 + e^{- α}) - e^{- α})

f (α) = (e^{α} + 1) (l n (e^{α} + 1) - α) - 1

Facebook Tweet Pin