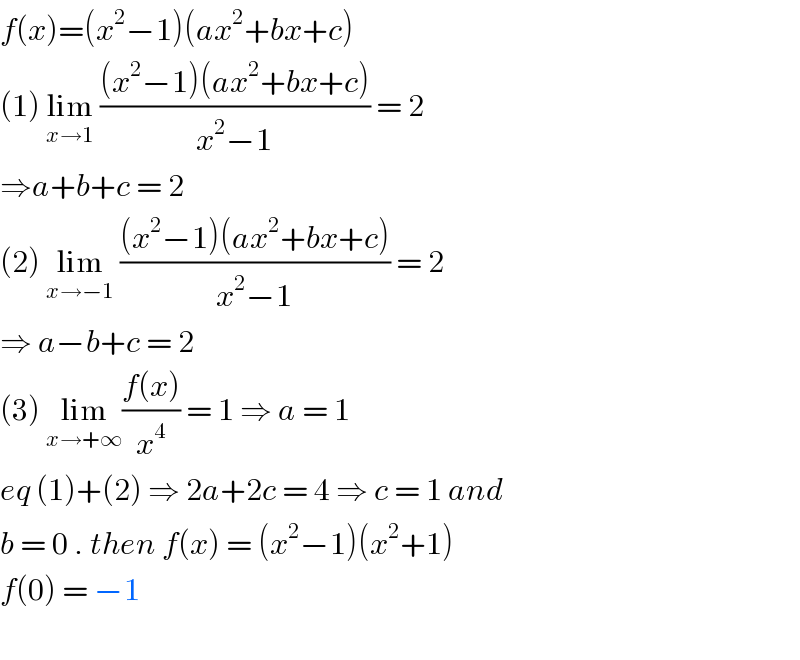Find-f-0-when-a-polynomial-f-x-satisfies-lim-x-1-f-x-x-2-1-2-lim-x-1-f-x-x-2-1-2-lim-x-f-x-x-4-1-pls-Help-

Question Number 94214 by abony1303 last updated on 17/May/20

F i n d f (0) w h e n a p o l y n o m i a l f (x) s a t i s f i e s

\lim_{x \to 1} \frac{f (x)}{x^{2} - 1} = 2

\lim_{x \to - 1} \frac{f (x)}{x^{2} - 1} = 2

\lim_{x \to + \infty} \frac{f (x)}{x^{4}} = 1 p l s H e l p!

Commented by john santu last updated on 17/May/20

\lim_{x \to + \infty} \frac{f (x)}{x^{4}} = ? ?

Commented by john santu last updated on 17/May/20

f (x) = (x^{2} - 1) ({a x}^{2} + b)

Commented by abony1303 last updated on 17/May/20

c h a n g e d

Commented by i jagooll last updated on 17/May/20

f (x) = (x^{2} - 1) ({a x}^{2} + b x + c)

(1) \lim_{x \to 1} \frac{(x^{2} - 1) ({a x}^{2} + b x + c)}{x^{2} - 1} = 2

\Rightarrow a + b + c = 2

(2) \lim_{x \to - 1} \frac{(x^{2} - 1) ({a x}^{2} + b x + c)}{x^{2} - 1} = 2

\Rightarrow a - b + c = 2

(3) \lim_{x \to + \infty} \frac{f (x)}{x^{4}} = 1 \Rightarrow a = 1

e q (1) + (2) \Rightarrow 2 a + 2 c = 4 \Rightarrow c = 1 a n d

b = 0 . t h e n f (x) = (x^{2} - 1) (x^{2} + 1)

f (0) = - 1