Menu Close

Find-n-N-n-100-4-2-n-n-3-Note-that-S-denotes-the-cardinality-or-number-of-elements-of-a-set-S-

Tinku Tara 0 Comments
Pin




Question Number 112011 by Aina Samuel Temidayo last updated on 05/Sep/20
Find ∣{n∈N∣n≤100, 4!∣2^n −n^3 }∣. (Note that ∣S∣ denotes the cardinality or number of elements of a set,S).
$$\mathrm{Find}\:\mid\left\{\mathrm{n}\in\mathbb{N}\mid\mathrm{n}\leqslant\mathrm{100},\:\mathrm{4}!\mid\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} \right\}\mid. \\ $$$$\left(\mathrm{Note}\:\mathrm{that}\:\mid\mathrm{S}\mid\:\mathrm{denotes}\:\mathrm{the}\:\mathrm{cardinality}\right. \\ $$$$\left.\mathrm{or}\:\mathrm{number}\:\mathrm{of}\:\mathrm{elements}\:\mathrm{of}\:\mathrm{a}\:\mathrm{set},\mathrm{S}\right). \\ $$
Answered by Rasheed.Sindhi last updated on 07/Sep/20
n may be odd or even. ^▶ n=2k+1 2^n −n^3 =2^(2k+1) −(2k+1)^3 =2.4^k −(8k^3 +1+3(2k)(2k+1)) =2.4^k −8k^3 −1−12k^2 −6k =2.4^k −8k^3 −12k^2 −6k−1 =an odd number(not divisible by 4) ^▶ n=2k 2^n −n^3 =2^(2k) −(2k)^3 =4^k −8k^3 =4(4^(k−1) −2k^3 ) ^▶ Hence only for n∈E 4 ∣ 2^n −n^3 ^▶ Even numbers upto 100 is 50 This proves that ∣{n∈N∣n≤100, 4!∣2^n −n^3 }∣=50 Recall that I considerd N={1,2,3,...} ( If we consider N={0,1,2,3,...} Then the answer is 51)
$${n}\:{may}\:{be}\:{odd}\:{or}\:{even}. \\ $$$$\:^{\blacktriangleright} {n}=\mathrm{2}{k}+\mathrm{1} \\ $$$$\:\:\:\:\:\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} =\mathrm{2}^{\mathrm{2}{k}+\mathrm{1}} −\left(\mathrm{2}{k}+\mathrm{1}\right)^{\mathrm{3}} \\ $$$$\:\:\:\:\:=\mathrm{2}.\mathrm{4}^{{k}} −\left(\mathrm{8}{k}^{\mathrm{3}} +\mathrm{1}+\mathrm{3}\left(\mathrm{2}{k}\right)\left(\mathrm{2}{k}+\mathrm{1}\right)\right) \\ $$$$\:\:\:\:\:=\mathrm{2}.\mathrm{4}^{{k}} −\mathrm{8}{k}^{\mathrm{3}} −\mathrm{1}−\mathrm{12}{k}^{\mathrm{2}} −\mathrm{6}{k} \\ $$$$\:\:\:\:\:=\mathrm{2}.\mathrm{4}^{{k}} −\mathrm{8}{k}^{\mathrm{3}} −\mathrm{12}{k}^{\mathrm{2}} −\mathrm{6}{k}−\mathrm{1} \\ $$$$\:\:\:\:={an}\:{odd}\:{number}\left({not}\:{divisible}\right. \\ $$$$\left.\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{by}\:\:\mathrm{4}\right) \\ $$$$\:^{\blacktriangleright} {n}=\mathrm{2}{k} \\ $$$$\:\:\:\:\:\:\:\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} =\mathrm{2}^{\mathrm{2}{k}} −\left(\mathrm{2}{k}\right)^{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{4}^{{k}} −\mathrm{8}{k}^{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{4}\left(\mathrm{4}^{{k}−\mathrm{1}} −\mathrm{2}{k}^{\mathrm{3}} \right) \\ $$$$\:^{\blacktriangleright} \mathcal{H}{ence}\:{only}\:{for}\:{n}\in\mathbb{E} \\ $$$$\:\:\:\:\:\:\mathrm{4}\:\mid\:\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} \\ $$$$\:^{\blacktriangleright} \mathcal{E}{ven}\:{numbers}\:{upto}\:\mathrm{100}\:{is}\:\mathrm{50} \\ $$$${This}\:{proves}\:{that} \\ $$$$\mid\left\{\mathrm{n}\in\mathbb{N}\mid\mathrm{n}\leqslant\mathrm{100},\:\mathrm{4}!\mid\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} \right\}\mid=\mathrm{50} \\ $$$${Recall}\:{that}\:{I}\:{considerd}\: \\ $$$$\:\:\:\mathbb{N}=\left\{\mathrm{1},\mathrm{2},\mathrm{3},…\right\} \\ $$$$\:\left(\:{If}\:{we}\:{consider}\right. \\ $$$$\:\:\:\mathbb{N}=\left\{\mathrm{0},\mathrm{1},\mathrm{2},\mathrm{3},…\right\} \\ $$$$\left.\:\:\:\:{Then}\:{the}\:{answer}\:{is}\:\mathrm{51}\right) \\ $$
Commented by Rasheed.Sindhi last updated on 07/Sep/20
Sorry I can′t do it.However with the help of calculator, the numbers are 4,10,16,....,6n−2 So the number of these numbers upto 100 is 17
$${Sorry}\:{I}\:{can}'{t}\:{do}\:{it}.{However} \\ $$$${with}\:{the}\:{help}\:{of}\:{calculator},\:{the} \\ $$$${numbers}\:{are}\:\mathrm{4},\mathrm{10},\mathrm{16},….,\mathrm{6}{n}−\mathrm{2} \\ $$$${So}\:{the}\:{number}\:{of}\:{these}\:{numbers} \\ $$$${upto}\:\mathrm{100}\:{is}\:\mathrm{17} \\ $$
Commented by kaivan.ahmadi last updated on 05/Sep/20
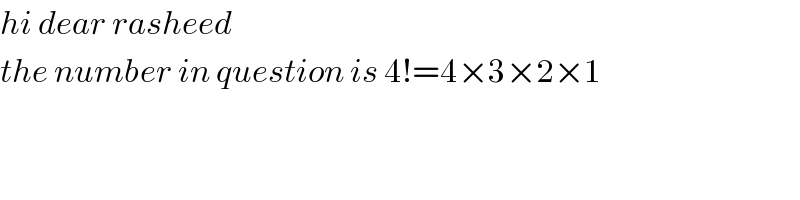
hi dear rasheed the number in question is 4!=4×3×2×1
$${hi}\:{dear}\:{rasheed} \\ $$$${the}\:{number}\:{in}\:{question}\:{is}\:\mathrm{4}!=\mathrm{4}×\mathrm{3}×\mathrm{2}×\mathrm{1} \\ $$
Commented by Rasheed.Sindhi last updated on 05/Sep/20
Misreading sir misreading! I′ll review my answer. ThanX dear kaivan!
$${Misreading}\:{sir}\:{misreading}! \\ $$$${I}'{ll}\:{review}\:{my}\:{answer}. \\ $$$$\mathcal{T}{han}\mathcal{X}\:\:{dear}\:{kaivan}! \\ $$
Commented by Aina Samuel Temidayo last updated on 06/Sep/20
Please I′m still waiting for the correction. Thanks.
$$\mathrm{Please}\:\mathrm{I}'\mathrm{m}\:\mathrm{still}\:\mathrm{waiting}\:\mathrm{for}\:\mathrm{the} \\ $$$$\mathrm{correction}.\:\mathrm{Thanks}. \\ $$
Answered by Rasheed.Sindhi last updated on 09/Sep/20
4! ∣ 2^n −n^3 24∣2^n −n^3 2^n ≡n^3 (mod 24) Let { ((2^n ≡u(mod 24))),((n^3 ≡v(mod 24)) :} [(n,(2^n mod 24_((u)) ),(n^3 mod 24_((v)) ),),(1,( 2),( 1),),(2,( 4),( 8),),(3,( 8),( 3),),(4,( 16),( 16),(u=v)),(5,( 8),( 5),),(6,( 16),( 0),),(7,( 8),( 7),),(8,( 16),( 8),),(9,( 8),( 9),),((10),( 16),( 16),(u=v)),((11),( 8),( 11),),((12),( 16),( 0),),((13),( 8),( 13),),((14),( 16),( 8),),((15),( 8),( 15),),((16),( 16),( 16),(u=v)),((17),( 8),( 17),),((18),( 16),( 0),),((19),( 8),( 19),),((20),( 16),( 8),),((21),( 8),( 21),),((22),( 16),( 16),(u=v)),((23),( 8),( 23),),((24),( 16),( 0),) ] Values of n for which u=v or 2^n ≡n^3 (mod 24) or in other words 4! ∣ 2^n −n^3 are: 4,10,16,...(6n−2).. This is an AP upto 100 of which are 17 terms. ∴ 4! ∣ 2^n −n^3 has 17 solutions for n∈N ∧ n≤100.
$$\mathrm{4}!\:\mid\:\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} \\ $$$$\mathrm{24}\mid\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} \\ $$$$\mathrm{2}^{{n}} \equiv{n}^{\mathrm{3}} \left({mod}\:\mathrm{24}\right) \\ $$$$ \\ $$$${Let}\:\begin{cases}{\mathrm{2}^{{n}} \equiv{u}\left({mod}\:\mathrm{24}\right)}\\{{n}^{\mathrm{3}} \equiv{v}\left({mod}\:\mathrm{24}\right.}\end{cases} \\ $$$$\:\:\:\:\: \\ $$$$ \\ $$$$\:\:\:\:\begin{bmatrix}{{n}}&{\underset{\left({u}\right)} {\mathrm{2}^{{n}} {mod}\:\mathrm{24}}}&{\underset{\left({v}\right)} {{n}^{\mathrm{3}} {mod}\:\mathrm{24}}}&{}\\{\mathrm{1}}&{\:\:\:\:\:\:\:\:\:\mathrm{2}}&{\:\:\:\:\:\:\:\mathrm{1}}&{}\\{\mathrm{2}}&{\:\:\:\:\:\:\:\:\mathrm{4}}&{\:\:\:\:\:\:\:\mathrm{8}}&{}\\{\mathrm{3}}&{\:\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\:\:\:\:\:\mathrm{3}}&{}\\{\mathrm{4}}&{\:\:\:\:\:\:\mathrm{16}}&{\:\:\:\:\:\mathrm{16}}&{{u}={v}}\\{\mathrm{5}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\:\:\:\:\mathrm{5}}&{}\\{\mathrm{6}}&{\:\:\:\:\:\mathrm{16}}&{\:\:\:\:\:\:\mathrm{0}}&{}\\{\mathrm{7}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\:\:\:\mathrm{7}}&{}\\{\mathrm{8}}&{\:\:\:\:\:\mathrm{16}}&{\:\:\:\:\:\mathrm{8}}&{}\\{\mathrm{9}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\:\:\:\mathrm{9}}&{}\\{\mathrm{10}}&{\:\:\:\:\:\mathrm{16}}&{\:\:\:\mathrm{16}}&{{u}={v}}\\{\mathrm{11}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\:\mathrm{11}}&{}\\{\mathrm{12}}&{\:\:\:\:\:\mathrm{16}}&{\:\:\:\:\mathrm{0}}&{}\\{\mathrm{13}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\:\mathrm{13}}&{}\\{\mathrm{14}}&{\:\:\:\:\:\mathrm{16}}&{\:\:\:\:\mathrm{8}}&{}\\{\mathrm{15}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\mathrm{15}}&{}\\{\mathrm{16}}&{\:\:\:\:\:\mathrm{16}}&{\:\:\mathrm{16}}&{{u}={v}}\\{\mathrm{17}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\mathrm{17}}&{}\\{\mathrm{18}}&{\:\:\:\:\:\mathrm{16}}&{\:\:\:\mathrm{0}}&{}\\{\mathrm{19}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\mathrm{19}}&{}\\{\mathrm{20}}&{\:\:\:\:\:\mathrm{16}}&{\:\:\:\mathrm{8}}&{}\\{\mathrm{21}}&{\:\:\:\:\:\:\mathrm{8}}&{\:\:\mathrm{21}}&{}\\{\mathrm{22}}&{\:\:\:\:\mathrm{16}}&{\:\:\mathrm{16}}&{{u}={v}}\\{\mathrm{23}}&{\:\:\:\:\:\:\:\mathrm{8}}&{\:\:\mathrm{23}}&{}\\{\mathrm{24}}&{\:\:\:\:\mathrm{16}}&{\:\:\:\mathrm{0}}&{}\end{bmatrix} \\ $$$${Values}\:{of}\:{n}\:{for}\:{which}\:{u}={v}\:{or} \\ $$$$\mathrm{2}^{{n}} \equiv{n}^{\mathrm{3}} \left({mod}\:\mathrm{24}\right)\:{or}\:{in}\:{other}\:{words} \\ $$$$\mathrm{4}!\:\mid\:\mathrm{2}^{{n}} −{n}^{\mathrm{3}} \:{are}: \\ $$$$\:\mathrm{4},\mathrm{10},\mathrm{16},…\left(\mathrm{6}{n}−\mathrm{2}\right).. \\ $$$$\mathcal{T}{his}\:{is}\:{an}\:{AP}\:{upto}\:\mathrm{100}\:{of}\:{which} \\ $$$${are}\:\mathrm{17}\:{terms}. \\ $$$$\therefore\:\mathrm{4}!\:\mid\:\mathrm{2}^{\boldsymbol{{n}}} −\boldsymbol{{n}}^{\mathrm{3}} \:\boldsymbol{{has}}\:\mathrm{17}\:\boldsymbol{{solutions}} \\ $$$$\:\:\:\:\:\boldsymbol{{for}}\:\boldsymbol{{n}}\in\mathbb{N}\:\wedge\:\boldsymbol{{n}}\leqslant\mathrm{100}. \\ $$
Commented by Aina Samuel Temidayo last updated on 07/Sep/20
Can you please complete it?
$$\mathrm{Can}\:\mathrm{you}\:\mathrm{please}\:\mathrm{complete}\:\mathrm{it}? \\ $$
Commented by Rasheed.Sindhi last updated on 09/Sep/20
The Answer is now complete. Sorry for late.
$$\mathcal{T}{he}\:\mathcal{A}{nswer}\:{is}\:{now}\:\boldsymbol{{complete}}. \\ $$$$\mathcal{S}{orry}\:{for}\:{late}. \\ $$
Commented by Aina Samuel Temidayo last updated on 09/Sep/20
Thanks.
$$\mathrm{Thanks}. \\ $$
Answered by A5T last updated on 15/Jul/25
24∣2^n −n^3 n is even because otherwise 2^n −n^3 is odd. First solution: n=4 ⇒n≥4 ⇒24∣2^(2k) −(2k)^3 =2^(2k) −8k^3 =8(2^(2k−3) −k^3 ) ⇒3∣2^(2k−3) −k^3 Suppose (k,3)=1 Then k^2 ≡1(mod 3)⇒k^3 ≡k(mod 3) ⇒2^(2k−3) −k^3 ≡−1−k(mod 3)≡0(mod 3) ⇒k=3q+2⇒n=2k=6q+4 Suppose (k,3)=3 2^(2k−3) −k^3 ≡−1−0 (contradiction, not ≡0(mod3) ⇒n=6q+4, (4,10,...,100) Total terms: ((100−4)/6)+1=16+1=17
$$\mathrm{24}\mid\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} \\ $$$$\mathrm{n}\:\mathrm{is}\:\mathrm{even}\:\mathrm{because}\:\mathrm{otherwise}\:\mathrm{2}^{\mathrm{n}} −\mathrm{n}^{\mathrm{3}} \:\mathrm{is}\:\mathrm{odd}. \\ $$$$\mathrm{First}\:\mathrm{solution}:\:\mathrm{n}=\mathrm{4}\:\Rightarrow\mathrm{n}\geqslant\mathrm{4} \\ $$$$\Rightarrow\mathrm{24}\mid\mathrm{2}^{\mathrm{2k}} −\left(\mathrm{2k}\right)^{\mathrm{3}} =\mathrm{2}^{\mathrm{2k}} −\mathrm{8k}^{\mathrm{3}} =\mathrm{8}\left(\mathrm{2}^{\mathrm{2k}−\mathrm{3}} −\mathrm{k}^{\mathrm{3}} \right) \\ $$$$\Rightarrow\mathrm{3}\mid\mathrm{2}^{\mathrm{2k}−\mathrm{3}} −\mathrm{k}^{\mathrm{3}} \\ $$$$\mathrm{Suppose}\:\left(\mathrm{k},\mathrm{3}\right)=\mathrm{1} \\ $$$$\mathrm{Then}\:\mathrm{k}^{\mathrm{2}} \equiv\mathrm{1}\left(\mathrm{mod}\:\mathrm{3}\right)\Rightarrow\mathrm{k}^{\mathrm{3}} \equiv\mathrm{k}\left(\mathrm{mod}\:\mathrm{3}\right) \\ $$$$\Rightarrow\mathrm{2}^{\mathrm{2k}−\mathrm{3}} −\mathrm{k}^{\mathrm{3}} \equiv−\mathrm{1}−\mathrm{k}\left(\mathrm{mod}\:\mathrm{3}\right)\equiv\mathrm{0}\left(\mathrm{mod}\:\mathrm{3}\right) \\ $$$$\Rightarrow\mathrm{k}=\mathrm{3q}+\mathrm{2}\Rightarrow\mathrm{n}=\mathrm{2k}=\mathrm{6q}+\mathrm{4} \\ $$$$\mathrm{Suppose}\:\left(\mathrm{k},\mathrm{3}\right)=\mathrm{3} \\ $$$$\mathrm{2}^{\mathrm{2k}−\mathrm{3}} −\mathrm{k}^{\mathrm{3}} \equiv−\mathrm{1}−\mathrm{0}\:\:\:\:\:\:\:\:\left(\mathrm{contradiction},\:\mathrm{not}\:\equiv\mathrm{0}\left(\mathrm{mod3}\right)\right. \\ $$$$\Rightarrow\mathrm{n}=\mathrm{6q}+\mathrm{4},\:\left(\mathrm{4},\mathrm{10},…,\mathrm{100}\right) \\ $$$$\mathrm{Total}\:\mathrm{terms}:\:\frac{\mathrm{100}−\mathrm{4}}{\mathrm{6}}+\mathrm{1}=\mathrm{16}+\mathrm{1}=\mathrm{17} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *