Question Number 14752 by Tinkutara last updated on 04/Jun/17
![Find ordered pair of (x, y) given x, y ∈ [0, 2π] if 3^(sin x + cos y) = 1 and 5^(sin^2 x + cos^2 y) = 5](https://www.tinkutara.com/question/Q14752.png)
Commented by Tinkutara last updated on 04/Jun/17

Commented by 1kanika# last updated on 04/Jun/17
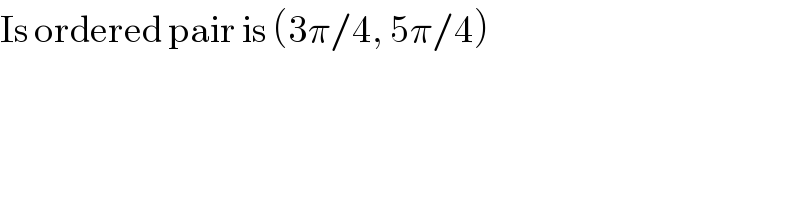
Commented by mrW1 last updated on 04/Jun/17
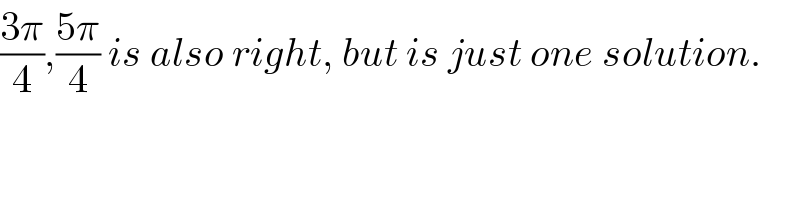
Commented by 1kanika# last updated on 04/Jun/17
Answered by mrW1 last updated on 04/Jun/17
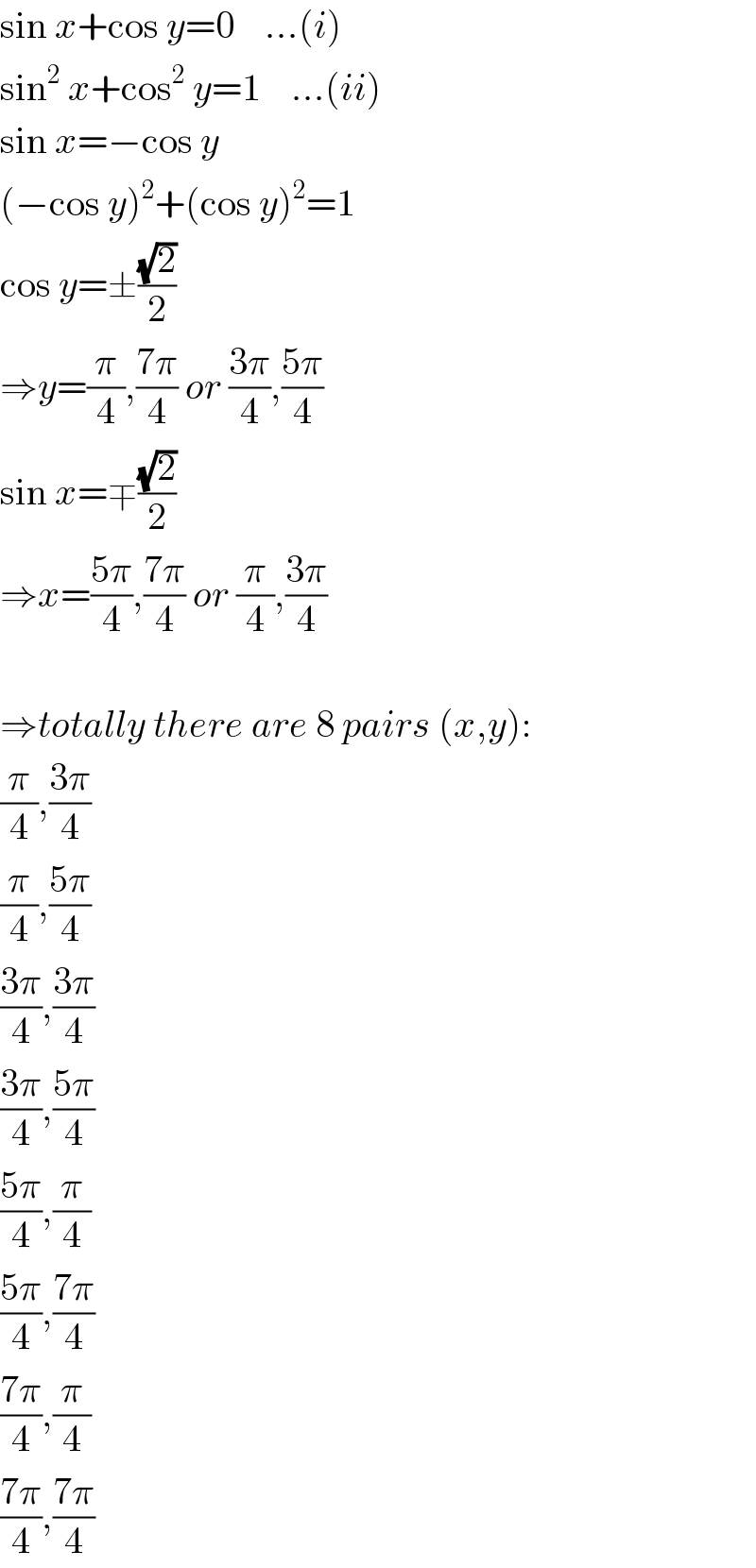
Commented by Tinkutara last updated on 04/Jun/17
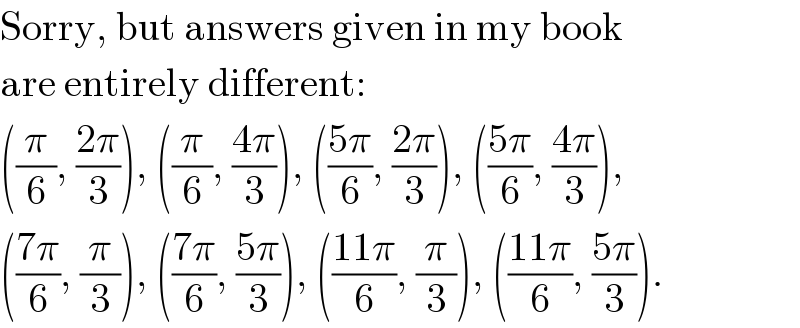
Commented by ajfour last updated on 04/Jun/17
Commented by Tinkutara last updated on 04/Jun/17

Commented by mrW1 last updated on 04/Jun/17

