Find-out-some-pairs-a-b-such-that-for-some-n-1-a-n-b-n-a-2n-b-2n-a-4n-b-4n-a-8n-b-8n-P-

Question Number 160061 by Rasheed.Sindhi last updated on 24/Nov/21

F i n d o u t s o m e p a i r s (a, b) s u c h t h a t

f o r s o m e n ⩾ 1

a^{n} + b^{n}, a^{2 n} + b^{2 n}, a^{4 n} + b^{4 n}, a^{8 n} + b^{8 n} \in P

Answered by nikif99 last updated on 24/Nov/21

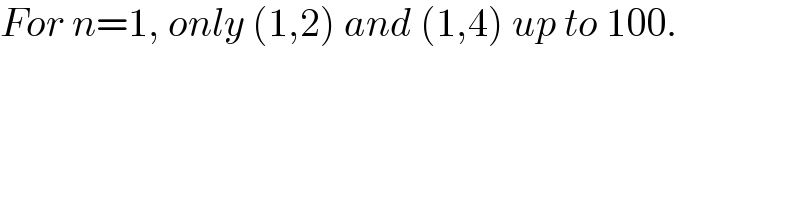
F o r n = 1, o n l y (1, 2) a n d (1, 4) u p t o 100 .

Commented by Rasheed.Sindhi last updated on 24/Nov/21

T h a n X a l o t s i r! Y o u r f i r s t e x a m p l e

i s a n e x a m p l e o f 5 - l e v e l

1 + 2 = 3 \in P

1^{2} + 2^{2} = 5 \in P

1^{4} + 2^{4} = 17 \in P

1^{8} + 2^{8} = 257 \in P

1^{16} + 2^{16} = 65537 \in P

I^{'} v e a l s o s e a r c h e d b u t m y s e a r c h

w a s n o t v e r y s y s t e m a t i c a n d I i g n o r e d

s m a l l n u m b e r s l i k e 1 & 2, s o b e n o t

s u c c e s s f u l! A n y w a y n o w w e c a n s a y t h a t

m a x i m u m n u m b e r o f l e v e l s ⩾ 5

THANKS again for your labour

and attention sir!

BTW, d i d y o u u s e a p r o g r a m f o r

s e a r c h i n g s u c h n u m b e r s ?

Commented by Rasheed.Sindhi last updated on 24/Nov/21

BTW s i r, y o u s e e m e d a n e x p d r i e n c e d

m e m b e r o f t h e f o r u m . A r e y o u r e a l l y

a n o l d m e m b e r o f t h e f o r u m ?

Commented by Rasheed.Sindhi last updated on 24/Nov/21

T h a n k s n i k i f s i r, v e r y m u c h!

Commented by nikif99 last updated on 24/Nov/21

P r o g r a m m i n g i n P a s c a l (d i n o s a u r e a s

p r o g r a m m i n g l a n g u a g e) . A l t h o u g h

u s e f u l i n c o m p u t i n g 8 t h p o w e r .

I r e c e n t l y j o i n e d t h i s g r o u p . I a m a

h o b b i s t o f m a t h .

Commented by Rasheed.Sindhi last updated on 25/Nov/21

A t r i v i a l e x a m p l e o f i n f i n i t y - l e v e l

i s p a i r i s (1, 1) . B e c a u s e 1^{n} + 1^{n} = 2 \in P

\forall n \in N