Question Number 148639 by tabata last updated on 29/Jul/21

$${find}\:{singular}\:{point}\:{of}\:{this}\:{following}\:{and} \\ $$$${whats}\:{the}\:{type}\:{of}\:{singular}\:{point}\:? \\ $$$$ \\ $$$$\left(\mathrm{1}\right){f}\left({z}\right)=\frac{\mathrm{1}}{{lnz}} \\ $$$$ \\ $$$$\left(\mathrm{2}\right){f}\left({z}\right)=\frac{\mathrm{1}−{cos}\left({z}+{i}\right)}{{z}\left({z}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$ \\ $$$$\left(\mathrm{3}\right){f}\left({z}\right)=\frac{{sinz}}{{z}^{\mathrm{2}} +{z}} \\ $$$$ \\ $$$$\left(\mathrm{4}\right){f}\left({z}\right)=\frac{{sin}\mathrm{2}{z}}{{z}^{\mathrm{2}} } \\ $$
Answered by mathmax by abdo last updated on 30/Jul/21
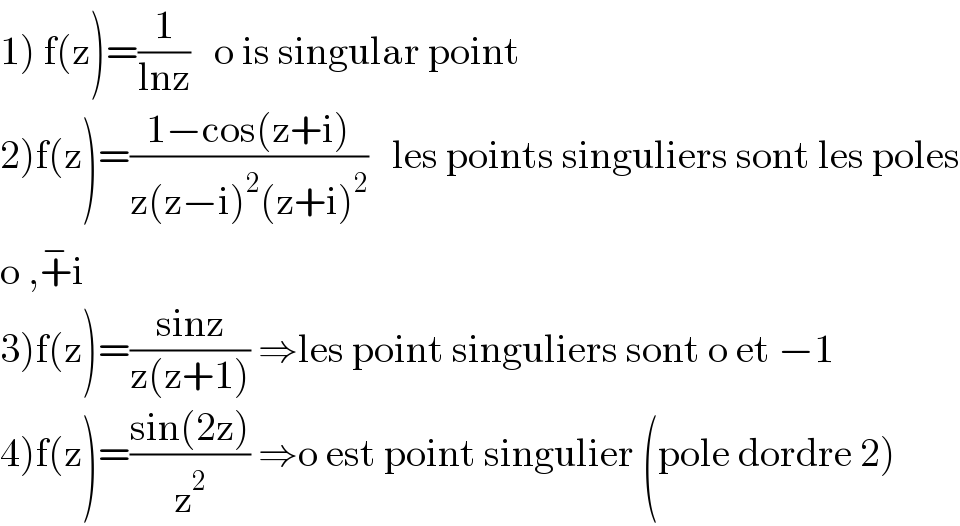
$$\left.\mathrm{1}\right)\:\mathrm{f}\left(\mathrm{z}\right)=\frac{\mathrm{1}}{\mathrm{lnz}}\:\:\:\mathrm{o}\:\mathrm{is}\:\mathrm{singular}\:\mathrm{point} \\ $$$$\left.\mathrm{2}\right)\mathrm{f}\left(\mathrm{z}\right)=\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{z}+\mathrm{i}\right)}{\mathrm{z}\left(\mathrm{z}−\mathrm{i}\right)^{\mathrm{2}} \left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{2}} }\:\:\:\mathrm{les}\:\mathrm{points}\:\mathrm{singuliers}\:\mathrm{sont}\:\mathrm{les}\:\mathrm{poles} \\ $$$$\mathrm{o}\:,\overset{−} {+}\mathrm{i} \\ $$$$\left.\mathrm{3}\right)\mathrm{f}\left(\mathrm{z}\right)=\frac{\mathrm{sinz}}{\mathrm{z}\left(\mathrm{z}+\mathrm{1}\right)}\:\Rightarrow\mathrm{les}\:\mathrm{point}\:\mathrm{singuliers}\:\mathrm{sont}\:\mathrm{o}\:\mathrm{et}\:−\mathrm{1} \\ $$$$\left.\mathrm{4}\right)\mathrm{f}\left(\mathrm{z}\right)=\frac{\mathrm{sin}\left(\mathrm{2z}\right)}{\mathrm{z}^{\mathrm{2}} }\:\Rightarrow\mathrm{o}\:\mathrm{est}\:\mathrm{point}\:\mathrm{singulier}\:\left(\mathrm{pole}\:\mathrm{dordre}\:\mathrm{2}\right) \\ $$
Commented by Sozan last updated on 30/Jul/21
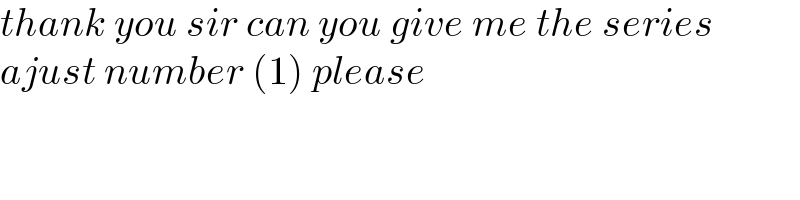
$${thank}\:{you}\:{sir}\:{can}\:{you}\:{give}\:{me}\:{the}\:{series}\: \\ $$$${ajust}\:{number}\:\left(\mathrm{1}\right)\:{please} \\ $$
