find-the-distance-of-point-2-1-2-to-plane-passing-through-points-1-2-3-0-4-2-and-1-3-4-

Question Number 118747 by bemath last updated on 19/Oct/20

f i n d t h e d i s t a n c e o f p o i n t (2, 1, - 2) t o p l a n e

p a s s i n g t h r o u g h p o i n t s (- 1, 2, - 3);

(0, - 4, - 2) a n d (1, 3, 4) .

Answered by benjo_mathlover last updated on 19/Oct/20

E q u a t i o n o f p l a n e p a s s i n g t h r o u g h p o i n t s

(x_{1}, y_{1}, z_{1}); (x_{2}, y_{2}, z_{2}); (x_{3}, y_{3}, z_{3}) i s

| \begin{matrix} x - x_{1} y - y_{1} z - z_{1} \\ x_{2} - x_{1} y_{2} - y_{1} z_{2} - z_{1} \\ x_{3} - x_{1} y_{3} - y_{1} z_{3} - z_{1} \end{matrix} | = 0

s o w e g e t e q u a t i o n o f p l a n e i s

| \begin{matrix} x + 1 y - 2 z + 3 \\ 1 - 6 1 \\ 2 1 7 \end{matrix} | = 0

(x + 1) (- 43) - (y - 2) (5) + (z + 3) (13) = 0

- 43 x - 43 - 5 y + 10 + 13 z + 39 = 0

\Rightarrow 43 x + 5 y - 13 z - 6 = 0

W e w a n t t o c o m p u t e t h e d i s t a n c e o f

p o i n t (2, 1, - 2) t o p l a n e 43 x + 5 y - 13 z - 6 = 0

b y t h e f o r m u l a \Rightarrow d = \frac{∣ 43 (2) + 5 (1) - 13 (- 2) - 6 ∣}{\sqrt{43^{2} + 5^{2} + {(- 13)}^{2}}}

\Rightarrow d = \frac{111}{\sqrt{2043}}

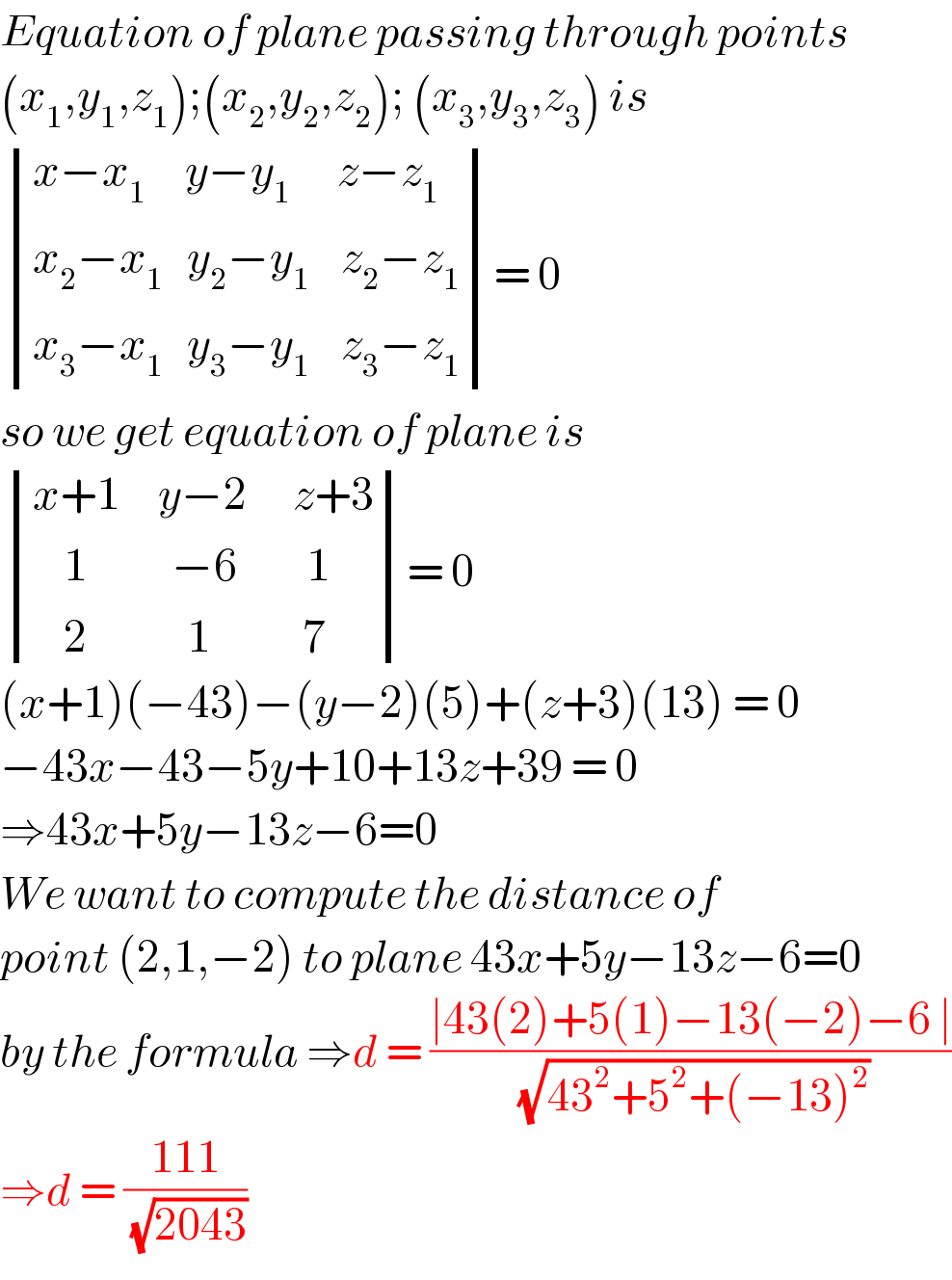
Answered by 1549442205PVT last updated on 19/Oct/20

Suppose A (- 1, 2, - 3), B (0, - 4, - 2)

C (1, 3, 4) \Rightarrow \vec{AB} = (1, - 6, 1), \vec{AC} = (2, 1, 7)

The normal vector of the plane P is

\vec{n} = \vec{AB} \times \vec{AC} = ((\begin{matrix} - 6 & 1 \\ 1 & 7 \end{matrix}), (\begin{matrix} 1 & 1 \\ 7 & 2 \end{matrix}), (\begin{matrix} 1 & - 6 \\ 2 & 1 \end{matrix}))

= (- 43, - 5, 13) . Therefore, the equation of

P pass through A (- 1, 2, - 3) with the

normal \vec{n} = (- 44, - 5, 13) is :

- 43 (x + 1) - 5 (y - 2) + 13 (z + 3) = 0

\Leftrightarrow - 43 x - 5 y + 13 z + 6 = 0

The distance from the pointM (2, 1, - 2)

to P is MH = \frac{∣ - 43.2 - 5.1 + 13 (- 2) + 6 ∣}{\sqrt{43^{2} + 5^{2} + 13^{2}}}

= \frac{111}{3 \sqrt{227}}

Answered by mr W last updated on 19/Oct/20

Commented by mr W last updated on 19/Oct/20

P (2, 1, - 2)

A (- 1, 2, - 3)

B (0, - 4, - 2)

C (1, 3, 4)

\vec{A B} = (1, - 6, 1)

\vec{A C} = (2, 1, 7)

\vec{A D} = \vec{A B} \times \vec{A C} = (1, - 6, 1) \times (2, 1, 7)

= (- 43, - 5, 13)

\vec{A P} = (3, - 1, 1)

{P P}^{'} = A Q = \frac{∣ \vec{A P} \cdot \vec{A D} ∣}{∣ \vec{A D} ∣}

= \frac{∣ (3, - 1, 1) \cdot (- 43, - 5, 13) ∣}{\sqrt{{(- 43)}^{2} + {(- 5)}^{2} + 13^{2}}}

= \frac{∣ - 3 \times 43 + 1 \times 5 + 1 \times 13 ∣}{3 \sqrt{227}}

= \frac{37}{\sqrt{227}}

Answered by ajfour last updated on 19/Oct/20

O A = - i + 2 j - 3 k

O B = - 4 j - 2 k

O C = i + 3 j + 4 k

A B = i - 6 j + k; B C = i + 7 j + 6 k

\bar{n} = A B \times B C = | \begin{matrix} i & j & k \\ 1 & - 6 & 1 \\ 1 & 7 & 6 \end{matrix} |

= - 43 i - 5 j + 13 k

d i s t a n c e o f P (2, 1, - 2) w e f i r s t

f i n d A P = 3 i - j + k . N o w

= \frac{A P . \bar{n}}{∣ \bar{n} ∣} = ∣ \frac{(3 i - j + k) . (- 43 i - 5 j + 13 k)}{\sqrt{43^{2} + 5^{2} + 13^{2}}} ∣

= \frac{∣ - 129 + 5 + 13 ∣}{\sqrt{2043}} = \frac{111}{\sqrt{2043}} = \frac{37}{\sqrt{227}} .

Facebook Tweet Pin