find-the-equations-of-two-circles-which-thier-center-both-2-2-and-tangent-with-circle-x-2-y-2-8x-10y-5-0-

Question Number 123393 by malwan last updated on 25/Nov/20

f i n d t h e e q u a t i o n s o f [t w o] c i r c l e s

w h i c h t h i e r c e n t e r b o t h (2, - 2)

a n d t a n g e n t w i t h c i r c l e

x^{2} + y^{2} - 8 x + 10 y + 5 = 0

Commented by malwan last updated on 25/Nov/20

c a n y o u d r o w i t s i r ?

Commented by MJS_new last updated on 25/Nov/20

sorry I have no drawing app

Commented by talminator2856791 last updated on 25/Nov/20

you can use this app drawing tool

Commented by malwan last updated on 25/Nov/20

c a n y o u t r y s i r ?

Commented by MJS_new last updated on 25/Nov/20

sorry I never used it and I {don}^{'} t have the time

to get used to it . but {it}^{'} s easy to sketch

Commented by talminator2856791 last updated on 25/Nov/20

yes i tried but it is difficult to use this

Answered by talminator2856791 last updated on 25/Nov/20

x^{2} + y^{2} - 8 x + 10 y + 5 = {(x - 4)}^{2} + {(y + 5)}^{2} + 5 - 16 - 25

{(x - 4)}^{2} + {(y + 5)}^{2} = r^{2} = 36

r = 6

r (4; - 5)

distance between centres d

d = \sqrt{2^{2} + 3^{2}}

d = \sqrt{13}

\sqrt{13} ≪ r

equations of circles :

{(x - 2)}^{2} + {(y + 2)}^{2} = {(6 - \sqrt{13})}^{2}

{(x - 2)}^{2} + {(y + 2)}^{2} = {(6 + \sqrt{13})}^{2}

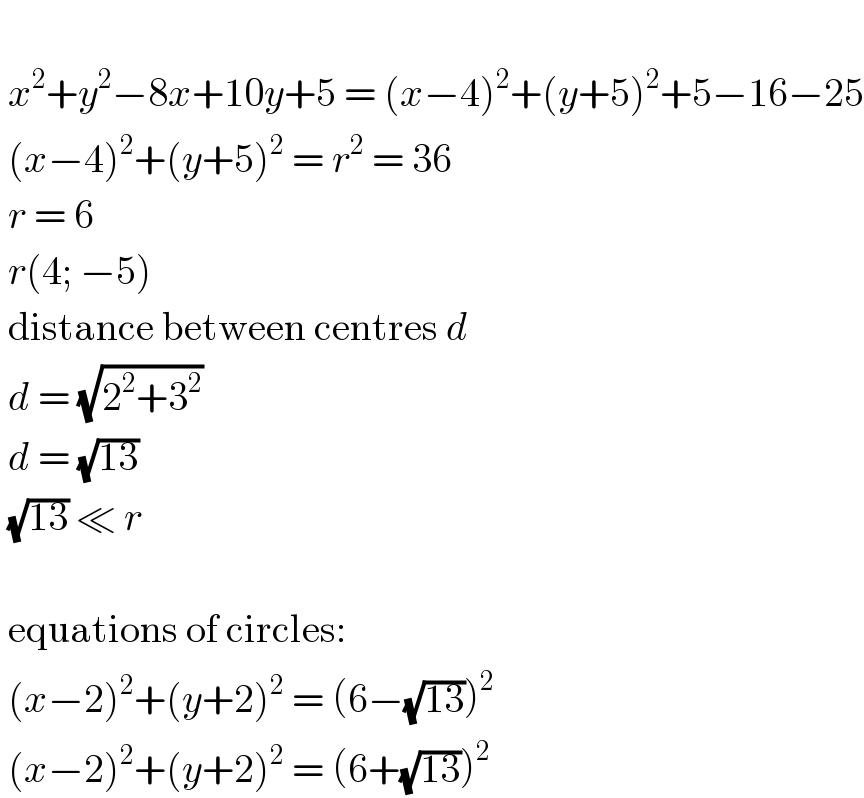
Commented by malwan last updated on 25/Nov/20

t h a n k y o u s i r

Answered by MJS_new last updated on 25/Nov/20

(1) {(x - 2)}^{2} + {(y + 2)}^{2} - r^{2} = 0

(2) x^{2} + y^{2} - 8 x + 10 y + 5 = 0

(1) x^{2} + y^{2} - 4 x + 4 y + 8 - r^{2} = 0

(1) - (2) 4 x - 6 y + 3 - r^{2} = 0 \Rightarrow y = \frac{4 x - r^{2} + 3}{6}

insert in (1) or (2)

\frac{13}{9} x^{2} - \frac{2 (r^{2} + 3)}{9} + \frac{r^{4} - 66 r^{2} + 369}{36} = 0

x^{2} - \frac{2 (o^{2} + 3)}{13} x + \frac{r^{4} - 66 r^{2} + 369}{52} = 0

tangenting circles means we must find r in

order to get a ? double solution for x

x^{2} + p x + q = 0 \Rightarrow x = - \frac{p}{2} \pm \sqrt{\frac{p^{2}}{4} - q}

we need \frac{p^{2}}{4} - q = 0

\Rightarrow \frac{9}{676} (r^{4} - 98 r^{2} + 529) = 0

\Rightarrow r = 6 \pm \sqrt{13} [r > 0]

\Rightarrow

circle 1 : {(x - 2)}^{2} + {(y + 2)}^{2} + 49 - 12 \sqrt{13} = 0

circle 2 : {(x - 2)}^{2} + {(y + 2)}^{2} + 49 + 12 \sqrt{13} = 0

Commented by malwan last updated on 25/Nov/20

t h a n k y o u s o m u c h s i r

Commented by peter frank last updated on 25/Nov/20

thank you