Question Number 178694 by peter frank last updated on 20/Oct/22
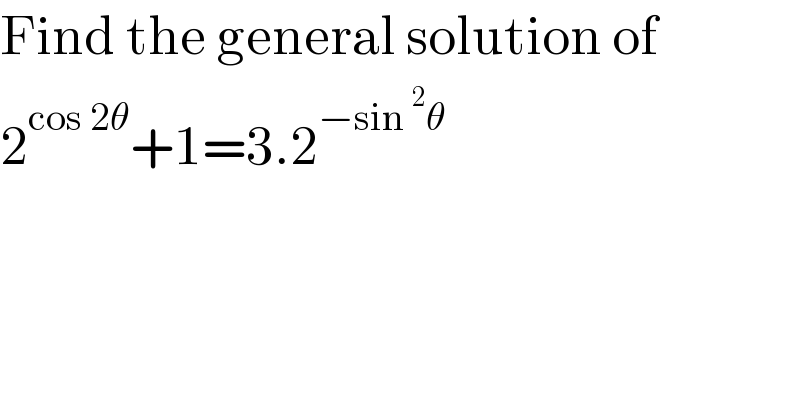
Answered by Frix last updated on 20/Oct/22

Commented by Ar Brandon last updated on 20/Oct/22
Welldone sir MJS��������
Commented by Rasheed.Sindhi last updated on 21/Oct/22

Commented by Ar Brandon last updated on 21/Oct/22
������
Commented by ARUNG_Brandon_MBU last updated on 21/Oct/22




Commented by ARUNG_Brandon_MBU last updated on 21/Oct/22
Q177400
Commented by Rasheed.Sindhi last updated on 21/Oct/22
Commented by ARUNG_Brandon_MBU last updated on 21/Oct/22
����
Commented by MJS_new last updated on 22/Oct/22

Commented by ARUNG_Brandon_MBU last updated on 22/Oct/22
�� oh! "Her Majesty had been my personal defense against the weirdness which had been creeping into this forum in those days"
But back then:-
Her Majesty: "I have told you several times I am not MJS believe it or not."
���� You're funny, Sir. You made my day ��
Commented by Rasheed.Sindhi last updated on 22/Oct/22

Commented by ARUNG_Brandon_MBU last updated on 22/Oct/22
���� Nice week-end to you, Sir RS ! Thanks for your company.
Commented by Rasheed.Sindhi last updated on 22/Oct/22
��������
Commented by peter frank last updated on 22/Oct/22

Commented by Frix last updated on 24/Oct/22

