Find-the-limit-x-0-lim-tan-x-x-1-x-2-

Question Number 82494 by niroj last updated on 21/Feb/20

Find the limit

\underset{x \to 0}{\overset{\lim}{}} {(\frac{\tan x}{x})}^{\frac{1}{x^{2}}}

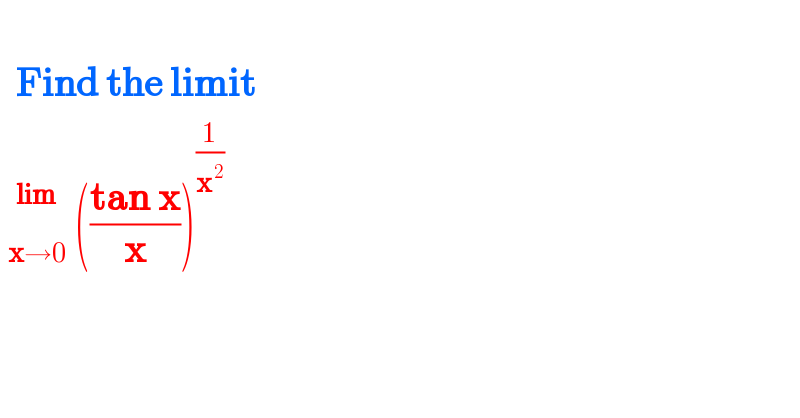
Commented by abdomathmax last updated on 21/Feb/20

l e t f (x) = {(\frac{t a n x}{x})}^{\frac{1}{x^{2}}} \Rightarrow f (x) = e^{\frac{1}{x^{2}} l n (\frac{t a n x}{x})}

u (x) = t a n x = u (0) + \frac{x}{1!} u^{(1)} (o) + \frac{x^{2}}{2!} u^{(2)} (0) + \frac{x^{3}}{3!} u^{(3)} (0) + \dots

u^{^{'}} (x) = 1 + {t a n}^{2} x \Rightarrow u^{^{'}} (0) = 1

u^{(2)} (x) = 2 t a n x (1 + {t a n}^{2} x) \Rightarrow u^{(2)} (0) = 0

u^{(3)} (x) = 2 (1 + {t a n}^{2} x) (1 + {t a n}^{2} x) +

+ (2 t a n x) (2 t a n x (1 + {t a n}^{2} x)) \Rightarrow u^{(3)} (0) = 2 \Rightarrow

t a n x = x + \frac{x^{3}}{3} + o (x^{5}) \Rightarrow \frac{t a n x}{x} = 1 + \frac{x^{2}}{3} + o (x^{4}) \Rightarrow

l n (\frac{t a n x}{x}) = l n (1 + \frac{x^{2}}{3} + o (x^{4})) \sim \frac{x^{2}}{3} \Rightarrow

\frac{1}{x^{2}} l n (\frac{t a n x}{x}) \sim \frac{1}{3} \Rightarrow {l i m}_{x \to 0} f (x) = e^{\frac{1}{3}} =^{3} \sqrt{e}

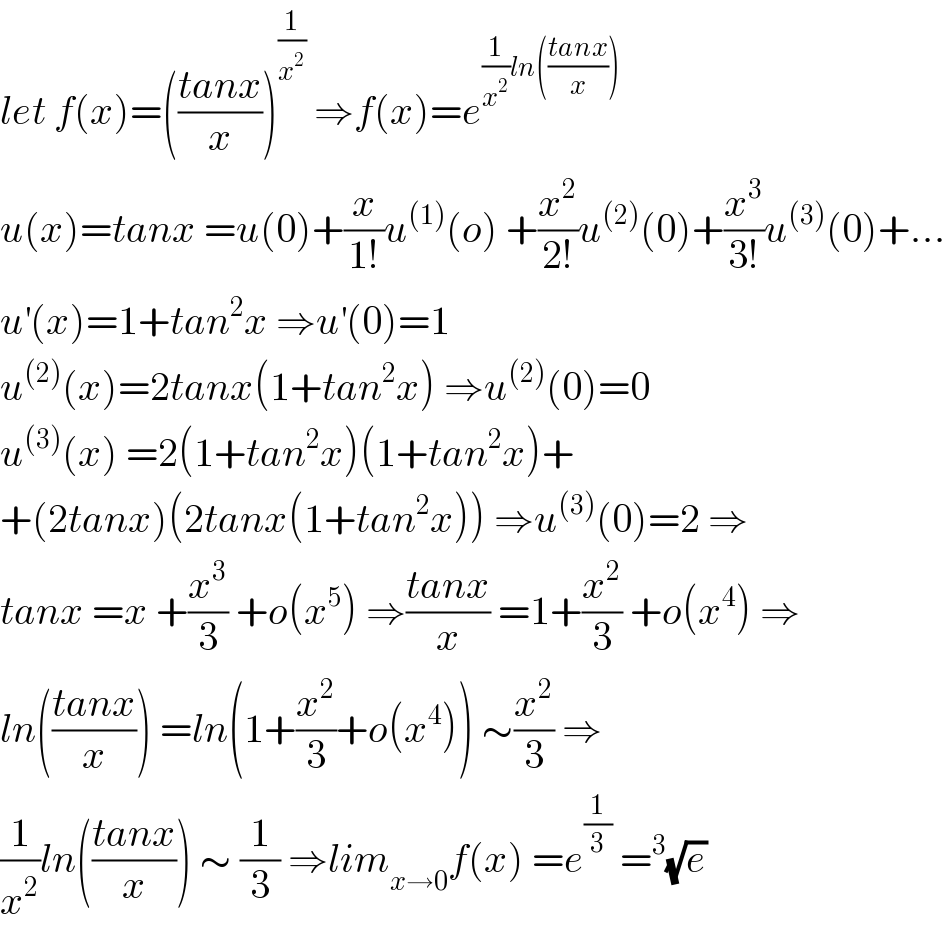
Commented by jagoll last updated on 22/Feb/20

\lim_{x \to 0} {(1 + \frac{\tan x}{x} - 1)}^{\frac{1}{x^{2}}} =

\lim_{x \to 0} {(1 + \frac{\tan x - x}{x})}^{\frac{1}{x^{2}}} =

e^{\lim_{x \to 0} (\frac{\tan x - x}{x^{3}})} = e^{\lim_{x \to 0} (\frac{\sec^{2} x - 1}{3 x^{2}})}

= e^{\lim_{x \to 0} (\frac{1 - \cos^{2} x}{3 x^{2} \cos^{2} x})} = e^{\frac{1}{3}}

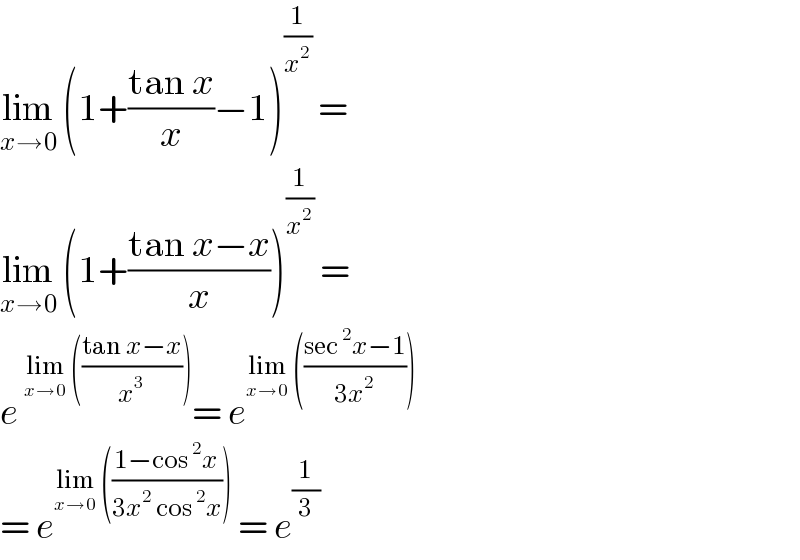
Commented by john santu last updated on 22/Feb/20

n i c e

Commented by niroj last updated on 22/Feb/20

g r e a t .

Facebook Tweet Pin