Question Number 15741 by ajfour last updated on 13/Jun/17
$${Find}\:{the}\:{minimum}\:{value}\:\:{of} \\ $$$$\:\:\boldsymbol{{x}}^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{2}} +\boldsymbol{{z}}^{\mathrm{2}} ,\:{with}\:{the}\:{condition} \\ $$$$\:{ax}+{by}+{cz}={p}\:. \\ $$
Answered by mrW1 last updated on 13/Jun/17
$$\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} }\:\mathrm{is}\:\mathrm{the}\:\mathrm{distance}\:\mathrm{of}\:\mathrm{a}\:\mathrm{point} \\ $$$$\left(\mathrm{x},\mathrm{y},\mathrm{z}\right)\:\mathrm{on}\:\mathrm{the}\:\mathrm{plane}\:{ax}+{by}+{cz}−{p}=\mathrm{0} \\ $$$$\mathrm{to}\:\mathrm{the}\:\mathrm{origin}\:\left(\mathrm{0},\mathrm{0},\mathrm{0}\right).\: \\ $$$$ \\ $$$$\mathrm{since}\:\mathrm{the}\:\mathrm{distance}\:\mathrm{from}\:\left(\mathrm{0},\mathrm{0},\mathrm{0}\right)\:\mathrm{to}\:\mathrm{the}\:\mathrm{plane} \\ $$$${ax}+{by}+{cz}−\mathrm{p}\:\mathrm{is} \\ $$$$\mathrm{d}=\frac{\mid\mathrm{p}\mid}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} }} \\ $$$$ \\ $$$$\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} }\:\geqslant\mathrm{d} \\ $$$$\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} \geqslant\mathrm{d}^{\mathrm{2}} =\frac{\mathrm{p}^{\mathrm{2}} }{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} } \\ $$
Commented by ajfour last updated on 13/Jun/17
$${good}\:{viewpoint}\:{sir},\:{i}\:{thought} \\ $$$${it}\:{would}\:{again}\:{involve}\:{partial} \\ $$$${derivatives}. \\ $$$$ \\ $$
Commented by mrW1 last updated on 13/Jun/17
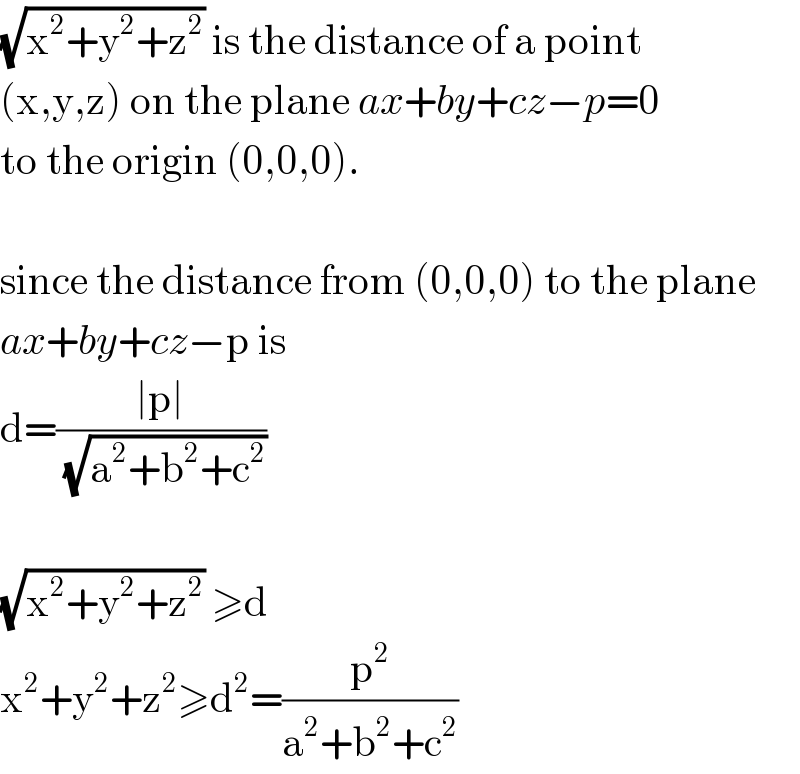
![Solution in conventional way: z=((p−(ax+by))/c)=p′−(a′x+b′y) with p′=(p/c), a′=(a/c), b′=(b/c) S=x^2 +y^2 +z^2 =x^2 +y^2 +p′^2 −2p′(a′x+b′y)+(a′x+b′y)^2 =x^2 +y^2 +p′^2 −2p′(a′x+b′y)+a′^2 x^2 +b′^2 y^2 +2a′b′xy =(1+a′^2 )x^2 +(1+b′^2 )y^2 +2a′b′xy−2p′a′x−2p′b′y+p′^2 (∂S/∂x)=2(1+a′^2 )x+2a′b′y−2p′a′=0 ⇒(1+a′^2 )x+a′b′y=p′a′ (∂S/∂y)=2(1+b′^2 )y+2a′b′x−2p′b′=0 ⇒(1+b′^2 )y+a′b′x=p′b′ ⇒y=((p′b′)/((1+b′^2 )))−((a′b′)/((1+b′^2 )))x ⇒(1+a′^2 )x−(((a′b′)^2 )/((1+b′^2 )))x=p′a′−((p′a′b′^2 )/((1+b′^2 ))) ⇒(((1+a′^2 )(1+b′^2 )−(a′b′)^2 )/((1+b′^2 )))x=((p′a′)/((1+b′^2 ))) ⇒x=((p′a′)/((1+a′^2 )(1+b′^2 )−(a′b′)^2 ))=((p′a′)/(1+a′^2 +b′^2 )) ⇒y=((p′b′)/((1+a′^2 )(1+b′^2 )−(a′b′)^2 ))=((p′b′)/(1+a′^2 +b′^2 )) S_(min) =(1+a′^2 )(((p′a′)/(1+a′^2 +b′^2 )))^2 +(1+b′^2 )(((p′b′)/(1+a′^2 +b′^2 )))^2 +2a′b′(((p′a′)/(1+a′^2 +b′^2 )))(((p′b′)/(1+a′^2 +b′^2 )))−2p′a′(((p′a′)/(1+a′^2 +b′^2 )))−2p′b′(((p′b′)/(1+a′^2 +b′^2 )))+p′^2 =(1/((1+a′^2 +b′^2 )^2 ))[(1+a′^2 )p′^2 a′^2 +(1+b′^2 )p′^2 b′^2 +2p′^2 a′^2 b′^2 −2p′^2 (a′^2 +b′^2 )(1+a′^2 +b′^2 )+p′^2 (1+a′^2 +b′^2 )^2 ] =((p′^2 )/((1+a′^2 +b′^2 )^2 ))[(a′^2 +b′^2 )(1+a′^2 +b′^2 )+(1−a′^2 −b′^2 )(1+a′^2 +b′^2 )] =((p′^2 )/((1+a′^2 +b′^2 )^2 ))[a′^2 +b′^2 +1−a′^2 −b′^2 ](1+a′^2 +b′^2 ) =((p′^2 )/(1+a′^2 +b′^2 )) =((p^2 /c^2 )/(1+(a^2 /c^2 )+(b^2 /c^2 )))=(p^2 /(a^2 +b^2 +c^2 ))](https://www.tinkutara.com/question/Q15774.png)
$$\mathrm{Solution}\:\mathrm{in}\:\mathrm{conventional}\:\mathrm{way}: \\ $$$$ \\ $$$$\mathrm{z}=\frac{\mathrm{p}−\left(\mathrm{ax}+\mathrm{by}\right)}{\mathrm{c}}=\mathrm{p}'−\left(\mathrm{a}'\mathrm{x}+\mathrm{b}'\mathrm{y}\right) \\ $$$$\mathrm{with}\:\mathrm{p}'=\frac{\mathrm{p}}{\mathrm{c}},\:\mathrm{a}'=\frac{\mathrm{a}}{\mathrm{c}},\:\mathrm{b}'=\frac{\mathrm{b}}{\mathrm{c}} \\ $$$$ \\ $$$$\mathrm{S}=\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} =\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{p}'^{\mathrm{2}} −\mathrm{2p}'\left(\mathrm{a}'\mathrm{x}+\mathrm{b}'\mathrm{y}\right)+\left(\mathrm{a}'\mathrm{x}+\mathrm{b}'\mathrm{y}\right)^{\mathrm{2}} \\ $$$$=\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{p}'^{\mathrm{2}} −\mathrm{2p}'\left(\mathrm{a}'\mathrm{x}+\mathrm{b}'\mathrm{y}\right)+\mathrm{a}'^{\mathrm{2}} \mathrm{x}^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \mathrm{y}^{\mathrm{2}} +\mathrm{2a}'\mathrm{b}'\mathrm{xy} \\ $$$$=\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\mathrm{x}^{\mathrm{2}} +\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)\mathrm{y}^{\mathrm{2}} +\mathrm{2a}'\mathrm{b}'\mathrm{xy}−\mathrm{2p}'\mathrm{a}'\mathrm{x}−\mathrm{2p}'\mathrm{b}'\mathrm{y}+\mathrm{p}'^{\mathrm{2}} \\ $$$$ \\ $$$$\frac{\partial\mathrm{S}}{\partial\mathrm{x}}=\mathrm{2}\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\mathrm{x}+\mathrm{2a}'\mathrm{b}'\mathrm{y}−\mathrm{2p}'\mathrm{a}'=\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\mathrm{x}+\mathrm{a}'\mathrm{b}'\mathrm{y}=\mathrm{p}'\mathrm{a}' \\ $$$$ \\ $$$$\frac{\partial\mathrm{S}}{\partial\mathrm{y}}=\mathrm{2}\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)\mathrm{y}+\mathrm{2a}'\mathrm{b}'\mathrm{x}−\mathrm{2p}'\mathrm{b}'=\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)\mathrm{y}+\mathrm{a}'\mathrm{b}'\mathrm{x}=\mathrm{p}'\mathrm{b}' \\ $$$$ \\ $$$$\Rightarrow\mathrm{y}=\frac{\mathrm{p}'\mathrm{b}'}{\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)}−\frac{\mathrm{a}'\mathrm{b}'}{\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)}\mathrm{x} \\ $$$$\Rightarrow\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\mathrm{x}−\frac{\left(\mathrm{a}'\mathrm{b}'\right)^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)}\mathrm{x}=\mathrm{p}'\mathrm{a}'−\frac{\mathrm{p}'\mathrm{a}'\mathrm{b}'^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)} \\ $$$$\Rightarrow\frac{\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)−\left(\mathrm{a}'\mathrm{b}'\right)^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)}\mathrm{x}=\frac{\mathrm{p}'\mathrm{a}'}{\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)} \\ $$$$\Rightarrow\mathrm{x}=\frac{\mathrm{p}'\mathrm{a}'}{\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)−\left(\mathrm{a}'\mathrm{b}'\right)^{\mathrm{2}} }=\frac{\mathrm{p}'\mathrm{a}'}{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} } \\ $$$$\Rightarrow\mathrm{y}=\frac{\mathrm{p}'\mathrm{b}'}{\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)−\left(\mathrm{a}'\mathrm{b}'\right)^{\mathrm{2}} }=\frac{\mathrm{p}'\mathrm{b}'}{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} } \\ $$$$ \\ $$$$\mathrm{S}_{\mathrm{min}} =\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\left(\frac{\mathrm{p}'\mathrm{a}'}{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} }\right)^{\mathrm{2}} +\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)\left(\frac{\mathrm{p}'\mathrm{b}'}{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} }\right)^{\mathrm{2}} +\mathrm{2a}'\mathrm{b}'\left(\frac{\mathrm{p}'\mathrm{a}'}{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} }\right)\left(\frac{\mathrm{p}'\mathrm{b}'}{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} }\right)−\mathrm{2p}'\mathrm{a}'\left(\frac{\mathrm{p}'\mathrm{a}'}{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} }\right)−\mathrm{2p}'\mathrm{b}'\left(\frac{\mathrm{p}'\mathrm{b}'}{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} }\right)+\mathrm{p}'^{\mathrm{2}} \\ $$$$=\frac{\mathrm{1}}{\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)^{\mathrm{2}} }\left[\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} \right)\mathrm{p}'^{\mathrm{2}} \mathrm{a}'^{\mathrm{2}} +\left(\mathrm{1}+\mathrm{b}'^{\mathrm{2}} \right)\mathrm{p}'^{\mathrm{2}} \mathrm{b}'^{\mathrm{2}} +\mathrm{2p}'^{\mathrm{2}} \mathrm{a}'^{\mathrm{2}} \mathrm{b}'^{\mathrm{2}} −\mathrm{2p}'^{\mathrm{2}} \left(\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)+\mathrm{p}'^{\mathrm{2}} \left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)^{\mathrm{2}} \right] \\ $$$$=\frac{\mathrm{p}'^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)^{\mathrm{2}} }\left[\left(\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)+\left(\mathrm{1}−\mathrm{a}'^{\mathrm{2}} −\mathrm{b}'^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)\right] \\ $$$$=\frac{\mathrm{p}'^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right)^{\mathrm{2}} }\left[\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} +\mathrm{1}−\mathrm{a}'^{\mathrm{2}} −\mathrm{b}'^{\mathrm{2}} \right]\left(\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} \right) \\ $$$$=\frac{\mathrm{p}'^{\mathrm{2}} }{\mathrm{1}+\mathrm{a}'^{\mathrm{2}} +\mathrm{b}'^{\mathrm{2}} } \\ $$$$=\frac{\frac{\mathrm{p}^{\mathrm{2}} }{\mathrm{c}^{\mathrm{2}} }}{\mathrm{1}+\frac{\mathrm{a}^{\mathrm{2}} }{\mathrm{c}^{\mathrm{2}} }+\frac{\mathrm{b}^{\mathrm{2}} }{\mathrm{c}^{\mathrm{2}} }}=\frac{\mathrm{p}^{\mathrm{2}} }{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} } \\ $$
Commented by mrW1 last updated on 13/Jun/17
$$\mathrm{this}\:\mathrm{way}\:\mathrm{is}\:\mathrm{lengthy},\:\mathrm{but}\:\mathrm{the}\:\mathrm{result}\:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{same}. \\ $$