Find-the-nth-term-100-92-76-44-

Question Number 62664 by Cheyboy last updated on 24/Jun/19

F i n d t h e n t h t e r m

100, 92, 76, 44, \dots \dots

Answered by MJS last updated on 24/Jun/19

a_{n} = 108 - 4 \times 2^{n}

a_{n} = - \frac{4}{3} n^{3} + 4 n^{2} - \frac{32}{3} n + 108

\dots

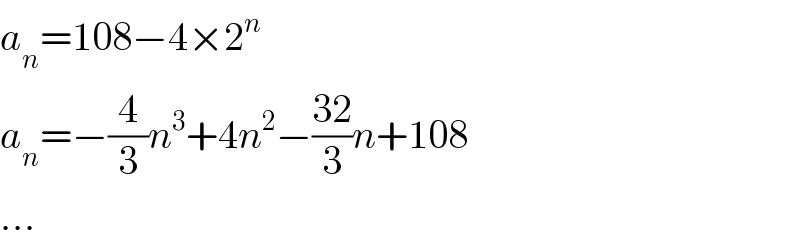
Commented by ajfour last updated on 24/Jun/19

wonderful sir! how to expect and judge the first one?

Commented by mr W last updated on 24/Jun/19

100 = 104 - 2^{2}

92 = 100 - 2^{3} = 104 - (2^{2} + 2^{3})

76 = 92 - 2^{4} = 104 - (2^{2} + 2^{3} + 2^{4})

\dots

a_{n} = 104 - (2^{2} + 2^{3} + 2^{4} + \dots + 2^{n + 1})

= 104 - \frac{2^{2} (2^{n} - 1)}{2 - 1} = 108 - 2^{n + 2}

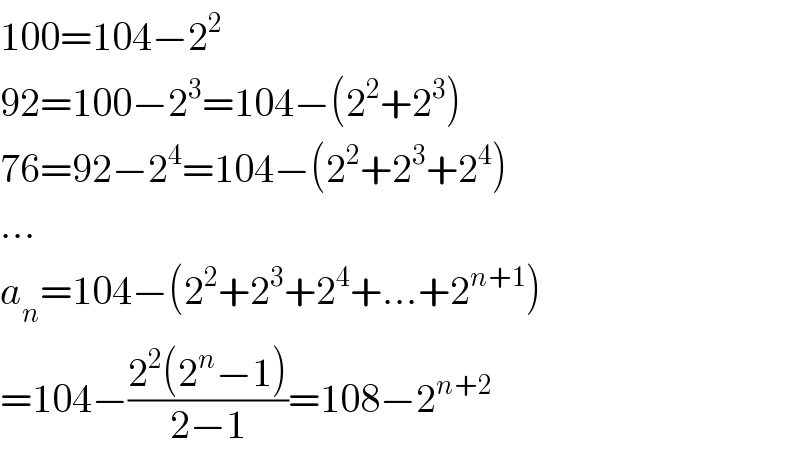
Commented by MJS last updated on 24/Jun/19

we can solve

\underset{i = 1}{\sum^{4}} c_{i} = 100

\underset{i = 1}{\sum^{4}} c_{i}^{2} = 92

\underset{i = 1}{\sum^{4}} c_{i}^{3} = 76

\underset{i = 1}{\sum^{4}} c_{i}^{4} = 44

using c_{1, 2} = α \pm \sqrt{β}; c_{3, 4} = γ \pm \sqrt{δ}

\Rightarrow a_{n} = \underset{i = 1}{\sum^{4}} c_{i}^{n}

but the solution {doesn}^{'} t look very nice

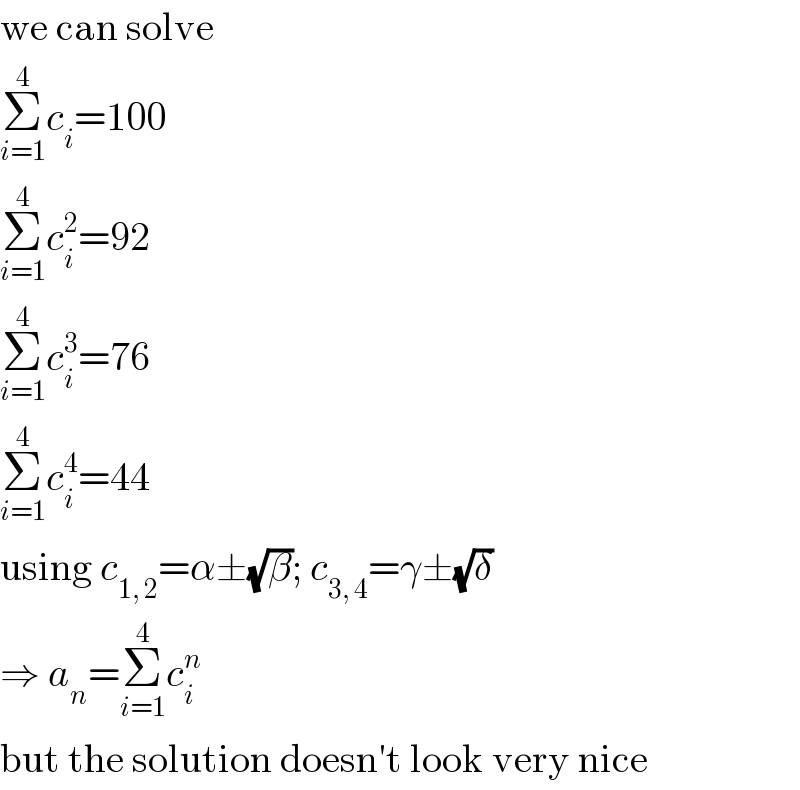
Commented by Cheyboy last updated on 25/Jun/19

T h a n k y o u v e r y m u c h

Facebook Tweet Pin