Menu Close

Find-the-roots-of-the-equation-x-2-x-1-1-x-2-x-1-10-3-

Tinku Tara 0 Comments
Pin




Question Number 149766 by mathdanisur last updated on 07/Aug/21
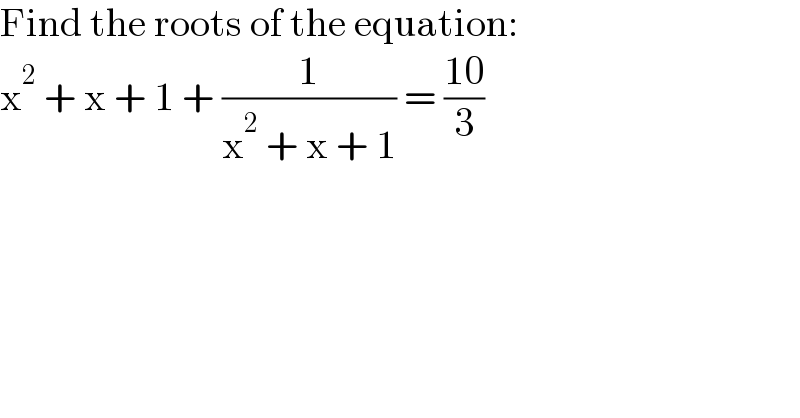
Find the roots of the equation: x^2 + x + 1 + (1/(x^2 + x + 1)) = ((10)/3)
$$\mathrm{Find}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}: \\ $$$$\mathrm{x}^{\mathrm{2}} \:+\:\mathrm{x}\:+\:\mathrm{1}\:+\:\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} \:+\:\mathrm{x}\:+\:\mathrm{1}}\:=\:\frac{\mathrm{10}}{\mathrm{3}} \\ $$
Commented by amin96 last updated on 07/Aug/21
x^2 +x+1=y ⇒ y+(1/y)=((10)/3) y^2 +1=((10y)/3) ⇒ 3y^2 −10y+3=0 Δ=100−36=64 y=((10+8)/6)=3 x^2 +x+1=3 ⇒ x^2 +x−2=0 Δ=9 { ((x_1 =((−1+3)/2)=1)),((x_2 =((−1−3)/2)=−2)) :}
$${x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}=\mathrm{y}\:\:\:\:\Rightarrow\:\:{y}+\frac{\mathrm{1}}{{y}}=\frac{\mathrm{10}}{\mathrm{3}} \\ $$$${y}^{\mathrm{2}} +\mathrm{1}=\frac{\mathrm{10}{y}}{\mathrm{3}}\:\:\Rightarrow\:\:\mathrm{3}{y}^{\mathrm{2}} −\mathrm{10}{y}+\mathrm{3}=\mathrm{0} \\ $$$$\Delta=\mathrm{100}−\mathrm{36}=\mathrm{64}\:\:\:\:{y}=\frac{\mathrm{10}+\mathrm{8}}{\mathrm{6}}=\mathrm{3} \\ $$$${x}^{\mathrm{2}} +{x}+\mathrm{1}=\mathrm{3}\:\:\Rightarrow\:\:{x}^{\mathrm{2}} +{x}−\mathrm{2}=\mathrm{0}\:\:\:\Delta=\mathrm{9} \\ $$$$\begin{cases}{{x}_{\mathrm{1}} =\frac{−\mathrm{1}+\mathrm{3}}{\mathrm{2}}=\mathrm{1}}\\{{x}_{\mathrm{2}} =\frac{−\mathrm{1}−\mathrm{3}}{\mathrm{2}}=−\mathrm{2}}\end{cases}\:\: \\ $$
Commented by mathdanisur last updated on 07/Aug/21
Thankyou Ser
$$\mathrm{Thankyou}\:\mathrm{Ser} \\ $$
Answered by Canebulok last updated on 07/Aug/21
Solution: let: ⇒ x^2 +x+1 = k ∵ ⇒ k+(1/k) = ((10)/3) ⇒ k^2 +1 = ((10)/3)∙k ⇒ k^2 −((10)/3)∙k+1 = 0 By using quadratic formula: ⇒ k = (((((10)/3))±(√((−((10)/3))^2 −4)))/2) = (((((10)/3))±(√((64)/9)))/2) ∵ ⇒ k = 3 ; k = (1/3) By substituting back: (1st solutions) ⇒ x^2 +x+1 = 3 ⇒ x^2 +x−2 = 0 ⇒ (x+2)(x−1) = 0 ⇒ x_1 = −2 ; x_2 = 1 (2nd solutions) ⇒ x^2 +x+1 = (1/3) ⇒ x^2 +x+(2/3) = 0 By quadratic formula: ⇒ x = ((−1±(√(1^2 −4((2/3)))))/2) x_(3,4) = ((−1±i(√(5/3)))/2) Solution by: Kevin
$$\: \\ $$$$\boldsymbol{{Solution}}: \\ $$$${let}: \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} +{x}+\mathrm{1}\:=\:{k} \\ $$$$\because \\ $$$$\Rightarrow\:{k}+\frac{\mathrm{1}}{{k}}\:=\:\frac{\mathrm{10}}{\mathrm{3}} \\ $$$$\Rightarrow\:{k}^{\mathrm{2}} +\mathrm{1}\:=\:\frac{\mathrm{10}}{\mathrm{3}}\centerdot{k} \\ $$$$\Rightarrow\:{k}^{\mathrm{2}} −\frac{\mathrm{10}}{\mathrm{3}}\centerdot{k}+\mathrm{1}\:=\:\mathrm{0} \\ $$$${By}\:{using}\:{quadratic}\:{formula}: \\ $$$$\Rightarrow\:{k}\:=\:\frac{\left(\frac{\mathrm{10}}{\mathrm{3}}\right)\pm\sqrt{\left(−\frac{\mathrm{10}}{\mathrm{3}}\right)^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}} \\ $$$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:=\:\frac{\left(\frac{\mathrm{10}}{\mathrm{3}}\right)\pm\sqrt{\frac{\mathrm{64}}{\mathrm{9}}}}{\mathrm{2}} \\ $$$$\because \\ $$$$\Rightarrow\:{k}\:=\:\mathrm{3}\:\:\:;\:\:\:{k}\:=\:\frac{\mathrm{1}}{\mathrm{3}} \\ $$$${By}\:{substituting}\:{back}: \\ $$$$\left(\mathrm{1}{st}\:{solutions}\right) \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} +{x}+\mathrm{1}\:=\:\mathrm{3} \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} +{x}−\mathrm{2}\:=\:\mathrm{0} \\ $$$$\Rightarrow\:\left({x}+\mathrm{2}\right)\left({x}−\mathrm{1}\right)\:=\:\mathrm{0} \\ $$$$\Rightarrow\:{x}_{\mathrm{1}} \:=\:−\mathrm{2}\:\:\:;\:\:{x}_{\mathrm{2}} \:=\:\mathrm{1} \\ $$$$\: \\ $$$$\left(\mathrm{2}{nd}\:{solutions}\right) \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} +{x}+\mathrm{1}\:=\:\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} +{x}+\frac{\mathrm{2}}{\mathrm{3}}\:=\:\mathrm{0} \\ $$$${By}\:{quadratic}\:{formula}: \\ $$$$\Rightarrow\:{x}\:=\:\frac{−\mathrm{1}\pm\sqrt{\mathrm{1}^{\mathrm{2}} −\mathrm{4}\left(\frac{\mathrm{2}}{\mathrm{3}}\right)}}{\mathrm{2}} \\ $$$$\: \\ $$$$\:\:\:\:\:\:{x}_{\mathrm{3},\mathrm{4}} \:\:=\:\frac{−\mathrm{1}\pm{i}\sqrt{\frac{\mathrm{5}}{\mathrm{3}}}}{\mathrm{2}} \\ $$$$\: \\ $$$$\boldsymbol{{Solution}}\:\boldsymbol{{by}}:\:{Kevin} \\ $$
Commented by mathdanisur last updated on 07/Aug/21
Thankyou Ser
$$\mathrm{Thankyou}\:\boldsymbol{\mathrm{S}}\mathrm{er} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *