find-the-slope-for-the-curve-r-3-sin-2-at-pi-4-

Question Number 87130 by jagoll last updated on 03/Apr/20

find the slope for the curve

r = 3 \sin 2 θ at θ = \frac{π}{4} ?

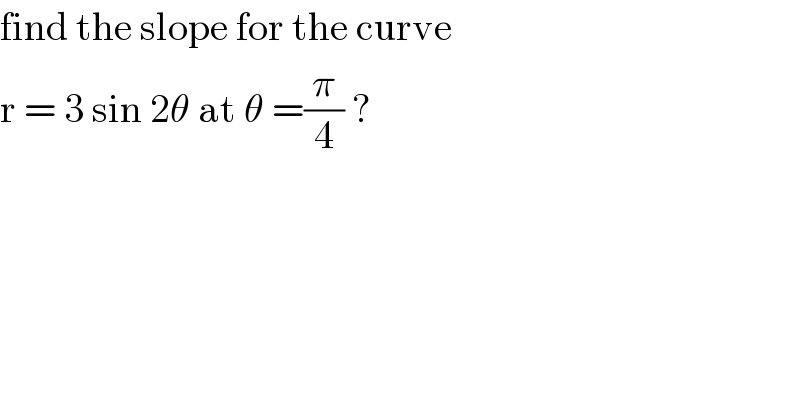
Commented by mr W last updated on 03/Apr/20

y = r \sin θ = 3 \sin 2 θ \sin θ

x = r \cos θ = 3 \sin 2 θ \cos θ

\frac{d y}{d x} = \frac{d y}{d θ} \times \frac{1}{\frac{d x}{d θ}} = \frac{\sin 2 θ \cos θ + 2 \cos 2 θ \sin θ}{- \sin 2 θ \sin θ + 2 \cos 2 θ \cos θ}

= \frac{1 \times \frac{\sqrt{2}}{2} + 2 \times 0 \times \frac{\sqrt{2}}{2}}{- 1 \times \frac{\sqrt{2}}{2} + 2 \times 0 \times \frac{\sqrt{2}}{2}} = - 1

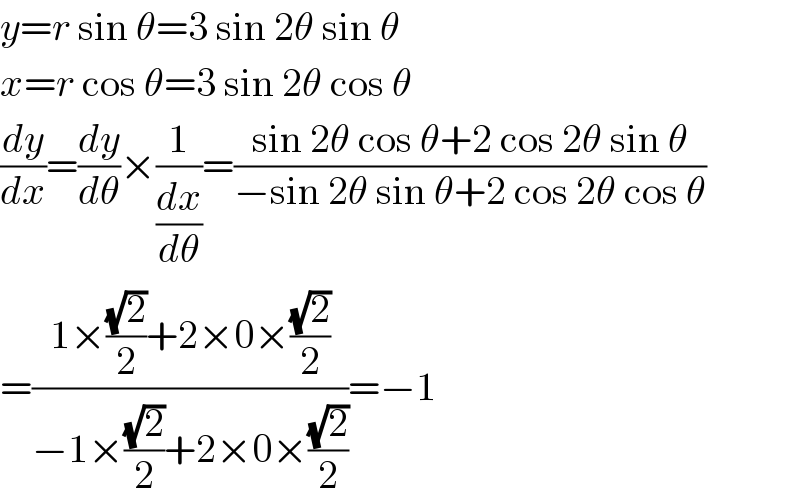
Commented by mr W last updated on 03/Apr/20

Commented by john santu last updated on 03/Apr/20

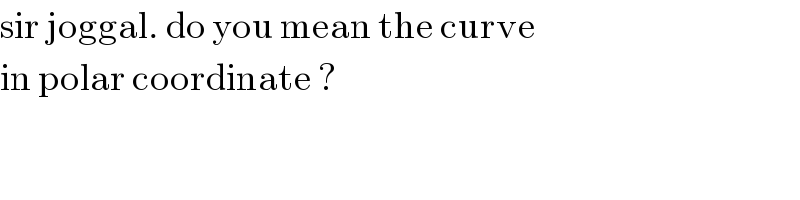
sir joggal . do you mean the curve

in polar coordinate ?

Commented by jagoll last updated on 03/Apr/20

yes mister john

Commented by jagoll last updated on 03/Apr/20

thank you mr w

Facebook Tweet Pin