find-the-sum-of-the-series-whose-nth-term-is-2n-1-n-n-1-n-2-i-have-a-problem-with-this-and-i-need-help-please-

Question Number 103623 by Lordose last updated on 16/Jul/20

find the sum of the series whose nth

term is \frac{2 n - 1}{n (n + 1) (n + 2} .

i have a problem with this and i need

help please

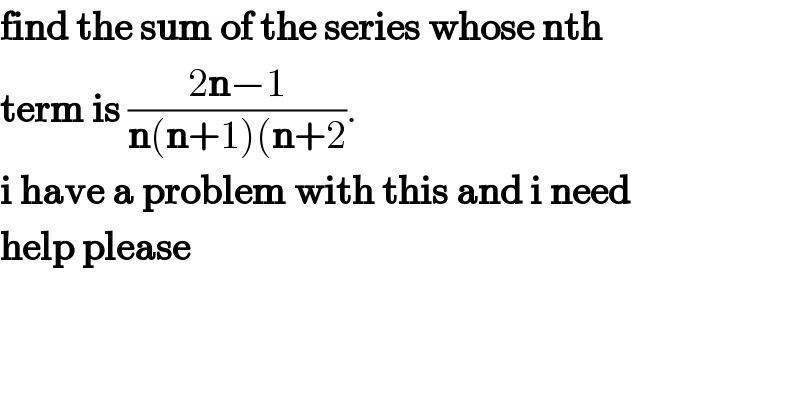
Answered by OlafThorendsen last updated on 16/Jul/20

find the sum of the series whose nth

term is \frac{2 n - 1}{n (n + 1) (n + 2} .

i have a problem with this and i need

help please

u_{n} = \frac{2 n - 1}{n (n + 1) (n + 2)}

u_{n} = - \frac{1}{2} . \frac{1}{n} + \frac{3}{n + 1} - \frac{5}{2} . \frac{1}{n + 2}

\underset{n = 1}{\sum^{p}} u_{n} = - \frac{1}{2} \underset{n = 1}{\sum^{p}} \frac{1}{n} + 3 \underset{n = 1}{\sum^{p}} \frac{1}{n + 1} - \frac{5}{2} \underset{n = 1}{\sum^{p}} \frac{1}{n + 2}

\underset{n = 1}{\sum^{p}} u_{n} = - \frac{1}{2} \underset{n = 1}{\sum^{p}} \frac{1}{n} + 3 \underset{n = 2}{\sum^{p + 1}} \frac{1}{n} - \frac{5}{2} \underset{n = 3}{\sum^{p + 2}} \frac{1}{n}

\underset{n = 1}{\sum^{p}} u_{n} = - \frac{1}{2} (1 + \frac{1}{2}) + 3 (\frac{1}{2}) + 3 (\frac{1}{p + 1}) - \frac{5}{2} (\frac{1}{p + 1} + \frac{1}{p + 2})

\underset{n = 1}{\sum^{p}} u_{n} = \frac{3}{4} + \frac{1}{2} (\frac{1}{p + 1}) - \frac{5}{2} (\frac{1}{p + 2})

\underset{n = 1}{\sum^{p}} u_{n} = \frac{3}{4} - \frac{2 p + \frac{3}{2}}{(p + 1) (p + 2)}

and \underset{n = 1}{\sum^{\infty}} u_{n} = \frac{3}{4}

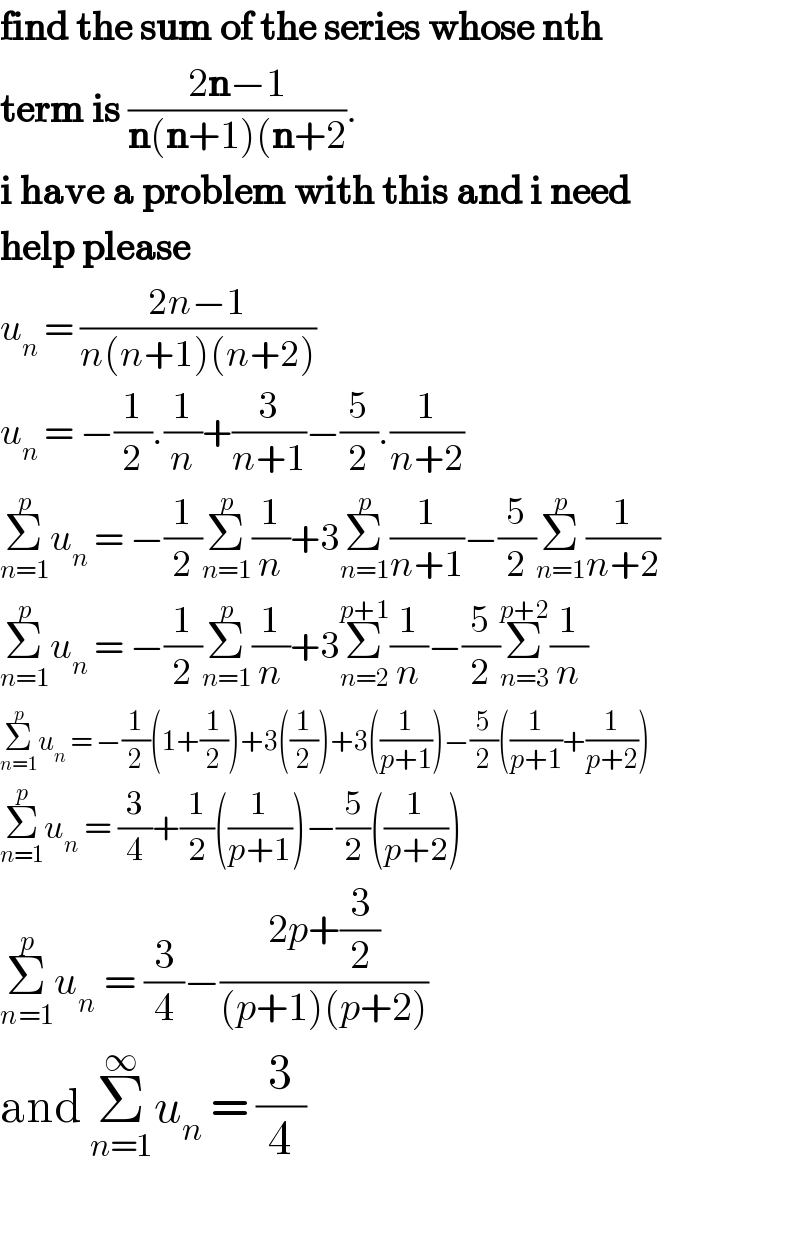
Commented by Lordose last updated on 16/Jul/20

sir i {don}^{'} t understand the 4 th line

can you explain pls

Answered by bobhans last updated on 16/Jul/20

\underset{n = 1}{\sum^{\infty}} \frac{2 n - 1}{n (n + 1) (n + 2)}

= \underset{n = 1}{\sum^{\infty}} \frac{3}{n + 1} - \underset{n = 1}{\sum^{\infty}} \frac{1}{2 n} - \underset{n = 1}{\sum^{\infty}} \frac{5}{2 (n + 2)}

= \underset{n = 2}{\sum^{\infty}} \frac{3}{n} - \frac{1}{2} \underset{n = 1}{\sum^{\infty}} \frac{1}{n} - \frac{5}{2} \underset{n = 3}{\sum^{\infty}} \frac{1}{n}

= \underset{n = 1}{\sum^{\infty}} \frac{3}{n} - 3 - \frac{1}{2} \underset{n = 1}{\sum^{\infty}} \frac{1}{n} - \frac{5}{2} {\underset{n = 1}{\sum^{\infty}} \frac{1}{n} - 1 - \frac{1}{2}}

= - 3 + \frac{15}{4} + (3 - \frac{1}{2} - \frac{5}{2}) \underset{n = 1}{\sum^{\infty}} \frac{1}{n}

= \frac{3}{4} + 0 \lim_{k \to \infty} \underset{n = 1}{\sum^{k}} \frac{1}{n} = \frac{3}{4} + 0 = \frac{3}{4}

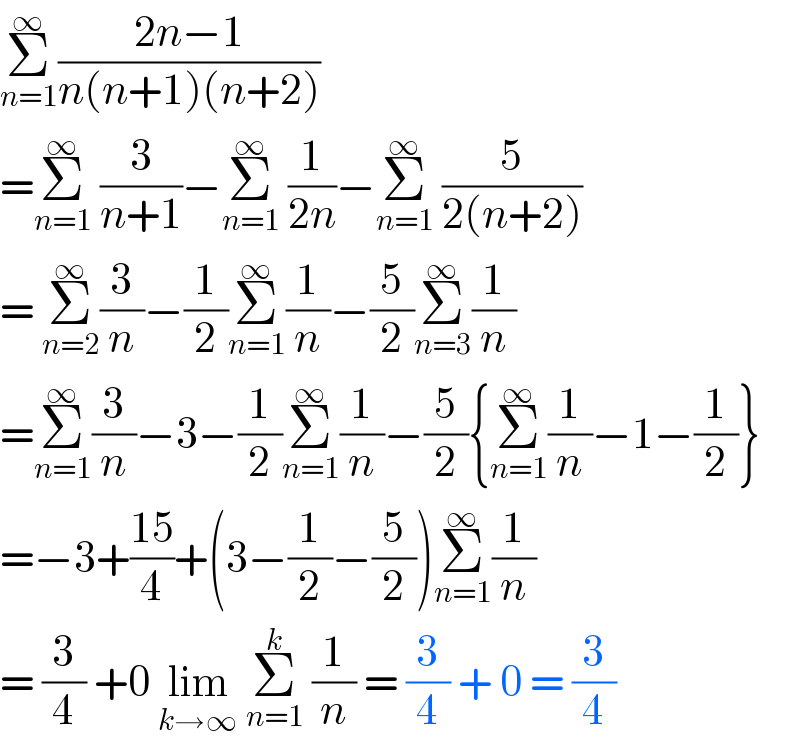
Commented by Lordose last updated on 16/Jul/20

can anyone make me understand pls

Commented by bobhans last updated on 16/Jul/20

w h a t p a r t y o u {d o n}^{'} t u n d e r s t a n d ?

Facebook Tweet Pin