Find-the-sum-to-n-term-1-2-3-4-5-6-7-8-9-

Question Number 147878 by Tawa11 last updated on 24/Jul/21

Find the sum to n term : 1.2.3 + 4.5.6 + 7.8.9 + \dots

Answered by Olaf_Thorendsen last updated on 24/Jul/21

S = \underset{k = 1}{\sum^{n}} (3 k - 2) (3 k - 1) (3 k)

S = 3 \underset{k = 1}{\sum^{n}} (9 k^{3} - 9 k^{2} + 2 k)

S = 3 (9 \frac{n^{2} {(n + 1)}^{2}}{4} - 9 \frac{n (n + 1) (2 n + 1)}{6} + 2 \frac{n (n + 1)}{2})

S = 3 n (n + 1) (9 \frac{n (n + 1)}{4} - 9 \frac{(2 n + 1)}{6} + 1)

S = \frac{3}{4} n (n + 1) (9 n^{2} - 3 n - 2)

S = \frac{3}{4} n (n + 1) (3 n - 2) (3 n + 1)

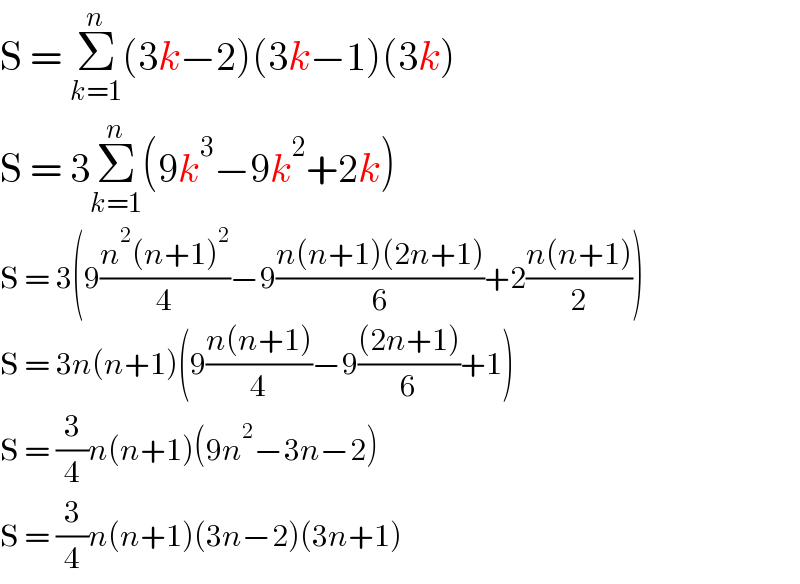
Commented by puissant last updated on 24/Jul/21

monsieur au debut c^{'} est k qui varie

mais vous avez mis n . . on va supposer

que c^{'} est une erreur . .

Commented by Tawa11 last updated on 24/Jul/21

God bless you sir . I appreciste .

Commented by Olaf_Thorendsen last updated on 24/Jul/21

bien vu .