Menu Close

find-the-value-of-x-1-x-4-x-2-1-3-dx-

Tinku Tara 0 Comments
Pin




Question Number 62806 by mathmax by abdo last updated on 25/Jun/19
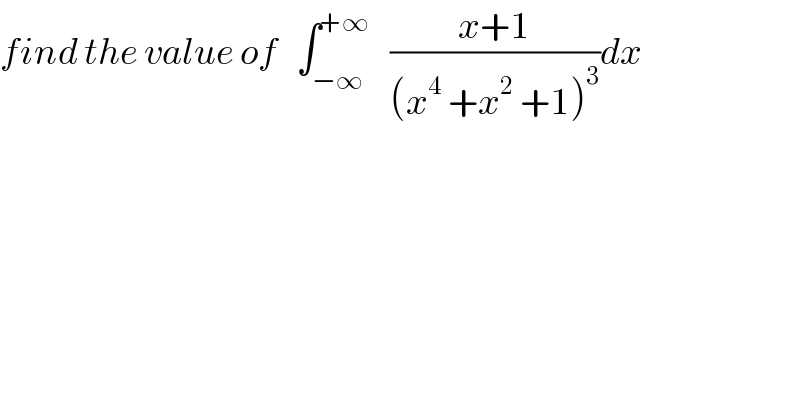
find the value of ∫_(−∞) ^(+∞) ((x+1)/((x^4 +x^2 +1)^3 ))dx
$${find}\:{the}\:{value}\:{of}\:\:\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{x}+\mathrm{1}}{\left({x}^{\mathrm{4}} \:+{x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{3}} }{dx} \\ $$
Commented by mathmax by abdo last updated on 27/Jun/19
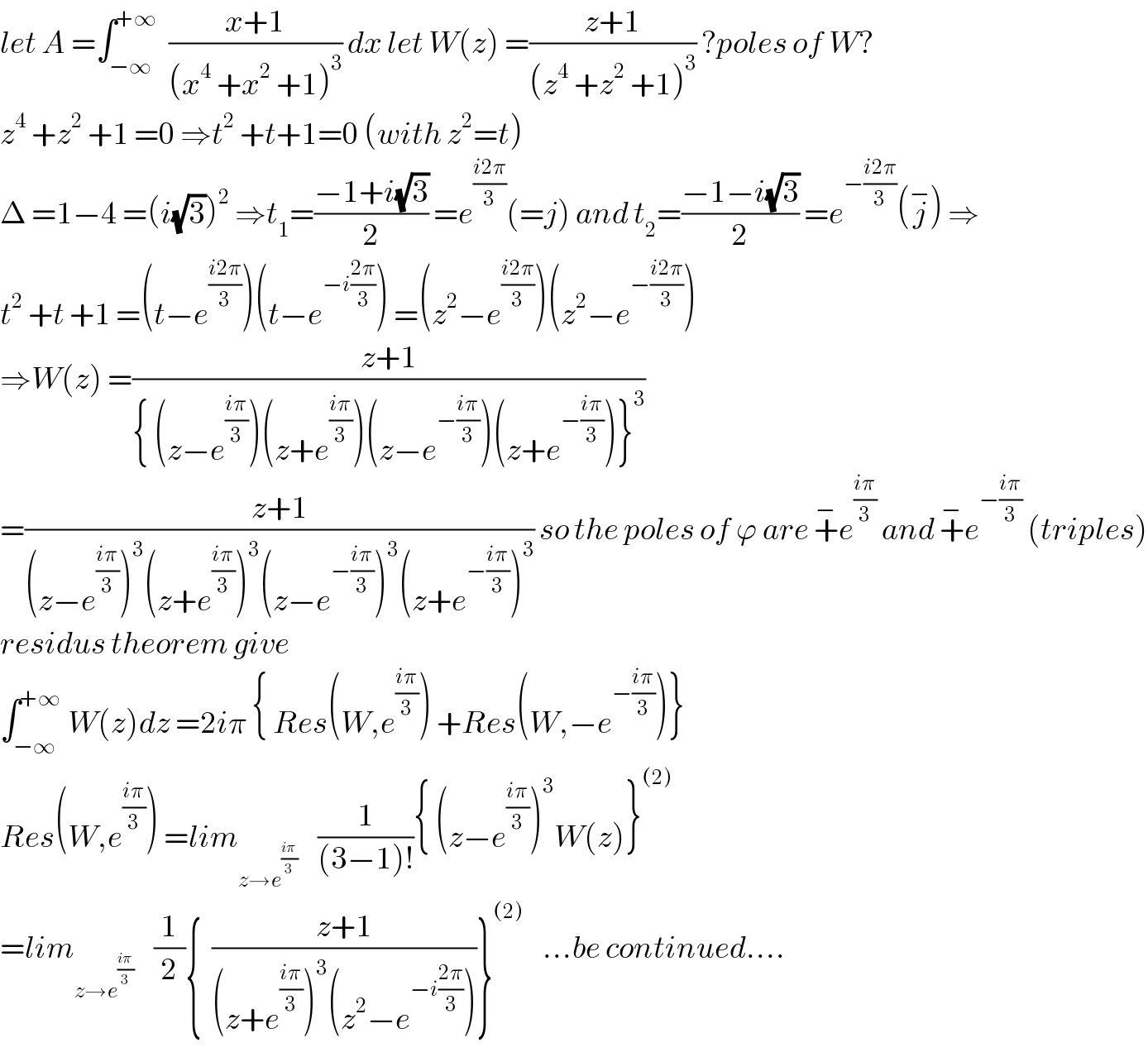
let A =∫_(−∞) ^(+∞) ((x+1)/((x^4 +x^2 +1)^3 )) dx let W(z) =((z+1)/((z^4 +z^2 +1)^3 )) ?poles of W? z^4 +z^2 +1 =0 ⇒t^2 +t+1=0 (with z^2 =t) Δ =1−4 =(i(√3))^2 ⇒t_1 =((−1+i(√3))/2) =e^((i2π)/3) (=j) and t_2 =((−1−i(√3))/2) =e^(−((i2π)/3)) (j^− ) ⇒ t^2 +t +1 =(t−e^((i2π)/3) )(t−e^(−i((2π)/3)) ) =(z^2 −e^((i2π)/3) )(z^2 −e^(−((i2π)/3)) ) ⇒W(z) =((z+1)/({ (z−e^((iπ)/3) )(z+e^((iπ)/3) )(z−e^(−((iπ)/3)) )(z+e^(−((iπ)/3)) )}^3 )) =((z+1)/((z−e^((iπ)/3) )^3 (z+e^((iπ)/3) )^3 (z−e^(−((iπ)/3)) )^3 (z+e^(−((iπ)/3)) )^3 )) so the poles of ϕ are +^− e^((iπ)/3) and +^− e^(−((iπ)/3)) (triples) residus theorem give ∫_(−∞) ^(+∞) W(z)dz =2iπ { Res(W,e^((iπ)/3) ) +Res(W,−e^(−((iπ)/3)) )} Res(W,e^((iπ)/3) ) =lim_(z→e^((iπ)/3) ) (1/((3−1)!)){ (z−e^((iπ)/3) )^3 W(z)}^((2)) =lim_(z→e^((iπ)/3) ) (1/2){ ((z+1)/((z+e^((iπ)/3) )^3 (z^2 −e^(−i((2π)/3)) )))}^((2)) ...be continued....
$${let}\:{A}\:=\int_{−\infty} ^{+\infty} \:\:\frac{{x}+\mathrm{1}}{\left({x}^{\mathrm{4}} \:+{x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{3}} }\:{dx}\:{let}\:{W}\left({z}\right)\:=\frac{{z}+\mathrm{1}}{\left({z}^{\mathrm{4}} \:+{z}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{3}} }\:?{poles}\:{of}\:{W}? \\ $$$${z}^{\mathrm{4}} \:+{z}^{\mathrm{2}} \:+\mathrm{1}\:=\mathrm{0}\:\Rightarrow{t}^{\mathrm{2}} \:+{t}+\mathrm{1}=\mathrm{0}\:\left({with}\:{z}^{\mathrm{2}} ={t}\right) \\ $$$$\Delta\:=\mathrm{1}−\mathrm{4}\:=\left({i}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} \:\Rightarrow{t}_{\mathrm{1}} =\frac{−\mathrm{1}+{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:={e}^{\frac{{i}\mathrm{2}\pi}{\mathrm{3}}} \left(={j}\right)\:{and}\:{t}_{\mathrm{2}} =\frac{−\mathrm{1}−{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:={e}^{−\frac{{i}\mathrm{2}\pi}{\mathrm{3}}} \left(\overset{−} {{j}}\right)\:\Rightarrow \\ $$$${t}^{\mathrm{2}} \:+{t}\:+\mathrm{1}\:=\left({t}−{e}^{\frac{{i}\mathrm{2}\pi}{\mathrm{3}}} \right)\left({t}−{e}^{−{i}\frac{\mathrm{2}\pi}{\mathrm{3}}} \right)\:=\left({z}^{\mathrm{2}} −{e}^{\frac{{i}\mathrm{2}\pi}{\mathrm{3}}} \right)\left({z}^{\mathrm{2}} −{e}^{−\frac{{i}\mathrm{2}\pi}{\mathrm{3}}} \right) \\ $$$$\Rightarrow{W}\left({z}\right)\:=\frac{{z}+\mathrm{1}}{\left\{\:\left({z}−{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)\left({z}+{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)\left({z}−{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)\left({z}+{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)\right\}^{\mathrm{3}} } \\ $$$$=\frac{{z}+\mathrm{1}}{\left({z}−{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} \left({z}+{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} \left({z}−{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} \left({z}+{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} }\:{so}\:{the}\:{poles}\:{of}\:\varphi\:{are}\:\overset{−} {+}{e}^{\frac{{i}\pi}{\mathrm{3}}} \:{and}\:\overset{−} {+}{e}^{−\frac{{i}\pi}{\mathrm{3}}} \:\left({triples}\right) \\ $$$${residus}\:{theorem}\:{give}\: \\ $$$$\int_{−\infty} ^{+\infty} \:{W}\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:\left\{\:{Res}\left({W},{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)\:+{Res}\left({W},−{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)\right\} \\ $$$${Res}\left({W},{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)\:={lim}_{{z}\rightarrow{e}^{\frac{{i}\pi}{\mathrm{3}}} } \:\:\:\:\frac{\mathrm{1}}{\left(\mathrm{3}−\mathrm{1}\right)!}\left\{\:\left({z}−{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} {W}\left({z}\right)\right\}^{\left(\mathrm{2}\right)} \\ $$$$={lim}_{{z}\rightarrow{e}^{\frac{{i}\pi}{\mathrm{3}}} } \:\:\:\:\frac{\mathrm{1}}{\mathrm{2}}\left\{\:\:\frac{{z}+\mathrm{1}}{\left({z}+{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} \left({z}^{\mathrm{2}} −{e}^{−{i}\frac{\mathrm{2}\pi}{\mathrm{3}}} \right)}\right\}^{\left(\mathrm{2}\right)} \:\:\:\:…{be}\:{continued}…. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *