find-x-2-2-findx-

Question Number 96241 by joki last updated on 30/May/20

$$\mathrm{find}\:\mathrm{x}^{\mathrm{2}} =\mathrm{2}^{×} \:\:\:\mathrm{findx}? \\ $$

Answered by 1549442205 last updated on 31/May/20

It is easy to see that x∈{0,1} don′t satisfy and x∈{2,4} satisfying the given equation We prove that 2^x >x^2 for any x ≥5 Indeed,consider the function f(x)= 2^x −x^2 .We have f ′ (x)=2^x ln2−2x, f ′′(x)=2^x ln^2 2−2>32ln^2 2−2>0 Hence,f ′(x) is increasing function on ]5;+∞)⇒f ′(x)>f ′(5)=32ln2−10>0 This show that the function f(x)=2^x −x^2 is increasing on [5;+∞)⇒f(x)>f(5)=2^5 −25>0 ⇒2^x −x^2 >0⇒2^x >x^2 for every x≥5. Thus,the numbers x satisfy the given equation are x∈{2;4}

$$\mathrm{It}\:\mathrm{is}\:\mathrm{easy}\:\mathrm{to}\:\mathrm{see}\:\mathrm{that}\:\mathrm{x}\in\left\{\mathrm{0},\mathrm{1}\right\}\:\mathrm{don}'\mathrm{t}\:\mathrm{satisfy} \\ $$$$\mathrm{and}\:\mathrm{x}\in\left\{\mathrm{2},\mathrm{4}\right\}\:\mathrm{satisfying}\:\mathrm{the}\:\mathrm{given}\:\mathrm{equation} \\ $$$$\mathrm{We}\:\mathrm{prove}\:\mathrm{that}\:\mathrm{2}^{\mathrm{x}} >\mathrm{x}^{\mathrm{2}} \:\mathrm{for}\:\mathrm{any}\:\mathrm{x}\:\geqslant\mathrm{5} \\ $$$$\mathrm{Indeed},\mathrm{consider}\:\mathrm{the}\:\mathrm{function}\:\mathrm{f}\left(\mathrm{x}\right)= \\ $$$$\mathrm{2}^{\mathrm{x}} −\mathrm{x}^{\mathrm{2}} .\mathrm{We}\:\mathrm{have}\:\mathrm{f}\:'\:\left(\mathrm{x}\right)=\mathrm{2}^{\mathrm{x}} \mathrm{ln2}−\mathrm{2x}, \\ $$$$\mathrm{f}\:''\left(\mathrm{x}\right)=\mathrm{2}^{\mathrm{x}} \mathrm{ln}^{\mathrm{2}} \mathrm{2}−\mathrm{2}>\mathrm{32ln}^{\mathrm{2}} \mathrm{2}−\mathrm{2}>\mathrm{0} \\ $$$$\mathrm{Hence},\mathrm{f}\:'\left(\mathrm{x}\right)\:\mathrm{is}\:\mathrm{increasing}\:\mathrm{function}\:\mathrm{on} \\ $$$$\left.\right]\left.\mathrm{5};+\infty\right)\Rightarrow\mathrm{f}\:'\left(\mathrm{x}\right)>\mathrm{f}\:'\left(\mathrm{5}\right)=\mathrm{32ln2}−\mathrm{10}>\mathrm{0} \\ $$$$\mathrm{This}\:\mathrm{show}\:\mathrm{that} \\ $$$$\mathrm{the}\:\mathrm{function}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{2}^{\mathrm{x}} −\mathrm{x}^{\mathrm{2}} \:\mathrm{is}\:\mathrm{increasing} \\ $$$$\mathrm{on}\:\left[\mathrm{5};+\infty\right)\Rightarrow\mathrm{f}\left(\mathrm{x}\right)>\mathrm{f}\left(\mathrm{5}\right)=\mathrm{2}^{\mathrm{5}} −\mathrm{25}>\mathrm{0} \\ $$$$\Rightarrow\mathrm{2}^{\mathrm{x}} −\mathrm{x}^{\mathrm{2}} >\mathrm{0}\Rightarrow\mathrm{2}^{\mathrm{x}} >\mathrm{x}^{\mathrm{2}} \:\mathrm{for}\:\mathrm{every}\:\mathrm{x}\geqslant\mathrm{5}. \\ $$$$\mathrm{Thus},\mathrm{the}\:\mathrm{numbers}\:\mathrm{x}\:\mathrm{satisfy}\:\mathrm{the}\:\mathrm{given} \\ $$$$\mathrm{equation}\:\mathrm{are}\:\mathrm{x}\in\left\{\mathrm{2};\mathrm{4}\right\} \\ $$

Answered by mr W last updated on 31/May/20

x^2 =2^x ⇒x=±2^(x/2) =±e^((x/2)ln 2) ⇒xe^(−(x/2)ln 2) =±1 ⇒(−(x/2)ln 2)e^(−(x/2)ln 2) =±((ln 2)/2) ⇒−(x/2)ln 2=W(±((ln 2)/2)) with W()=Lambert W function ⇒x=−((2/(ln 2)))W(±((ln 2)/2))= { ((−(2/(ln 2))W(((ln 2)/2))≈−(2/(ln 2))×0.26570574=−0.766665)),((−(2/(ln 2))W(−((ln 2)/2))= { ((−(2/(ln 2))×(−0.693147)=2)),((−(2/(ln 2))×(−1.386294)=4)) :})) :} that means there are three roots.

$${x}^{\mathrm{2}} =\mathrm{2}^{{x}} \\ $$$$\Rightarrow{x}=\pm\mathrm{2}^{\frac{{x}}{\mathrm{2}}} =\pm{e}^{\frac{{x}}{\mathrm{2}}\mathrm{ln}\:\mathrm{2}} \\ $$$$\Rightarrow{xe}^{−\frac{{x}}{\mathrm{2}}\mathrm{ln}\:\mathrm{2}} =\pm\mathrm{1} \\ $$$$\Rightarrow\left(−\frac{{x}}{\mathrm{2}}\mathrm{ln}\:\mathrm{2}\right){e}^{−\frac{{x}}{\mathrm{2}}\mathrm{ln}\:\mathrm{2}} =\pm\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}} \\ $$$$\Rightarrow−\frac{{x}}{\mathrm{2}}\mathrm{ln}\:\mathrm{2}=\mathbb{W}\left(\pm\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}\right)\:\:\:\:{with}\:\mathbb{W}\left(\right)={Lambert}\:{W}\:{function} \\ $$$$\Rightarrow{x}=−\left(\frac{\mathrm{2}}{\mathrm{ln}\:\mathrm{2}}\right)\mathbb{W}\left(\pm\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}\right)=\begin{cases}{−\frac{\mathrm{2}}{\mathrm{ln}\:\mathrm{2}}\mathbb{W}\left(\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}\right)\approx−\frac{\mathrm{2}}{\mathrm{ln}\:\mathrm{2}}×\mathrm{0}.\mathrm{26570574}=−\mathrm{0}.\mathrm{766665}}\\{−\frac{\mathrm{2}}{\mathrm{ln}\:\mathrm{2}}\mathbb{W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}\right)=\begin{cases}{−\frac{\mathrm{2}}{\mathrm{ln}\:\mathrm{2}}×\left(−\mathrm{0}.\mathrm{693147}\right)=\mathrm{2}}\\{−\frac{\mathrm{2}}{\mathrm{ln}\:\mathrm{2}}×\left(−\mathrm{1}.\mathrm{386294}\right)=\mathrm{4}}\end{cases}}\end{cases} \\ $$$${that}\:{means}\:{there}\:{are}\:\boldsymbol{{three}}\:{roots}. \\ $$

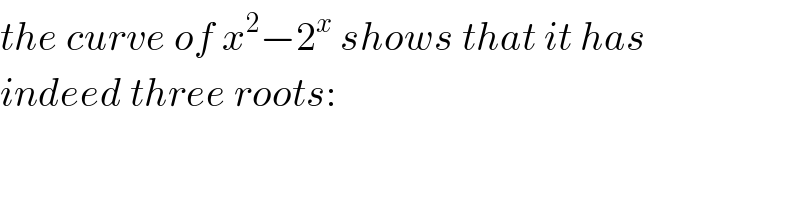
Commented by mr W last updated on 31/May/20