Find-x-x-x-2x-

Question Number 60946 by Tawa1 last updated on 27/May/19

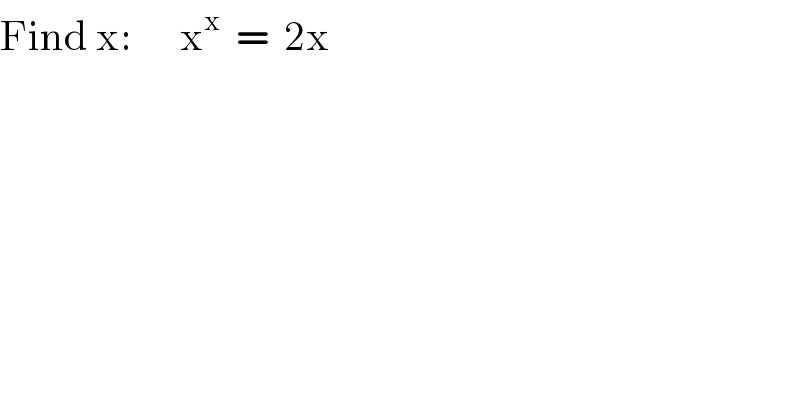
Find x : x^{x} = 2 x

Commented by Prithwish sen last updated on 27/May/19

x = 2

Commented by mr W last updated on 28/May/19

t h r e e c a s e s f o r e q n . x^{x} = a x :

c a s e 1 : a < 1 \Rightarrow n o s o l u t i o n

c a s e 2 : a = 1 \Rightarrow o n e s o l u t i o n x = 1

c a s e 3 : a > 1 \Rightarrow t w o s o l u t i o n s

Commented by Tawa1 last updated on 28/May/19

Oh, that mean Lambert cannot solve it sir

Commented by mr W last updated on 28/May/19

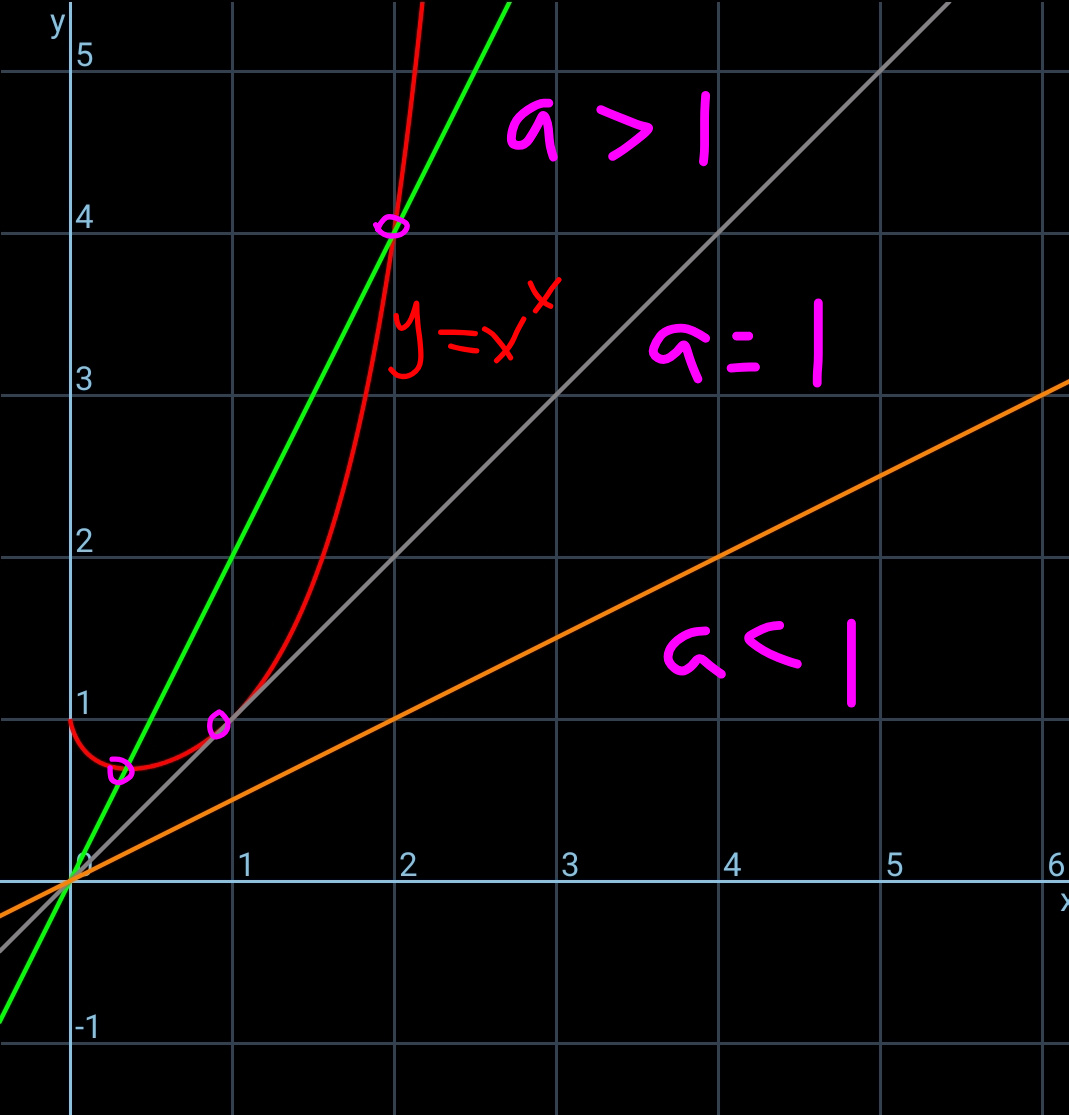
Answered by meme last updated on 27/May/19

\Rightarrow e^{x l n (x)} = e^{2 l n (x)}

\Rightarrow x l n (x) = 2 l n (x)

\Rightarrow x = 2

Commented by mr W last updated on 27/May/19

\Rightarrow e^{x l n (x)} = e^{l n (2 x)} \neq e^{2 \ln (x)}

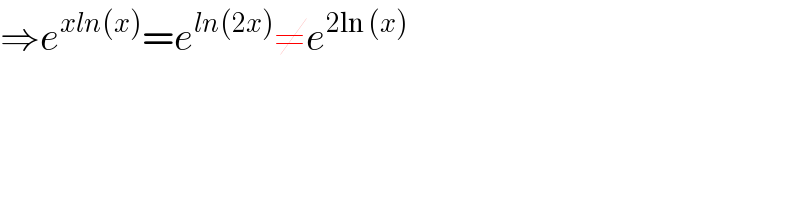
Commented by Tawa1 last updated on 28/May/19

Sir mrW . Can lambert work ?

Commented by mr W last updated on 28/May/19

n o, i^{'} m a f r a i d .

Commented by kaivan.ahmadi last updated on 28/May/19

e^{x l n x} = e^{l n (2 x)} = e^{l n 2 + l n x} = e^{l n 2} e^{l n x} \Rightarrow

\frac{e^{x l n x}}{e^{l n x}} = e^{l n 2} \Rightarrow e^{(x - 1) l n x} = e^{l n 2} \Rightarrow

(x - 1) l n x = l n 2 \Rightarrow x^{x - 1} = 2^{1} \Rightarrow x = 2

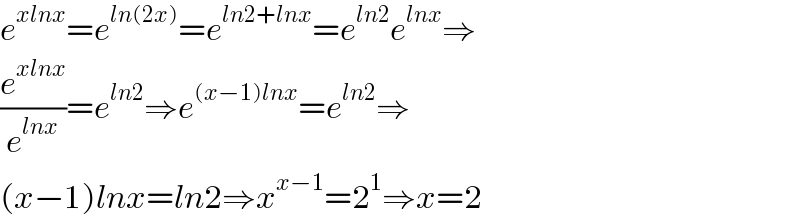
Commented by mr W last updated on 29/May/19

w e c a n g e t x^{x - 1} = 2^{1} d i r e c t l y f r o m

x^{x} = 2 x

\frac{x^{x}}{x} = 2

x^{x - 1} = 2^{1}

b u t x = 2 i s n o t t h e o n l y s o l u t i o n .

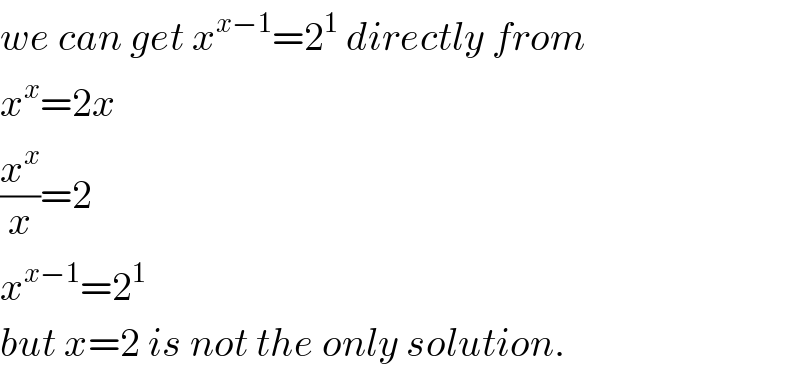
Answered by meme last updated on 27/May/19

\Rightarrow e^{x l n (x)} = e^{2 l n (x)}

\Rightarrow x l n (x) = 2 l n (x)

\Rightarrow x = 2

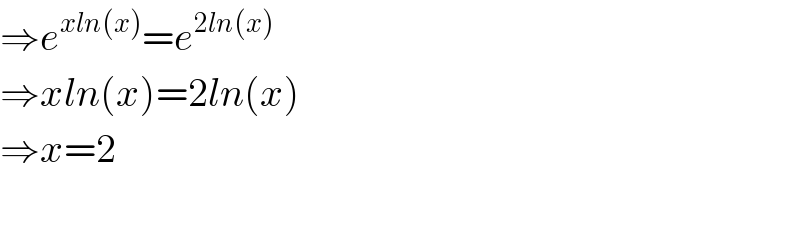
Commented by JDamian last updated on 27/May/19

e^{2 l n (x)} \neq 2 x

e^{2 l n (x)} = x^{2}

Answered by MJS last updated on 27/May/19

f (x) = x^{x} - 2 x

f (0) = 1 [because \lim_{x \to 0} f (x) = 1]

f (1) = - 1

f (2) = 0

f (3) = 21

\Rightarrow there must be a zero in [0; 1], approximating

we find

x \approx . 346323

and of course x = 2 is the other solution

Commented by Tawa1 last updated on 28/May/19

God bless you sir

Facebook Tweet Pin