For-any-Real-numbers-x-y-and-z-if-x-y-z-2-then-prove-that-xyz-8-1-x-1-y-1-z-

Question Number 109595 by nimnim last updated on 24/Aug/20

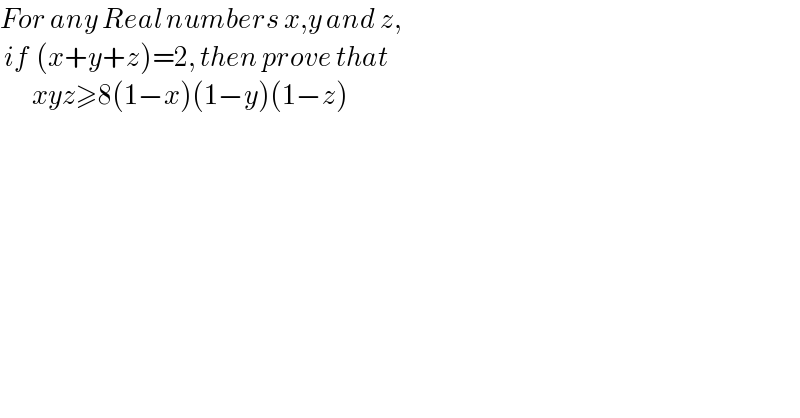
F o r a n y R e a l n u m b e r s x, y a n d z,

i f (x + y + z) = 2, t h e n p r o v e t h a t

x y z ⩾ 8 (1 - x) (1 - y) (1 - z)

Commented by 1549442205PVT last updated on 25/Aug/20

This inequality is only true for

x, y, z > 0 . Example, for x = - \frac{2}{3}, y = \frac{6}{5}

z = \frac{22}{15} then it is false . Then

xyz - 8 (1 - x) (1 - y) (1 - z) = - \frac{544}{25} < 0

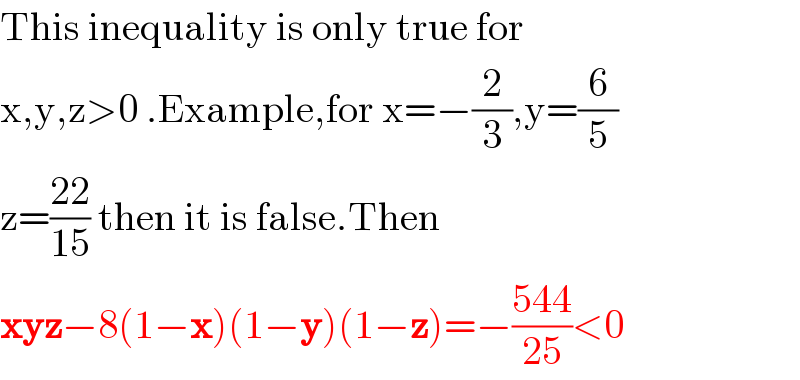
Answered by floor(10²Eta[1]) last updated on 24/Aug/20

\frac{x + y + z}{3} ⩾^{3} \sqrt{xyz} \Rightarrow \frac{2}{3} ⩾^{3} \sqrt{xyz} ∴ \frac{8}{27} ⩾ xyz

\Rightarrow xyz ⩾ 8 (1 - x) (1 - y) (1 - z)

\Leftrightarrow \frac{1}{27} ⩾ (1 - x) (1 - y) (1 - z)

\frac{(1 - x) + (1 - y) + (1 - z)}{3} ⩾^{3} \sqrt{(1 - x) (1 - y) (1 - z)}

\frac{3 - (x + y + z)}{3} ⩾^{3} \sqrt{(1 - x) (1 - y) (1 - z)}

\frac{1}{3} ⩾^{3} \sqrt{(1 - x) (1 - y) (1 - z)}

\frac{1}{27} ⩾ (1 - x) (1 - y) (1 - z)

∴ xyz ⩾ 8 (1 - x) (1 - y) (1 - z), x + y + z = 2

Commented by nimnim last updated on 24/Aug/20

T h a n k y o u s i r . .

Commented by floor(10²Eta[1]) last updated on 25/Aug/20

i just used AM - GM inequality which

is true for all real numbers