for-z-1-1-show-that-tan-arg-z-1-2-2i-z-1-

Question Number 87302 by M±th+et£s last updated on 03/Apr/20

f o r ∣ z - 1 ∣= 1 s h o w t h a t

t a n (\frac{a r g (z - 1)}{2}) - \frac{2 i}{z} = - 1

Commented by MJS last updated on 04/Apr/20

\tan \frac{\arg (z - 1)}{2} \in R

- \frac{2 i}{z} \notin R \forall z = a + b i; a \neq 0

\Rightarrow generally lhs \notin R but rhs = - 1 \in R the

equation is wrong

∣ z - 1 ∣= 1 is a circle :

z = a + b i

∣ a - 1 + b i ∣= 1 \Leftrightarrow \sqrt{{(a - 1)}^{2} + b^{2}} = 1 \Leftrightarrow b = \pm \sqrt{2 a - a^{2}}

\Rightarrow generally z = a + b i; a \neq 0

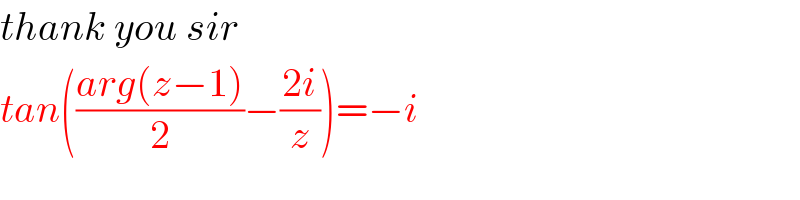
Commented by M±th+et£s last updated on 04/Apr/20

t h a n k y o u s i r

t a n (\frac{a r g (z - 1)}{2} - \frac{2 i}{z}) = - i

Commented by M±th+et£s last updated on 04/Apr/20

z - 1 = e^{i θ}

z = 1 + c o s θ + i s i n θ

z = 2 {c o s}^{2} \frac{θ}{2} + 2 c o s \frac{θ}{2} s i n \frac{θ}{2}

z = 2 c o s \frac{θ}{2} (c o s \frac{θ}{2} + i s i n \frac{θ}{2})

z = 2 c o s \frac{θ}{2} e^{i \frac{θ}{2}}

∴ t a n (\frac{a r g (z - 1)}{2} - \frac{2 i}{z})

= t a n \frac{θ}{2} - \frac{i}{c o s \frac{θ}{2}} e^{- i \frac{θ}{2}}

= t a n \frac{θ}{2} - i \frac{(c o s \frac{θ}{2} - i s i n \frac{θ}{2})}{c o s \frac{θ}{2}}

= \frac{s i n \frac{θ}{2} - i c o s \frac{θ}{2} - s i n \frac{θ}{2}}{c o s \frac{θ}{2}} = - i

Answered by MJS last updated on 04/Apr/20

let Z = z - 1

∣ Z ∣= 1 \Rightarrow Z = \cos θ + i \sin θ

\tan \frac{\arg Z}{2} = - i + \frac{2 i}{Z + 1}

\tan \frac{θ}{2} = - i + \frac{2 i}{1 + \cos θ + i \sin θ}

\tan \frac{θ}{2} = - i + \frac{2 i (1 + \cos θ - i \sin θ)}{{(1 + \cos θ)}^{2} + \sin^{2} θ}

\tan \frac{θ}{2} = - i + \frac{2 \sin θ + 2 (1 + \cos θ) i}{2 + 2 \cos θ}

\tan \frac{θ}{2} = - i + \frac{\sin θ}{1 + \cos θ} + i

\tan \frac{θ}{2} = \frac{\sin θ}{1 + \cos θ} this is a well known formula

true!

Commented by M±th+et£s last updated on 04/Apr/20

t h a n k y o u s i r