general-calculus-i-1-1-4x-n-0-2n-n-x-n-ii-pi-2-n-0-2n-n-4-n-1-2n-1-

Question Number 122704 by mnjuly1970 last updated on 19/Nov/20

\dots g e n e r a l c a l c u l u s \dots

i : \frac{1}{\sqrt{1 - 4 x}} \overset{? ?}{=} \underset{n = 0}{\sum^{\infty}} [(\begin{matrix} 2 n \\ n \end{matrix}) x^{n}]

i i : \frac{π}{2} \overset{?}{=} \underset{n = 0}{\sum^{\infty}} [\frac{(\begin{matrix} 2 n \\ n \end{matrix})}{4^{n}} \frac{1}{(2 n + 1)}]

Answered by Dwaipayan Shikari last updated on 19/Nov/20

\frac{1}{\sqrt{1 - 4 x}} = {(1 - 4 x)}^{- \frac{1}{2}} = 1 + 2 x + \frac{3}{4} 16 x^{2} + \frac{15}{8} 64 x^{3} + \dots

= 1 + 2 x + 12 x^{2} + 120 x^{3} + . .

= 1 + \frac{2!}{1!} x + \frac{4!}{2!} x^{2} + \frac{6!}{3!} x^{3} + . . = \underset{n = 0}{\sum^{\infty}} [(\begin{matrix} 2 n \\ n \end{matrix}) x^{n}]

Answered by Dwaipayan Shikari last updated on 19/Nov/20

{s i n}^{- 1} x = \int \frac{1}{\sqrt{1 - x^{2}}} d x

{s i n}^{- 1} x = \int 1 + \frac{x^{2}}{2} + \frac{3}{4} x^{4} + \frac{15}{8} x^{6} + . .

{s i n}^{- 1} x = x + \frac{x^{3}}{6} + \frac{3 x^{5}}{20} + \frac{15}{56} x^{7} + \dots

{s i n}^{- 1} 1 = 1 + \frac{1}{6} + \frac{3}{20} + \frac{15}{56} + \dots

\frac{π}{2} = 1 + \frac{2!}{4.3} + \frac{4!}{2.16.5} + \frac{6!}{3! 4^{2} . 7} + . .

= \underset{n = 0}{\sum^{\infty}} \frac{(\begin{matrix} 2 n \\ n \end{matrix})}{4^{n}} . \frac{1}{2 n + 1}

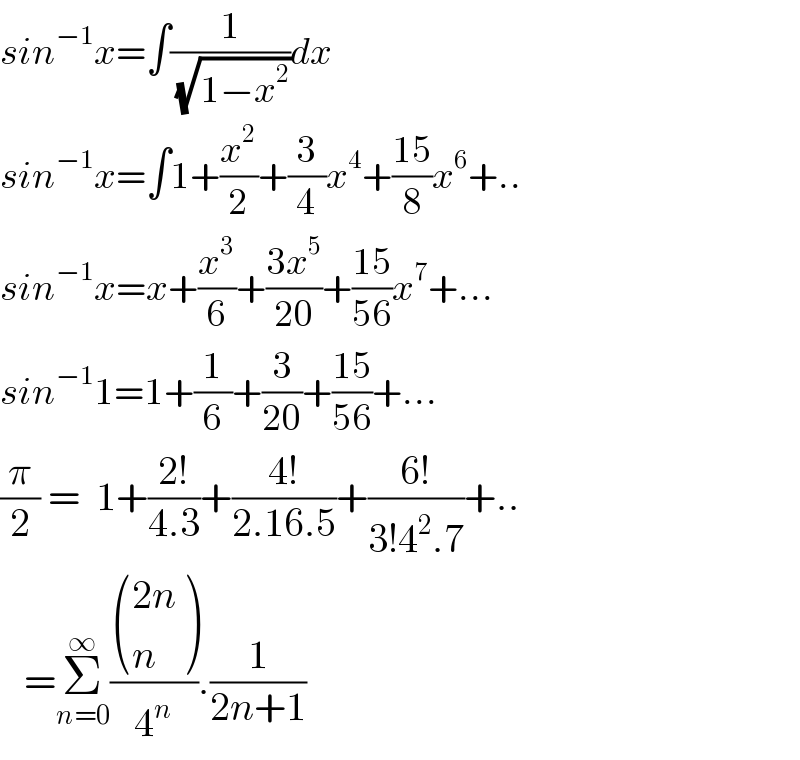
Commented by mnjuly1970 last updated on 19/Nov/20

m e r c e y m r p a y a n .

Commented by Dwaipayan Shikari last updated on 19/Nov/20

W i t h p l e a s u r e

Answered by mnjuly1970 last updated on 19/Nov/20

s o l u t i o n i i

i n (i) : 4 x = t^{2}

\frac{1}{\sqrt{1 - t^{2}}} = \underset{n = 0}{\sum^{\infty}} [(\begin{matrix} 2 n \\ n \end{matrix}) \frac{t^{2 n}}{4^{n}}]

\int_{0}^{x} \frac{1}{\sqrt{1 - t^{2}}} d t = \sum_{n ⩾ 0} {(\begin{matrix} 2 n \\ n \end{matrix}) {[\frac{t^{2 n + 1}}{(2 n + 1) 4^{n}}]}_{0}^{x}}

A r c s i n (x) = \underset{n = 0}{\sum^{\infty}} (\begin{matrix} 2 n \\ n \end{matrix}) \frac{x^{2 n + 1}}{(2 n + 1) 4^{n}}

x = 1 :: \frac{π}{2} = Σ [(\begin{matrix} 2 n \\ n \end{matrix}) \frac{1}{(2 n + 1) 4^{n}}] ✓