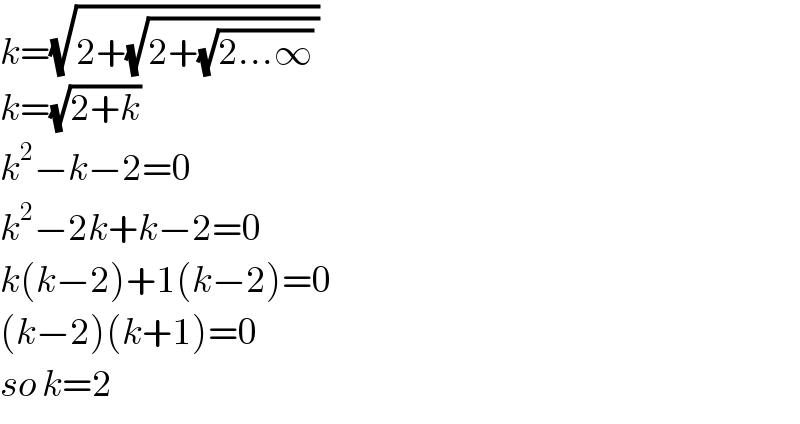Give-a-proof-for-2-2-2-2-2-2-

Question Number 49116 by hassentimol last updated on 03/Dec/18

Give a proof for :

2 = \sqrt{2 + \sqrt{2 + \sqrt{2 + \sqrt{2 + \sqrt{2 \dots}}}}}

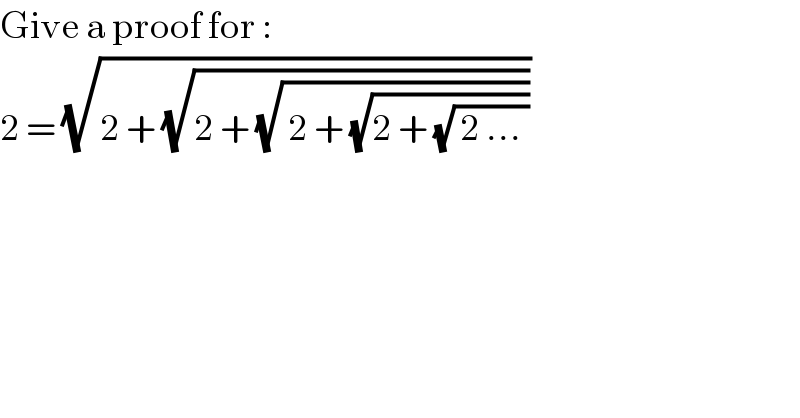
Commented by maxmathsup by imad last updated on 03/Dec/18

l e t u_{n + 1} = \sqrt{2 + u_{n}} w i t h n ⩾ 0 a n d u_{o} = \sqrt{2} \Rightarrow u_{n + 1} = f (u_{n}) w i t h f (x) = \sqrt{2 + x}

w e p r o v e b y r e c u r r e n c e t h a t u_{n} > 0 w e h a v e f o r x > 0 f^{^{'}} (x) = \frac{1}{2 \sqrt{2 + x}} > 0 s o

f i s i n c r e a s i n g o n [0, + \infty [l e t w (x) = x - f (x) \Rightarrow

w^{^{'}} (x) = 1 - f^{^{'}} (x) = 1 - \frac{1}{2 \sqrt{2 + x}} = \frac{2 \sqrt{2 + x} - 1}{2 \sqrt{2 + x}} > 0 \Rightarrow w i s i n c r e a s i n g o n [0, + \infty [

w e h a v e w (1) = 1 - f (1) = 1 - \sqrt{3} < 0 w (3) = 3 - f (3) = 3 - \sqrt{5} > 0 \Rightarrow \exists! x_{0} \in] 1, 3 [

/ w (x_{0}) = 0 w e v e r i f y t h a t x_{0} = 2 i f l = {l i m}_{n \to + \infty} u_{n} w e g e t f (l) = l \Rightarrow

l = x_{0} = 2 .

Commented by hassentimol last updated on 10/Dec/18

Thanks sir

Answered by tanmay.chaudhury50@gmail.com last updated on 03/Dec/18

k = \sqrt{2 + \sqrt{2 + \sqrt{2 \dots \infty}}}

k = \sqrt{2 + k}

k^{2} - k - 2 = 0

k^{2} - 2 k + k - 2 = 0

k (k - 2) + 1 (k - 2) = 0

(k - 2) (k + 1) = 0

s o k = 2