Question Number 121293 by abdullahquwatan last updated on 06/Nov/20
Commented by liberty last updated on 06/Nov/20
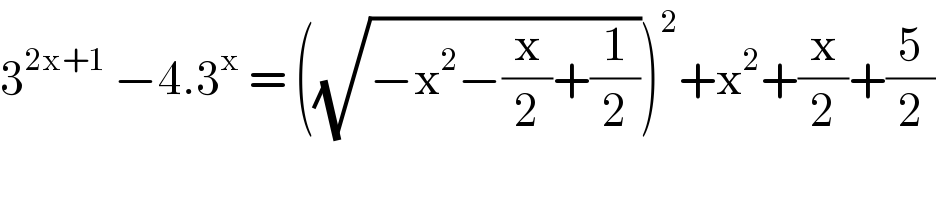
Commented by liberty last updated on 06/Nov/20
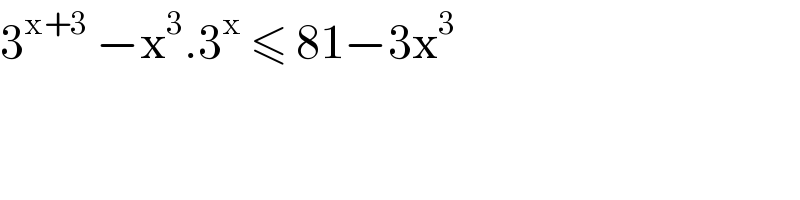
Commented by liberty last updated on 06/Nov/20
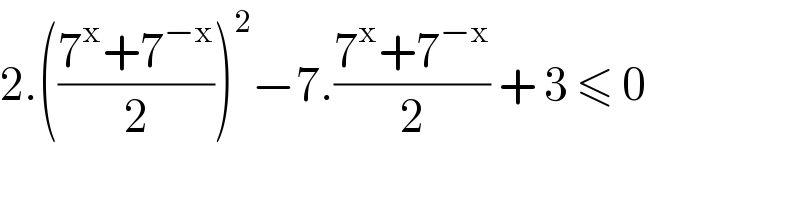
Commented by liberty last updated on 06/Nov/20
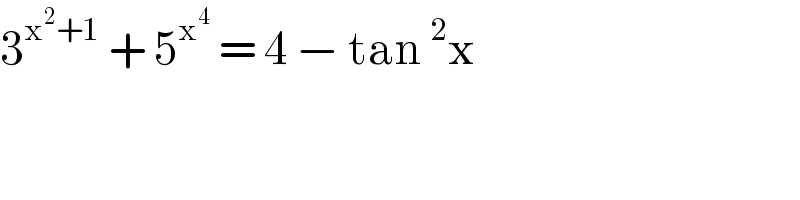
Commented by abdullahquwatan last updated on 06/Nov/20

Commented by talminator2856791 last updated on 06/Nov/20
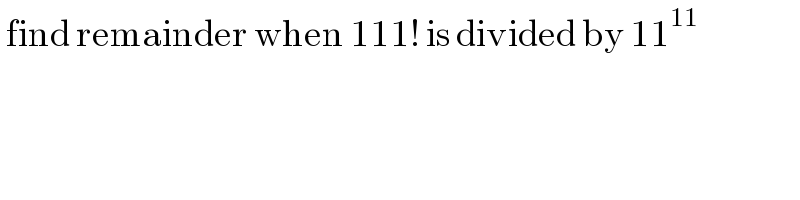
Answered by Ar Brandon last updated on 06/Nov/20

