give-the-algebric-form-of-1-i-i-

Question Number 33988 by abdo imad last updated on 28/Apr/18

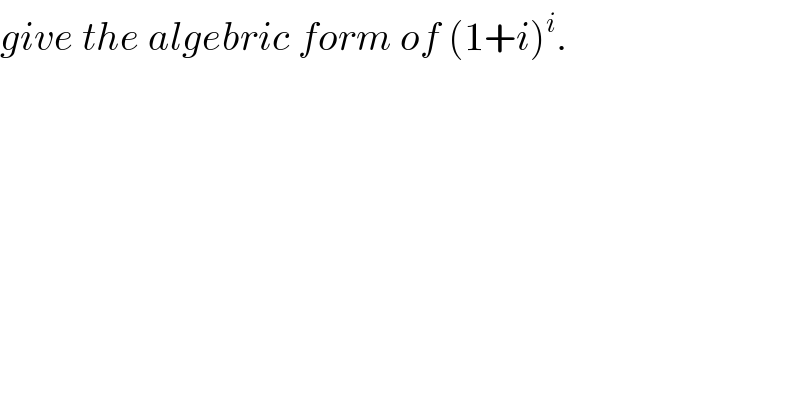
$${give}\:{the}\:{algebric}\:{form}\:{of}\:\left(\mathrm{1}+{i}\right)^{{i}} . \\ $$

Answered by MJS last updated on 28/Apr/18

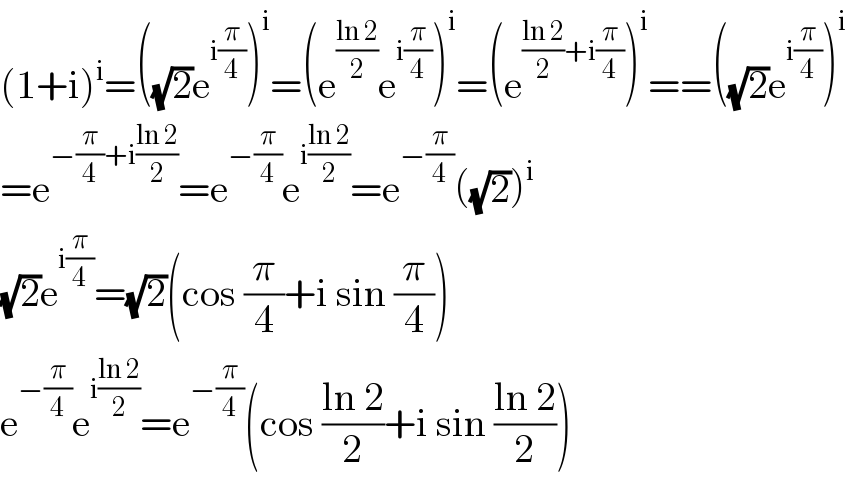
(1+i)^i =((√2)e^(i(π/4)) )^i =(e^((ln 2)/2) e^(i(π/4)) )^i =(e^(((ln 2)/2)+i(π/4)) )^i ==((√2)e^(i(π/4)) )^i =e^(−(π/4)+i((ln 2)/2)) =e^(−(π/4)) e^(i((ln 2)/2)) =e^(−(π/4)) ((√2))^i (√2)e^(i(π/4)) =(√2)(cos (π/4)+i sin (π/4)) e^(−(π/4)) e^(i((ln 2)/2)) =e^(−(π/4)) (cos ((ln 2)/2)+i sin ((ln 2)/2))

$$\left(\mathrm{1}+\mathrm{i}\right)^{\mathrm{i}} =\left(\sqrt{\mathrm{2}}\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{4}}} \right)^{\mathrm{i}} =\left(\mathrm{e}^{\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}} \mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{4}}} \right)^{\mathrm{i}} =\left(\mathrm{e}^{\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}+\mathrm{i}\frac{\pi}{\mathrm{4}}} \right)^{\mathrm{i}} ==\left(\sqrt{\mathrm{2}}\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{4}}} \right)^{\mathrm{i}} \\ $$$$=\mathrm{e}^{−\frac{\pi}{\mathrm{4}}+\mathrm{i}\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}} =\mathrm{e}^{−\frac{\pi}{\mathrm{4}}} \mathrm{e}^{\mathrm{i}\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}} =\mathrm{e}^{−\frac{\pi}{\mathrm{4}}} \left(\sqrt{\mathrm{2}}\right)^{\mathrm{i}} \\ $$$$\sqrt{\mathrm{2}}\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{4}}} =\sqrt{\mathrm{2}}\left(\mathrm{cos}\:\frac{\pi}{\mathrm{4}}+\mathrm{i}\:\mathrm{sin}\:\frac{\pi}{\mathrm{4}}\right) \\ $$$$\mathrm{e}^{−\frac{\pi}{\mathrm{4}}} \mathrm{e}^{\mathrm{i}\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}} =\mathrm{e}^{−\frac{\pi}{\mathrm{4}}} \left(\mathrm{cos}\:\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}+\mathrm{i}\:\mathrm{sin}\:\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{2}}\right) \\ $$

Facebook Tweet Pin

give-the-algebric-form-of-1-i-i-

Leave a Reply Cancel reply