Question Number 157454 by tounghoungko last updated on 23/Oct/21
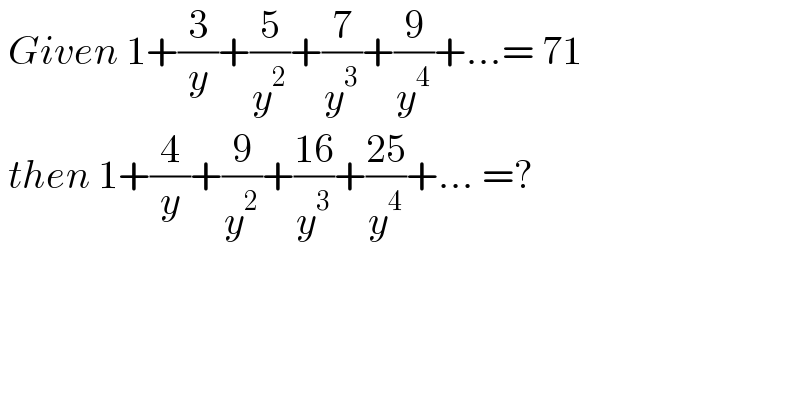
$$\:{Given}\:\mathrm{1}+\frac{\mathrm{3}}{{y}}+\frac{\mathrm{5}}{{y}^{\mathrm{2}} }+\frac{\mathrm{7}}{{y}^{\mathrm{3}} }+\frac{\mathrm{9}}{{y}^{\mathrm{4}} }+…=\:\mathrm{71} \\ $$$$\:{then}\:\mathrm{1}+\frac{\mathrm{4}}{{y}}+\frac{\mathrm{9}}{{y}^{\mathrm{2}} }+\frac{\mathrm{16}}{{y}^{\mathrm{3}} }+\frac{\mathrm{25}}{{y}^{\mathrm{4}} }+…\:=? \\ $$
Answered by FongXD last updated on 23/Oct/21
![let 1+(3/y)+(5/y^2 )+(7/y^3 )+(9/y^4 )+...=71 be equation (1) and 1+(4/y)+(9/y^2 )+((16)/y^3 )+((25)/y^4 )+...=M be equation (2) take (2)−(1): (1+(4/y)+(9/y^2 )+((16)/y^3 )+((25)/y^4 )+...)−(1+(3/y)+(5/y^2 )+(7/y^3 )+(9/y^4 )+...)=M−71 ⇔ M−71=(1/y)+(4/y^2 )+(9/y^3 )+((16)/y^4 )+((25)/y^5 )+... ⇔ M−71=(M/y), ⇒ M=((71y)/(y−1)) we have (1): 1+(3/y)+(5/y^2 )+(7/y^3 )+(9/y^4 )+...=71 ⇔ (1+(1/y)+(1/y^2 )+...)+2((1/y)+(1/y^2 )+(1/y^3 )+...)+2((1/y^2 )+(1/y^3 )+(1/y^4 )+...)+...=71 ⇔ (1/(1−(1/y)))+((2∙(1/y))/(1−(1/y)))+((2∙(1/y^2 ))/(1−(1/y)))+((2∙(1/y^3 ))/(1−(1/y)))+...=71 ⇔ −1+2(1+(1/y)+(1/y^2 )+(1/y^3 )+...)=71(1−(1/y)) ⇔ −1+(2/(1−(1/y)))=71(1−(1/y)) ⇔ 71(1−(1/y))^2 +(1+(1/y))−2=0 ⇔ 1−(1/y)=((−1+(√(569)))/(142)), [∵ 0<(1/y)<1] ⇒ y=((142)/(143−(√(569)))) we get: M=((71(((142)/(143−(√(569))))))/(((142)/(143−(√(569))))−1))=((71×142)/(142−(143−(√(569)))))=((10082)/( (√(569))−1)) ⇒ 1+(4/y)+(9/y^2 )+((16)/y^3 )+((25)/y^4 )+...=((5041((√(569))+1))/(284))](https://www.tinkutara.com/question/Q157460.png)
$$\mathrm{let}\:\mathrm{1}+\frac{\mathrm{3}}{\mathrm{y}}+\frac{\mathrm{5}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{7}}{\mathrm{y}^{\mathrm{3}} }+\frac{\mathrm{9}}{\mathrm{y}^{\mathrm{4}} }+…=\mathrm{71}\:\mathrm{be}\:\mathrm{equation}\:\left(\mathrm{1}\right) \\ $$$$\mathrm{and}\:\mathrm{1}+\frac{\mathrm{4}}{\mathrm{y}}+\frac{\mathrm{9}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{16}}{\mathrm{y}^{\mathrm{3}} }+\frac{\mathrm{25}}{\mathrm{y}^{\mathrm{4}} }+…=\mathrm{M}\:\mathrm{be}\:\mathrm{equation}\:\left(\mathrm{2}\right) \\ $$$$\mathrm{take}\:\left(\mathrm{2}\right)−\left(\mathrm{1}\right):\:\left(\mathrm{1}+\frac{\mathrm{4}}{\mathrm{y}}+\frac{\mathrm{9}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{16}}{\mathrm{y}^{\mathrm{3}} }+\frac{\mathrm{25}}{\mathrm{y}^{\mathrm{4}} }+…\right)−\left(\mathrm{1}+\frac{\mathrm{3}}{\mathrm{y}}+\frac{\mathrm{5}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{7}}{\mathrm{y}^{\mathrm{3}} }+\frac{\mathrm{9}}{\mathrm{y}^{\mathrm{4}} }+…\right)=\mathrm{M}−\mathrm{71} \\ $$$$\Leftrightarrow\:\mathrm{M}−\mathrm{71}=\frac{\mathrm{1}}{\mathrm{y}}+\frac{\mathrm{4}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{9}}{\mathrm{y}^{\mathrm{3}} }+\frac{\mathrm{16}}{\mathrm{y}^{\mathrm{4}} }+\frac{\mathrm{25}}{\mathrm{y}^{\mathrm{5}} }+… \\ $$$$\Leftrightarrow\:\mathrm{M}−\mathrm{71}=\frac{\mathrm{M}}{\mathrm{y}},\:\Rightarrow\:\mathrm{M}=\frac{\mathrm{71y}}{\mathrm{y}−\mathrm{1}} \\ $$$$\mathrm{we}\:\mathrm{have}\:\left(\mathrm{1}\right):\:\mathrm{1}+\frac{\mathrm{3}}{\mathrm{y}}+\frac{\mathrm{5}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{7}}{\mathrm{y}^{\mathrm{3}} }+\frac{\mathrm{9}}{\mathrm{y}^{\mathrm{4}} }+…=\mathrm{71} \\ $$$$\Leftrightarrow\:\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{y}}+\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{2}} }+…\right)+\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{y}}+\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{3}} }+…\right)+\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{4}} }+…\right)+…=\mathrm{71} \\ $$$$\Leftrightarrow\:\frac{\mathrm{1}}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}}+\frac{\mathrm{2}\centerdot\frac{\mathrm{1}}{\mathrm{y}}}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}}+\frac{\mathrm{2}\centerdot\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{2}} }}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}}+\frac{\mathrm{2}\centerdot\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{3}} }}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}}+…=\mathrm{71} \\ $$$$\Leftrightarrow\:−\mathrm{1}+\mathrm{2}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{y}}+\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{3}} }+…\right)=\mathrm{71}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}\right) \\ $$$$\Leftrightarrow\:−\mathrm{1}+\frac{\mathrm{2}}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}}=\mathrm{71}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}\right) \\ $$$$\Leftrightarrow\:\mathrm{71}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}\right)^{\mathrm{2}} +\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{y}}\right)−\mathrm{2}=\mathrm{0} \\ $$$$\Leftrightarrow\:\mathrm{1}−\frac{\mathrm{1}}{\mathrm{y}}=\frac{−\mathrm{1}+\sqrt{\mathrm{569}}}{\mathrm{142}},\:\left[\because\:\mathrm{0}<\frac{\mathrm{1}}{\mathrm{y}}<\mathrm{1}\right] \\ $$$$\Rightarrow\:\mathrm{y}=\frac{\mathrm{142}}{\mathrm{143}−\sqrt{\mathrm{569}}} \\ $$$$\mathrm{we}\:\mathrm{get}:\:\mathrm{M}=\frac{\mathrm{71}\left(\frac{\mathrm{142}}{\mathrm{143}−\sqrt{\mathrm{569}}}\right)}{\frac{\mathrm{142}}{\mathrm{143}−\sqrt{\mathrm{569}}}−\mathrm{1}}=\frac{\mathrm{71}×\mathrm{142}}{\mathrm{142}−\left(\mathrm{143}−\sqrt{\mathrm{569}}\right)}=\frac{\mathrm{10082}}{\:\sqrt{\mathrm{569}}−\mathrm{1}} \\ $$$$\Rightarrow\:\mathrm{1}+\frac{\mathrm{4}}{\mathrm{y}}+\frac{\mathrm{9}}{\mathrm{y}^{\mathrm{2}} }+\frac{\mathrm{16}}{\mathrm{y}^{\mathrm{3}} }+\frac{\mathrm{25}}{\mathrm{y}^{\mathrm{4}} }+…=\frac{\mathrm{5041}\left(\sqrt{\mathrm{569}}+\mathrm{1}\right)}{\mathrm{284}} \\ $$
Commented by Tawa11 last updated on 23/Oct/21

$$\mathrm{Great}\:\mathrm{sir} \\ $$
Commented by FongXD last updated on 23/Oct/21

$$\mathrm{Thanks},\:\mathrm{sir} \\ $$
Answered by qaz last updated on 23/Oct/21
![Σ_(n=0) ^∞ ((2n+1)/y^n ) ,(∣(1/y)∣<1) =(2xD+1)∣_(x=1/y) (1/(1−x)) =[((1+x)/((1−x)^2 ))]_(x=1/y) =((y(1+y))/((1−y)^2 ))=71 ⇒(1/y)=((140)/(143+(√(569)))) Σ_(n=0) ^∞ (((n+1)^2 )/y^n ) =(x^2 D^2 +3xD+1)∣_(x=1/y) (1/(1−x)) =[((2x^2 )/((1−x)^3 ))+((3x)/((1−x)^2 ))+(1/(1−x))]_(x=1/y) =[((2x^2 )/((1−x)^3 ))+(x/((1−x)^2 ))+((1+x)/((1−x)^2 ))]_(x=1/y) =[((x^2 +x)/((1−x)^3 ))]_(x=1/y) +71 =71[(x/(1−x))]_(x=1/y) +71 =((71)/4)((√(569))+1)](https://www.tinkutara.com/question/Q157472.png)
$$\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{2n}+\mathrm{1}}{\mathrm{y}^{\mathrm{n}} }\:\:\:\:\:\:\:\:,\left(\mid\frac{\mathrm{1}}{\mathrm{y}}\mid<\mathrm{1}\right) \\ $$$$=\left(\mathrm{2xD}+\mathrm{1}\right)\mid_{\mathrm{x}=\mathrm{1}/\mathrm{y}} \frac{\mathrm{1}}{\mathrm{1}−\mathrm{x}} \\ $$$$=\left[\frac{\mathrm{1}+\mathrm{x}}{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{2}} }\right]_{\mathrm{x}=\mathrm{1}/\mathrm{y}} \\ $$$$=\frac{\mathrm{y}\left(\mathrm{1}+\mathrm{y}\right)}{\left(\mathrm{1}−\mathrm{y}\right)^{\mathrm{2}} }=\mathrm{71} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{y}}=\frac{\mathrm{140}}{\mathrm{143}+\sqrt{\mathrm{569}}} \\ $$$$\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{y}^{\mathrm{n}} } \\ $$$$=\left(\mathrm{x}^{\mathrm{2}} \mathrm{D}^{\mathrm{2}} +\mathrm{3xD}+\mathrm{1}\right)\mid_{\mathrm{x}=\mathrm{1}/\mathrm{y}} \frac{\mathrm{1}}{\mathrm{1}−\mathrm{x}} \\ $$$$=\left[\frac{\mathrm{2x}^{\mathrm{2}} }{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{3}} }+\frac{\mathrm{3x}}{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{1}−\mathrm{x}}\right]_{\mathrm{x}=\mathrm{1}/\mathrm{y}} \\ $$$$=\left[\frac{\mathrm{2x}^{\mathrm{2}} }{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{3}} }+\frac{\mathrm{x}}{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{2}} }+\frac{\mathrm{1}+\mathrm{x}}{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{2}} }\right]_{\mathrm{x}=\mathrm{1}/\mathrm{y}} \\ $$$$=\left[\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{x}}{\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{3}} }\right]_{\mathrm{x}=\mathrm{1}/\mathrm{y}} +\mathrm{71} \\ $$$$=\mathrm{71}\left[\frac{\mathrm{x}}{\mathrm{1}−\mathrm{x}}\right]_{\mathrm{x}=\mathrm{1}/\mathrm{y}} +\mathrm{71} \\ $$$$=\frac{\mathrm{71}}{\mathrm{4}}\left(\sqrt{\mathrm{569}}+\mathrm{1}\right) \\ $$
Commented by tounghoungko last updated on 24/Oct/21

$${thx} \\ $$
