Given-2x-2-y-2-12y-2-7x-2-647-for-x-y-Z-Find-the-remaider-if-3x-2-y-4-divide-by-11-

Question Number 178686 by greougoury555 last updated on 20/Oct/22

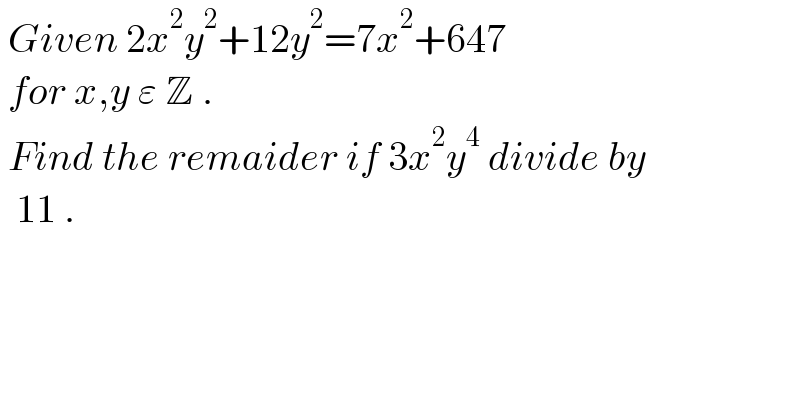
G i v e n 2 x^{2} y^{2} + 12 y^{2} = 7 x^{2} + 647

f o r x, y ε Z .

F i n d t h e r e m a i d e r i f 3 x^{2} y^{4} d i v i d e b y

11 .

Answered by Rasheed.Sindhi last updated on 20/Oct/22

2 x^{2} y^{2} + 12 y^{2} = 7 x^{2} + 647

2 y^{2} (x^{2} + 6) = 7 (x^{2} + 6) + 605

2 y^{2} (x^{2} + 6) - 7 (x^{2} + 6) = 605

(x^{2} + 6) (2 y^{2} - 7) = 5 \times 11^{2} \dots \dots A

P o s s i b l e v a l u e s f o r x^{2} + 6 :

1, 5, 11, 121, 55, 605, - 1, - 5, - 11, - 121, - 55, - 605

P o s s i b l e v a l u e s f o r x^{2}

\overset{\times}{- 5}, \overset{\times}{- 1}, \overset{\times}{5}, \overset{\times}{115}, {\begin{matrix} 49 \end{matrix}}^{✓}, \overset{\times}{599}, \overset{\times}{- 7}, \overset{\times}{- 11}, \overset{\times}{- 17}, \overset{\times}{- 127}, \overset{\times}{- 61}, \overset{\times}{- 611}

∵ x \in Z ∴ x^{2} = 49 \Rightarrow x = \pm 7

I f x^{2} + 6 = {(\pm 7)}^{2} + 6 = 55 t h e n f r o m A

2 y^{2} - 7 = 605 / 55 = 11

2 y^{2} = 18 \Rightarrow y = \pm 3

(x, y) = (\pm 7, \pm 3) o r (\pm 7, \mp 3)

3 x^{2} y^{4} = 3 {(\pm 7)}^{2} {(\pm 3)}^{4} = 3 {(\pm 7)}^{2} {(\mp 3)}^{4} = 11907

11907 (m o d 11) = \begin{matrix} 5 \end{matrix}

Commented by cortano1 last updated on 21/Oct/22

typo \Rightarrow {2 y}^{2} = 18; y = \pm 3

(x, y) = (\pm 7, \pm 3)

then {3 x}^{2} y^{4} = 3 \times 49 \times 81 = 11907

11907 = 1082 \times 11 + 5 = 5 (\mod 11)

Commented by Rasheed.Sindhi last updated on 20/Oct/22

T h a n k y o u s i r, I^{'} v e c o r r e c t e d n o w .

Answered by greougoury555 last updated on 21/Oct/22

2 x^{2} y^{2} - 7 x^{2} = 647 - 12 y^{2}

x^{2} (2 y^{2} - 7) = 605 + 42 - 12 y^{2}

x^{2} (2 y^{2} - 7) = 605 + 6 (7 - 2 y^{2)}

x^{2} (2 y^{2} - 7) + 6 (2 y^{2} - 7) = 605

(2 y^{2} - 7) (x^{2} + 6) = 11 \times 55

{\begin{cases} 2 y^{2} - 7 = 11 \Rightarrow y^{2} = 9 \\ x^{2} + 6 = 55 \Rightarrow x = 49 \end{cases}

\Rightarrow 3 x^{2} y^{4} = 3 \times 49 \times 81 = 11097

\Rightarrow \frac{11097}{11} = 1082 + \frac{5}{11}

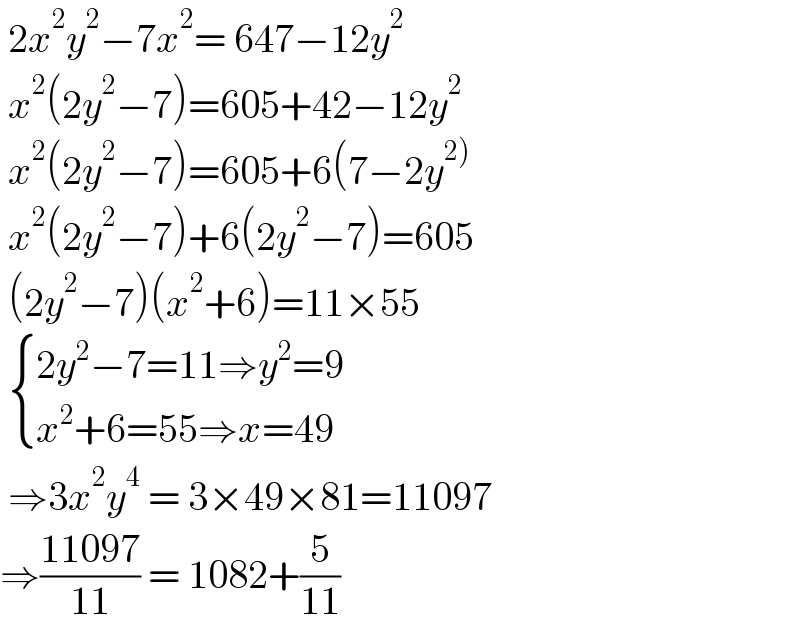
Commented by Rasheed.Sindhi last updated on 21/Oct/22

T y p o : 3 \times 49 \times 81 = 11907