Question Number 180014 by cortano1 last updated on 06/Nov/22
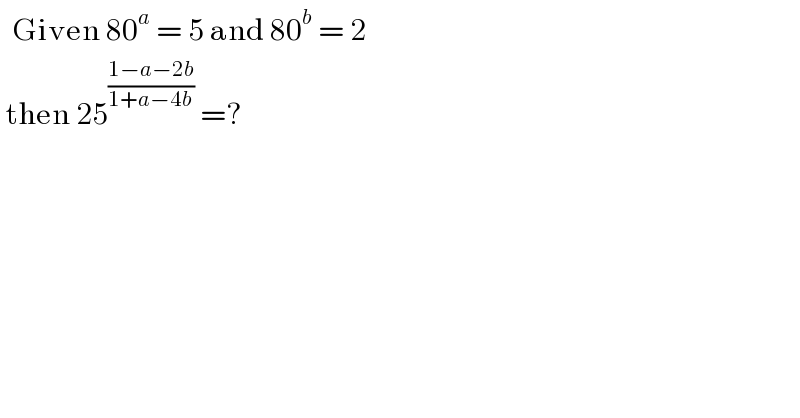
Answered by srikanth2684 last updated on 06/Nov/22
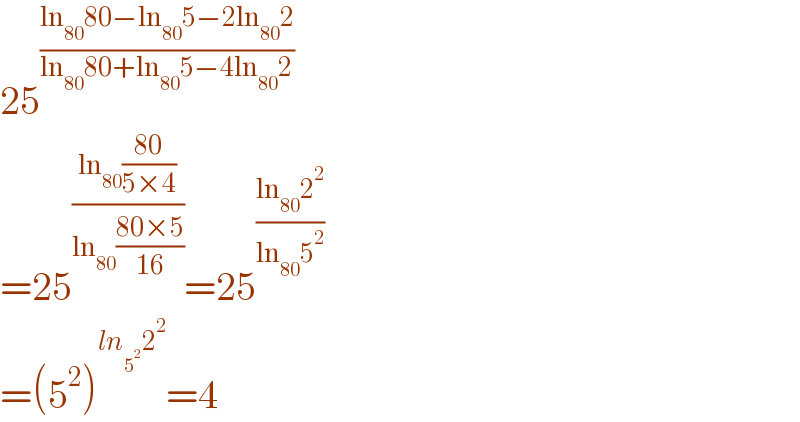
Commented by cortano1 last updated on 06/Nov/22

Answered by a.lgnaoui last updated on 06/Nov/22
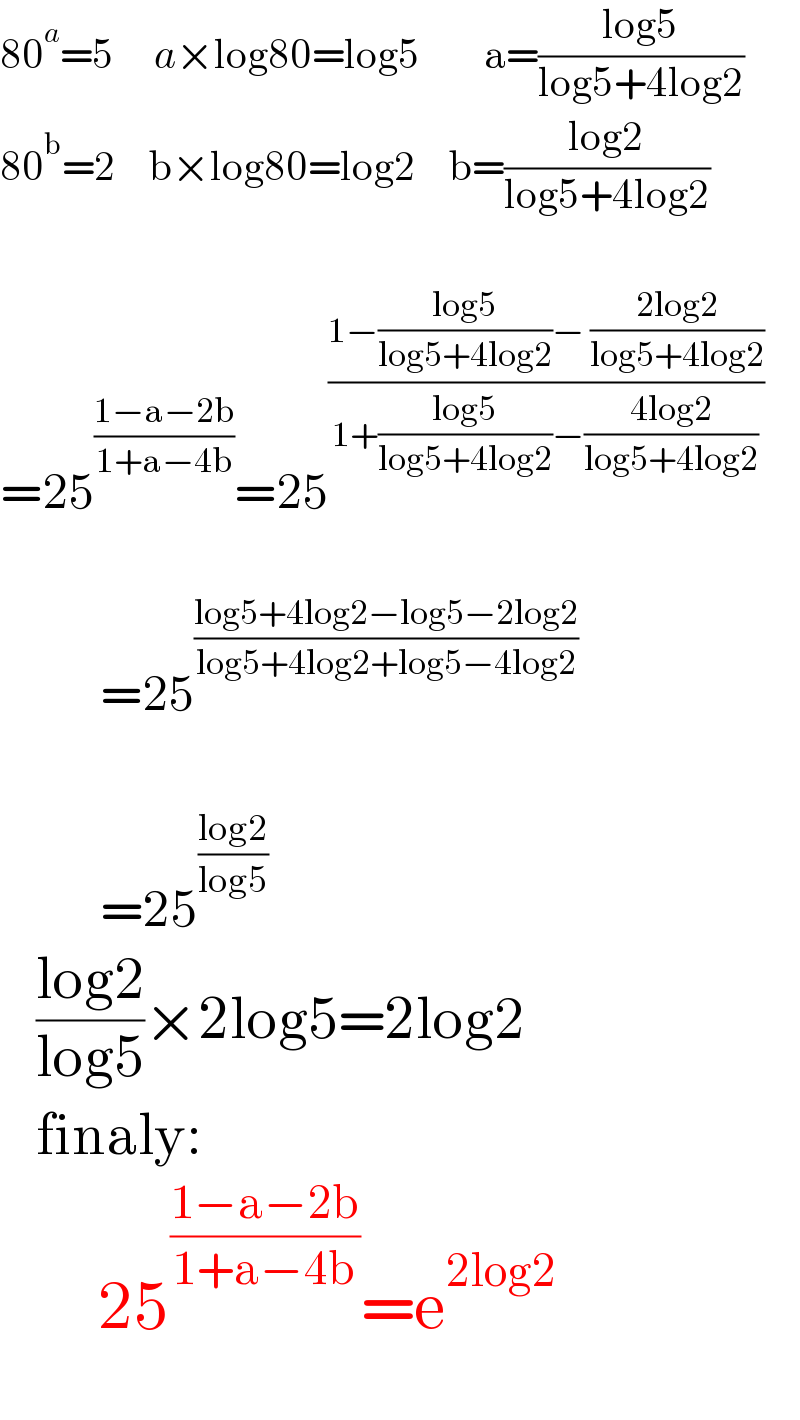
Commented by a.lgnaoui last updated on 06/Nov/22
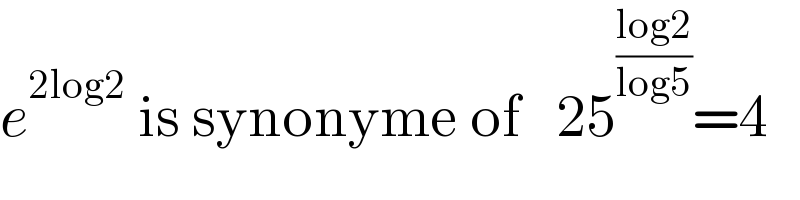
Commented by a.lgnaoui last updated on 06/Nov/22
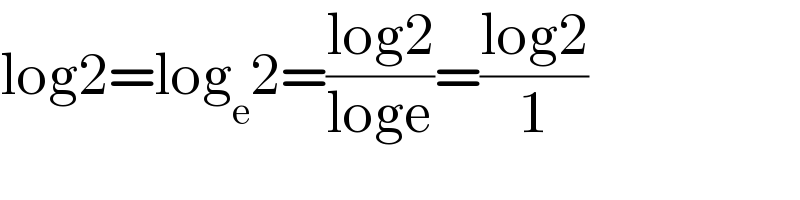
Commented by a.lgnaoui last updated on 06/Nov/22

Commented by cortano1 last updated on 06/Nov/22

