Question Number 101937 by 1549442205 last updated on 06/Jul/20
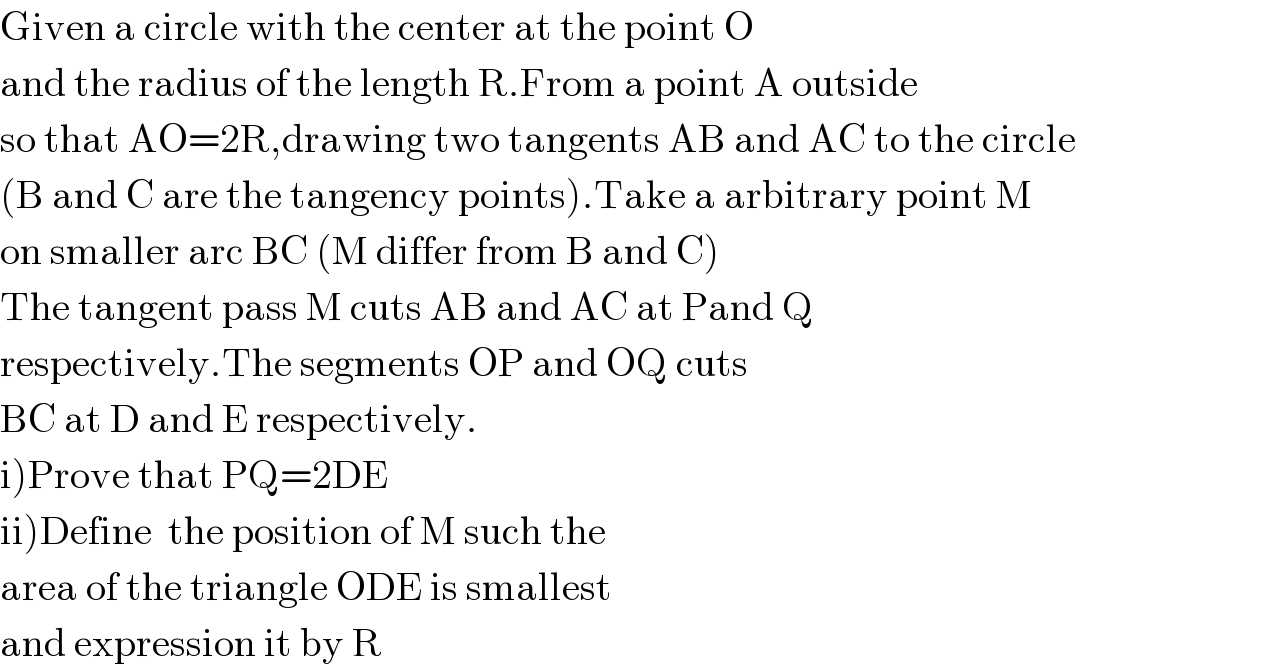
$$\mathrm{Given}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{with}\:\mathrm{the}\:\mathrm{center}\:\mathrm{at}\:\mathrm{the}\:\mathrm{point}\:\mathrm{O}\: \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:\mathrm{the}\:\mathrm{length}\:\mathrm{R}.\mathrm{From}\:\mathrm{a}\:\mathrm{point}\:\mathrm{A}\:\mathrm{outside} \\ $$$$\mathrm{so}\:\mathrm{that}\:\mathrm{AO}=\mathrm{2R},\mathrm{drawing}\:\mathrm{two}\:\mathrm{tangents}\:\mathrm{AB}\:\mathrm{and}\:\mathrm{AC}\:\mathrm{to}\:\mathrm{the}\:\mathrm{circle} \\ $$$$\left(\mathrm{B}\:\mathrm{and}\:\mathrm{C}\:\mathrm{are}\:\mathrm{the}\:\mathrm{tangency}\:\mathrm{points}\right).\mathrm{Take}\:\mathrm{a}\:\mathrm{arbitrary}\:\mathrm{point}\:\mathrm{M} \\ $$$$\mathrm{on}\:\mathrm{smaller}\:\mathrm{arc}\:\mathrm{BC}\:\left(\mathrm{M}\:\mathrm{differ}\:\mathrm{from}\:\mathrm{B}\:\mathrm{and}\:\mathrm{C}\right) \\ $$$$\mathrm{The}\:\mathrm{tangent}\:\mathrm{pass}\:\mathrm{M}\:\mathrm{cuts}\:\mathrm{AB}\:\mathrm{and}\:\mathrm{AC}\:\mathrm{at}\:\mathrm{Pand}\:\mathrm{Q} \\ $$$$\mathrm{respectively}.\mathrm{The}\:\mathrm{segments}\:\mathrm{OP}\:\mathrm{and}\:\mathrm{OQ}\:\mathrm{cuts} \\ $$$$\mathrm{BC}\:\mathrm{at}\:\mathrm{D}\:\mathrm{and}\:\mathrm{E}\:\mathrm{respectively}. \\ $$$$\left.\mathrm{i}\right)\mathrm{Prove}\:\mathrm{that}\:\mathrm{PQ}=\mathrm{2DE} \\ $$$$\left.\mathrm{ii}\right)\mathrm{Define}\:\:\mathrm{the}\:\mathrm{position}\:\mathrm{of}\:\mathrm{M}\:\mathrm{such}\:\mathrm{the}\: \\ $$$$\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{triangle}\:\mathrm{ODE}\:\mathrm{is}\:\mathrm{smallest} \\ $$$$\mathrm{and}\:\mathrm{expression}\:\mathrm{it}\:\mathrm{by}\:\mathrm{R} \\ $$
