Question Number 167925 by naka3546 last updated on 29/Mar/22
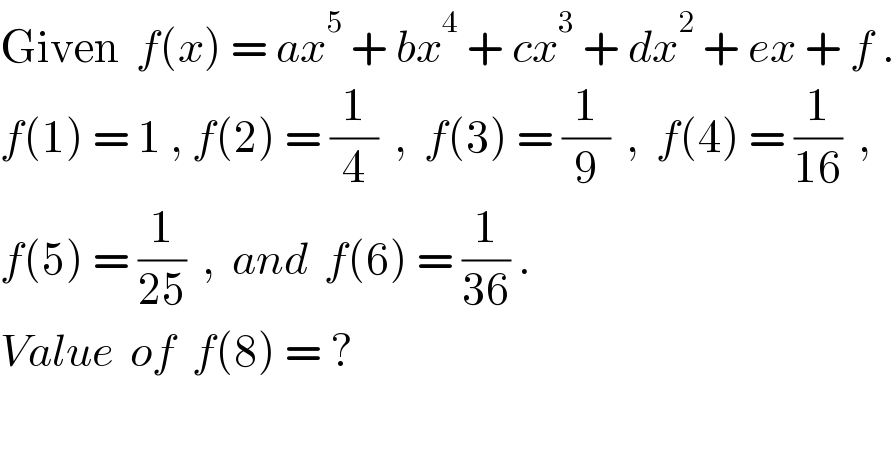
Answered by mr W last updated on 29/Mar/22
![f(8)= [(1,1,1,1,1,1,1),((32),(16),8,4,2,1,(1/4)),((243),(81),(27),9,3,1,(1/9)),((1024),(256),(64),(16),4,1,(1/16)),((3125),(625),(125),(25),5,1,(1/25)),((7776),(1296),(216),(36),6,1,(1/36)),((32768),(4096),(512),(64),8,1,0) ]/ [(1,1,1,1,1,1,0),((32),(16),8,4,2,1,0),((243),(81),(27),9,3,1,0),((1024),(256),(64),(16),4,1,0),((3125),(625),(125),(25),5,1,0),((7776),(1296),(216),(36),6,1,0),((32768),(4096),(512),(64),8,1,(−1)) ] =−((77328)/(34560)) =−((179)/(80))](https://www.tinkutara.com/question/Q167935.png)
Commented by naka3546 last updated on 29/Mar/22
Commented by Tawa11 last updated on 30/Mar/22

Commented by mr W last updated on 30/Mar/22
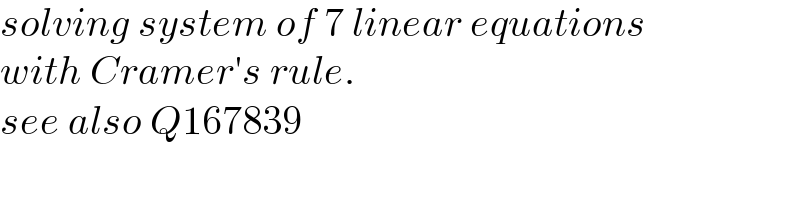
Commented by mr W last updated on 30/Mar/22
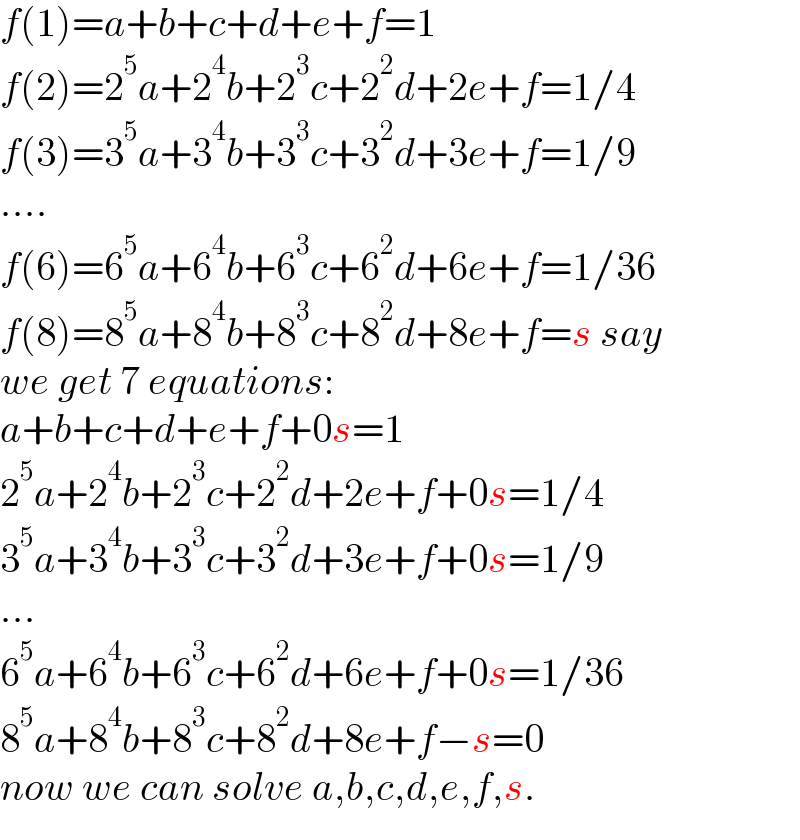
Commented by Tawa11 last updated on 30/Mar/22