Menu Close

given-vertex-parabola-at-point-1-2-and-focus-at-1-2-find-the-equation-of-parabola-

Tinku Tara 0 Comments
Pin




Question Number 115846 by bemath last updated on 29/Sep/20
given vertex parabola at point (1,−2) and focus at (−1,−2). find the equation of parabola
$${given}\:{vertex}\:{parabola}\:{at}\:{point} \\ $$$$\left(\mathrm{1},−\mathrm{2}\right)\:{and}\:{focus}\:{at}\:\left(−\mathrm{1},−\mathrm{2}\right). \\ $$$${find}\:{the}\:{equation}\:{of}\:{parabola} \\ $$
Commented by bemath last updated on 29/Sep/20
thank you mr PVT and bob
$${thank}\:{you}\:{mr}\:{PVT}\:{and}\:{bob} \\ $$
Answered by 1549442205PVT last updated on 29/Sep/20
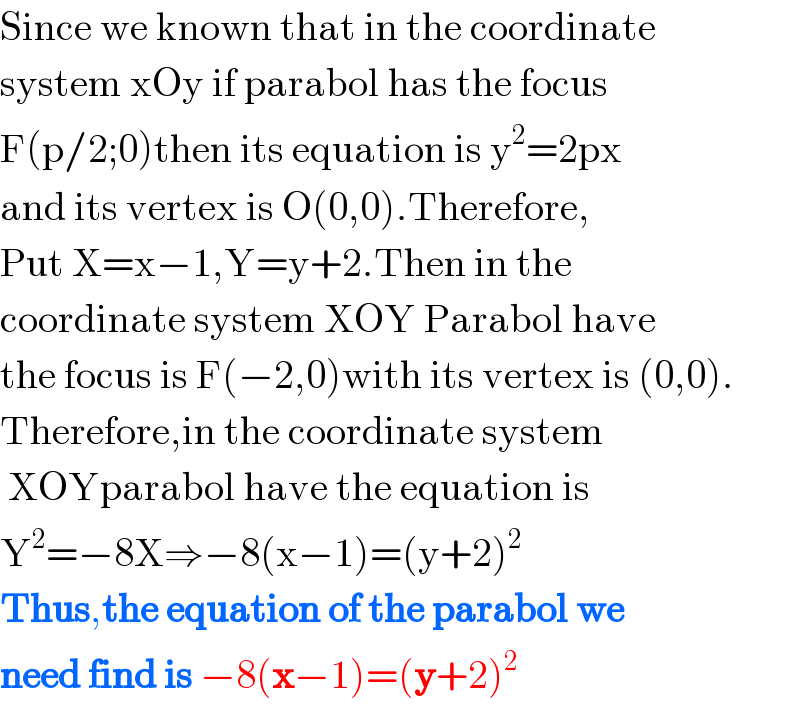
Since we known that in the coordinate system xOy if parabol has the focus F(p/2;0)then its equation is y^2 =2px and its vertex is O(0,0).Therefore, Put X=x−1,Y=y+2.Then in the coordinate system XOY Parabol have the focus is F(−2,0)with its vertex is (0,0). Therefore,in the coordinate system XOYparabol have the equation is Y^2 =−8X⇒−8(x−1)=(y+2)^2 Thus,the equation of the parabol we need find is −8(x−1)=(y+2)^2
$$\mathrm{Since}\:\mathrm{we}\:\mathrm{known}\:\mathrm{that}\:\mathrm{in}\:\mathrm{the}\:\mathrm{coordinate} \\ $$$$\mathrm{system}\:\mathrm{xOy}\:\mathrm{if}\:\mathrm{parabol}\:\mathrm{has}\:\mathrm{the}\:\mathrm{focus} \\ $$$$\mathrm{F}\left(\mathrm{p}/\mathrm{2};\mathrm{0}\right)\mathrm{then}\:\mathrm{its}\:\mathrm{equation}\:\mathrm{is}\:\mathrm{y}^{\mathrm{2}} =\mathrm{2px} \\ $$$$\mathrm{and}\:\mathrm{its}\:\mathrm{vertex}\:\mathrm{is}\:\mathrm{O}\left(\mathrm{0},\mathrm{0}\right).\mathrm{Therefore}, \\ $$$$\mathrm{Put}\:\mathrm{X}=\mathrm{x}−\mathrm{1},\mathrm{Y}=\mathrm{y}+\mathrm{2}.\mathrm{Then}\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{coordinate}\:\mathrm{system}\:\mathrm{XOY}\:\mathrm{Parabol}\:\mathrm{have} \\ $$$$\mathrm{the}\:\mathrm{focus}\:\mathrm{is}\:\mathrm{F}\left(−\mathrm{2},\mathrm{0}\right)\mathrm{with}\:\mathrm{its}\:\mathrm{vertex}\:\mathrm{is}\:\left(\mathrm{0},\mathrm{0}\right). \\ $$$$\mathrm{Therefore},\mathrm{in}\:\mathrm{the}\:\mathrm{coordinate}\:\mathrm{system} \\ $$$$\:\mathrm{XOYparabol}\:\mathrm{have}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{is} \\ $$$$\mathrm{Y}^{\mathrm{2}} =−\mathrm{8X}\Rightarrow−\mathrm{8}\left(\mathrm{x}−\mathrm{1}\right)=\left(\mathrm{y}+\mathrm{2}\right)^{\mathrm{2}} \: \\ $$$$\boldsymbol{\mathrm{Thus}},\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{equation}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{parabol}}\:\boldsymbol{\mathrm{we}} \\ $$$$\boldsymbol{\mathrm{need}}\:\boldsymbol{\mathrm{find}}\:\boldsymbol{\mathrm{is}}\:−\mathrm{8}\left(\boldsymbol{\mathrm{x}}−\mathrm{1}\right)=\left(\boldsymbol{\mathrm{y}}+\mathrm{2}\right)^{\mathrm{2}} \:\: \\ $$
Answered by bobhans last updated on 29/Sep/20
The line connecting the vertex and the focus is the axis and has equation y=−2 .The directrix is the line orthogonal to the axis, with a distance from the vertex equal to the distance between vertex and focus. (√((x+1)^2 +(y+2)^2 )) = ∣x−3∣ (x+1)^2 +(y+2)^2 = (x−3)^2 ⇔(y+2)^2 = (x−3)^2 −(x+1)^2 ⇔(y+2)^2 = (2x−2)(−4) ⇔(y+2)^2 = −8(x−1)
$${The}\:{line}\:{connecting}\:{the}\:{vertex}\:{and}\:{the} \\ $$$${focus}\:{is}\:{the}\:{axis}\:{and}\:{has}\:{equation}\:{y}=−\mathrm{2} \\ $$$$.{The}\:{directrix}\:{is}\:{the}\:{line}\:{orthogonal} \\ $$$${to}\:{the}\:{axis},\:{with}\:{a}\:{distance}\:{from}\:{the}\: \\ $$$${vertex}\:{equal}\:{to}\:{the}\:{distance}\:{between} \\ $$$${vertex}\:{and}\:{focus}. \\ $$$$\sqrt{\left({x}+\mathrm{1}\right)^{\mathrm{2}} +\left({y}+\mathrm{2}\right)^{\mathrm{2}} }\:=\:\mid{x}−\mathrm{3}\mid\: \\ $$$$\left({x}+\mathrm{1}\right)^{\mathrm{2}} +\left({y}+\mathrm{2}\right)^{\mathrm{2}} \:=\:\left({x}−\mathrm{3}\right)^{\mathrm{2}} \\ $$$$\Leftrightarrow\left({y}+\mathrm{2}\right)^{\mathrm{2}} \:=\:\left({x}−\mathrm{3}\right)^{\mathrm{2}} −\left({x}+\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\Leftrightarrow\left({y}+\mathrm{2}\right)^{\mathrm{2}} \:=\:\left(\mathrm{2}{x}−\mathrm{2}\right)\left(−\mathrm{4}\right) \\ $$$$\Leftrightarrow\left({y}+\mathrm{2}\right)^{\mathrm{2}} \:=\:−\mathrm{8}\left({x}−\mathrm{1}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *