Question Number 102094 by M±th+et+s last updated on 06/Jul/20

$${good}\:{evenig}\:{for}\:{all} \\ $$$$ \\ $$$${this}\:{is}\:{an}\:{answerd}\:{question}\:{i}\:{will}\:{repost}\:{it} \\ $$$${if}: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{f}\left({x}\right)=\sqrt{{x}−\mathrm{2}} \\ $$$${is}\:{there}\:{a}\:{cirtical}\:{point}\:{when}\:{x}=\mathrm{2}\:? \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by PRITHWISH SEN 2 last updated on 06/Jul/20

$$\mathrm{No} \\ $$
Commented by M±th+et+s last updated on 06/Jul/20

Commented by M±th+et+s last updated on 06/Jul/20

Commented by M±th+et+s last updated on 06/Jul/20
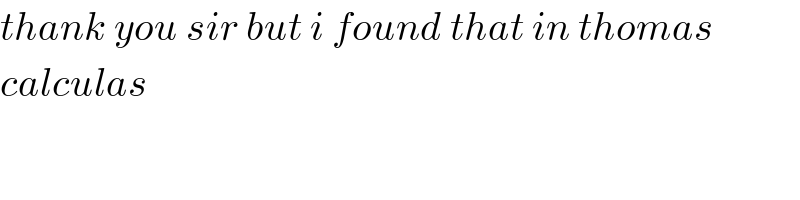
$${thank}\:{you}\:{sir}\:{but}\:{i}\:{found}\:{that}\:{in}\:{thomas}\: \\ $$$${calculas}\: \\ $$
Commented by PRITHWISH SEN 2 last updated on 06/Jul/20

$$\mathrm{value}\:\mathrm{of}\:\mathrm{x}\:\mathrm{at}\:\mathrm{f}^{'} \left(\mathrm{x}\right)=\mathrm{0}\:\mathrm{implies}\:\mathrm{the}\:\mathrm{exsistence}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{critical}\:\mathrm{point}\: \\ $$$$\mathrm{butin}\:\mathrm{this}\:\mathrm{case}\:\mathrm{f}^{'} \left(\mathrm{x}\right)\:\neq\mathrm{0}\:\mathrm{for}\:\mathrm{x}=\mathrm{2} \\ $$$$\mathrm{and}\:\mathrm{another}\:\mathrm{point}\:\mathrm{is}\:\mathrm{f}'\left(\mathrm{x}\right)\:\mathrm{must}\:\mathrm{be}\:\mathrm{atleast}\: \\ $$$$\mathrm{continuous}\:\mathrm{on}\:\mathrm{that}\:\mathrm{point}. \\ $$
