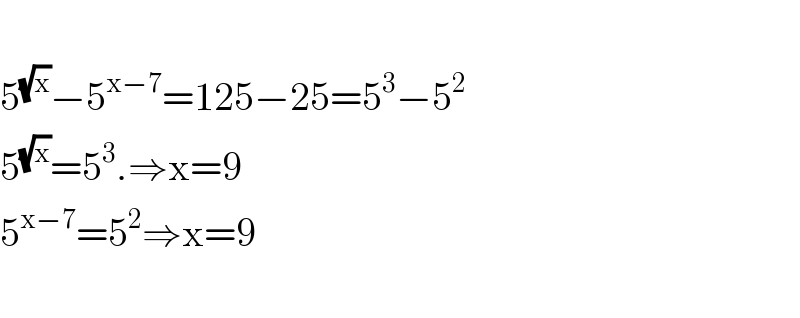Question Number 41707 by Necxx last updated on 11/Aug/18
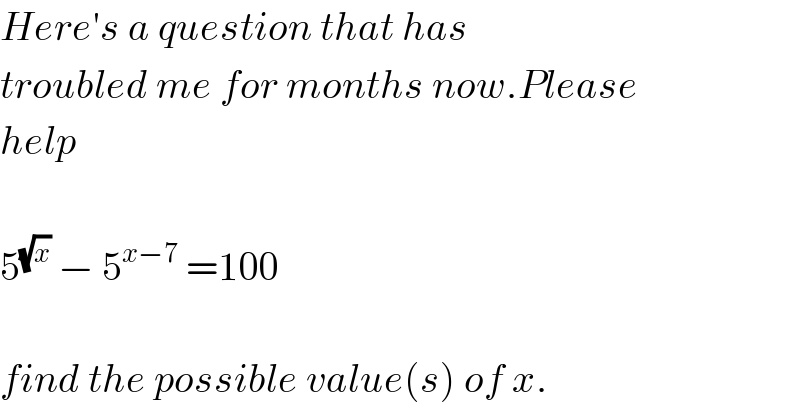
Answered by alex041103 last updated on 11/Aug/18

Commented by alex041103 last updated on 11/Aug/18
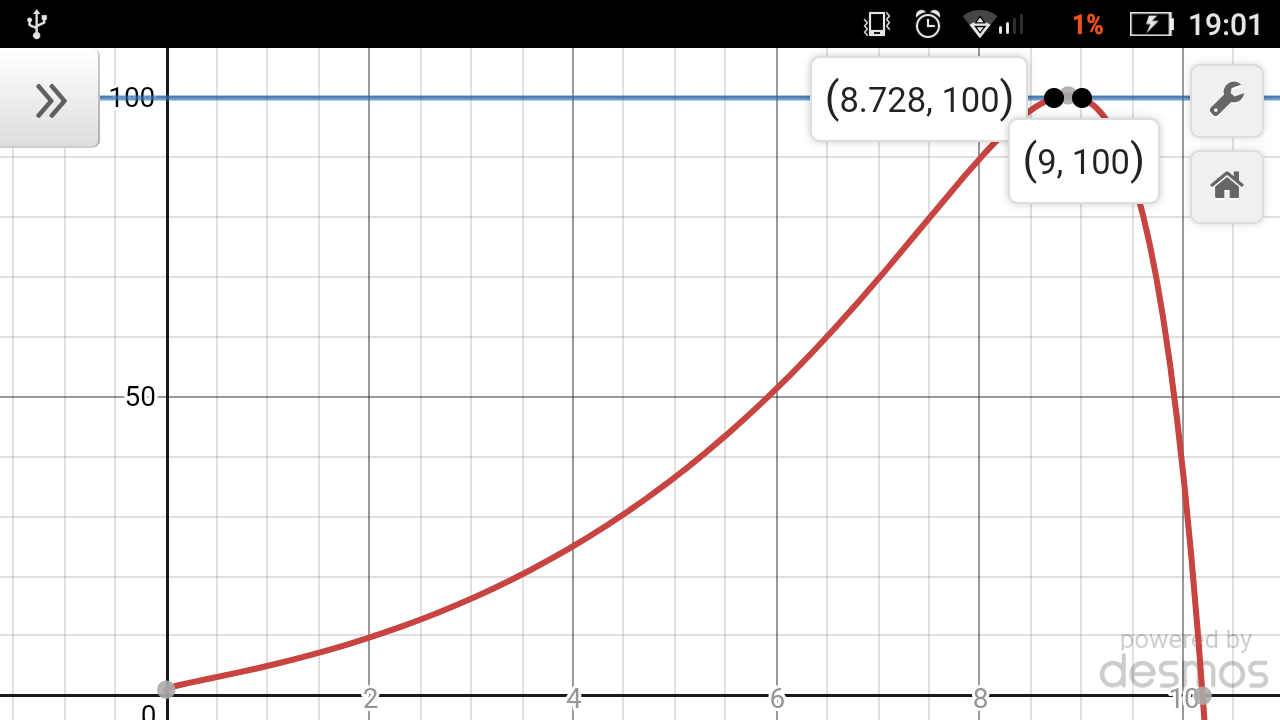
Commented by alex041103 last updated on 11/Aug/18
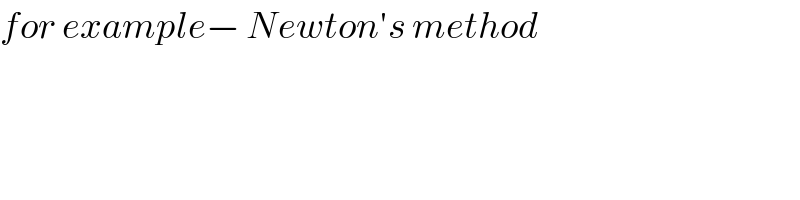
Commented by Necxx last updated on 11/Aug/18
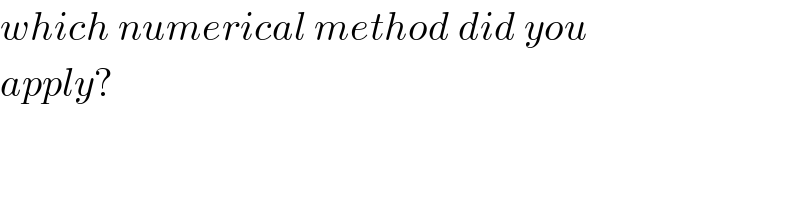
Commented by Necxx last updated on 11/Aug/18

Answered by peter frank last updated on 04/Oct/18