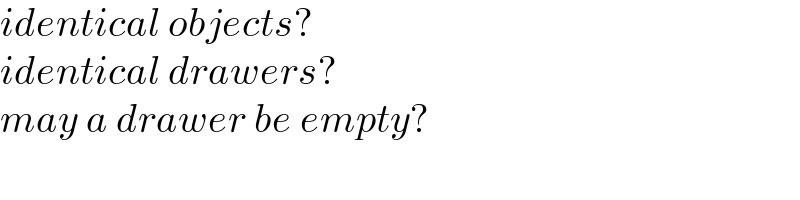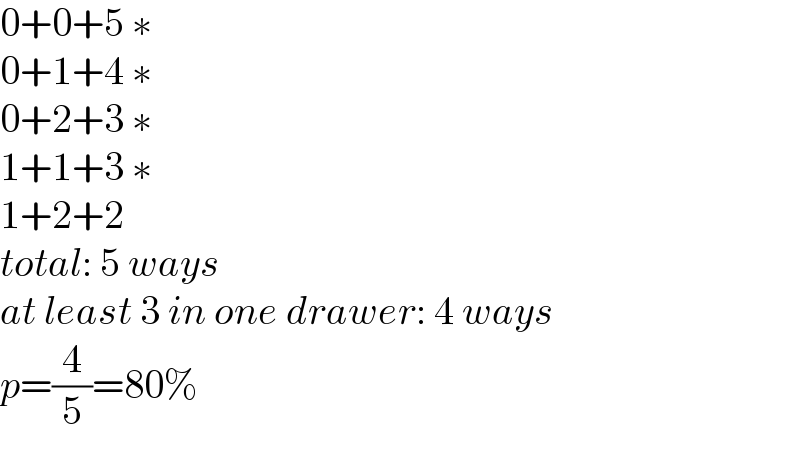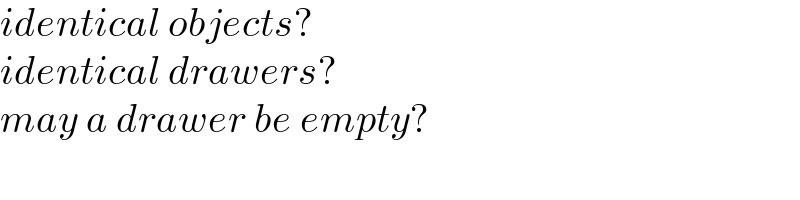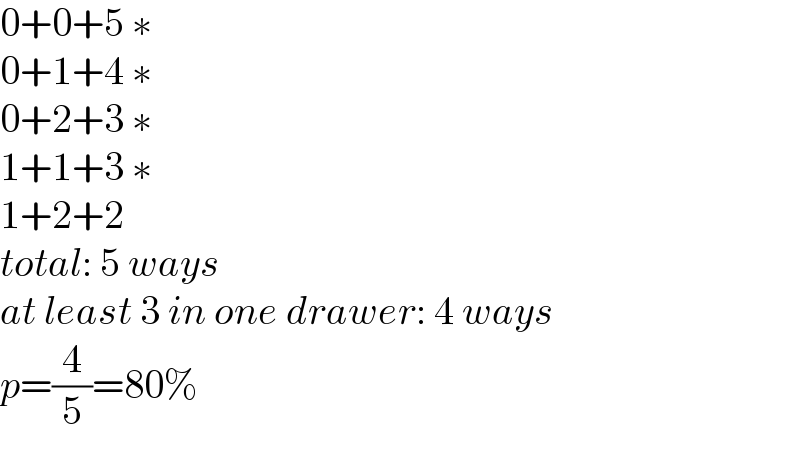Question Number 163283 by greg_ed last updated on 05/Jan/22
We store 5 objects in three discernible drawers. Suppose that the different ways of carrying out these arrangements are equally probable, calculate the probability that one of the 3 drawers contains at least 3 objects.
Commented by mr W last updated on 05/Jan/22
Answered by mr W last updated on 06/Jan/22