Question Number 80113 by jagoll last updated on 31/Jan/20
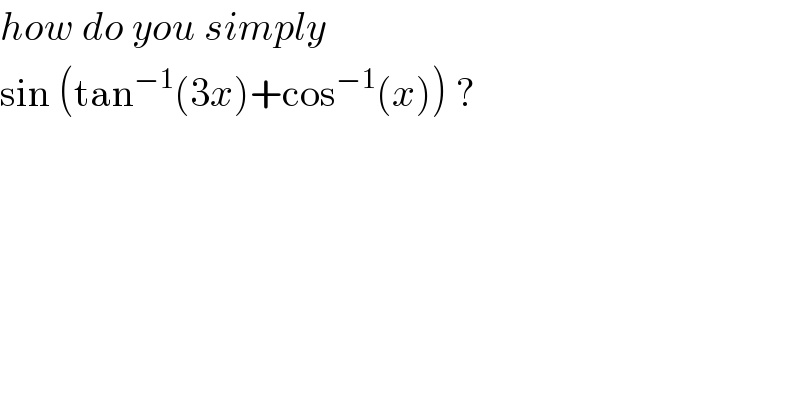
$${how}\:{do}\:{you}\:{simply} \\ $$$$\mathrm{sin}\:\left(\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{3}{x}\right)+\mathrm{cos}^{−\mathrm{1}} \left({x}\right)\right)\:? \\ $$
Commented by john santu last updated on 31/Jan/20
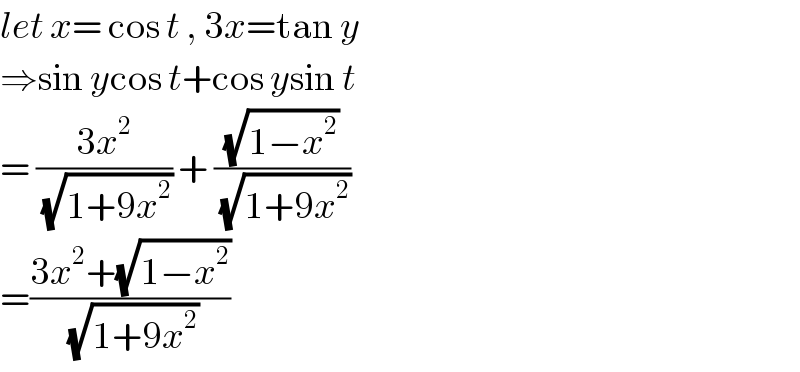
$${let}\:{x}=\:\mathrm{cos}\:{t}\:,\:\mathrm{3}{x}=\mathrm{tan}\:{y} \\ $$$$\Rightarrow\mathrm{sin}\:{y}\mathrm{cos}\:{t}+\mathrm{cos}\:{y}\mathrm{sin}\:{t}\: \\ $$$$=\:\frac{\mathrm{3}{x}^{\mathrm{2}} }{\:\sqrt{\mathrm{1}+\mathrm{9}{x}^{\mathrm{2}} }}\:+\:\frac{\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+\mathrm{9}{x}^{\mathrm{2}} }} \\ $$$$=\frac{\mathrm{3}{x}^{\mathrm{2}} +\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+\mathrm{9}{x}^{\mathrm{2}} }}\: \\ $$
