How-many-4-letters-word-can-be-formed-from-COMMUNICATION-

Question Number 130019 by I want to learn more last updated on 21/Jan/21

How many 4 letters word can be formed from COMMUNICATION

Answered by mr W last updated on 22/Jan/21

2 \times C

2 \times O

2 \times M

2 \times N

2 \times I

1 \times U

1 \times A

1 \times T

c a s e 1 : W X Y Z

4! {(1 + x)}^{5} {(1 + x)}^{3} = \dots 1680 x^{4} \dots

o r C_{4}^{8} \times 4! = 1680

c a s e 2 : X Y Y Z

\frac{4!}{2!} \times C_{1}^{5} \times x^{2} {(1 + x)}^{4} {(1 + x)}^{3} = \dots 1260 x^{4} \dots

o r C_{1}^{5} \times C_{2}^{7} \times \frac{4!}{2!} = 1260

c a s e 3 : X X Y Y

\frac{4!}{2! 2!} \times C_{2}^{5} \times x^{2} \times x^{2} {(1 + x)}^{3} {(1 + x)}^{3} = \dots 60 x^{4} \dots

o r C_{2}^{5} \times \frac{4!}{2! 2!} = 60

t o t a l 1680 + 1260 + 60 = 3000 w o r d s

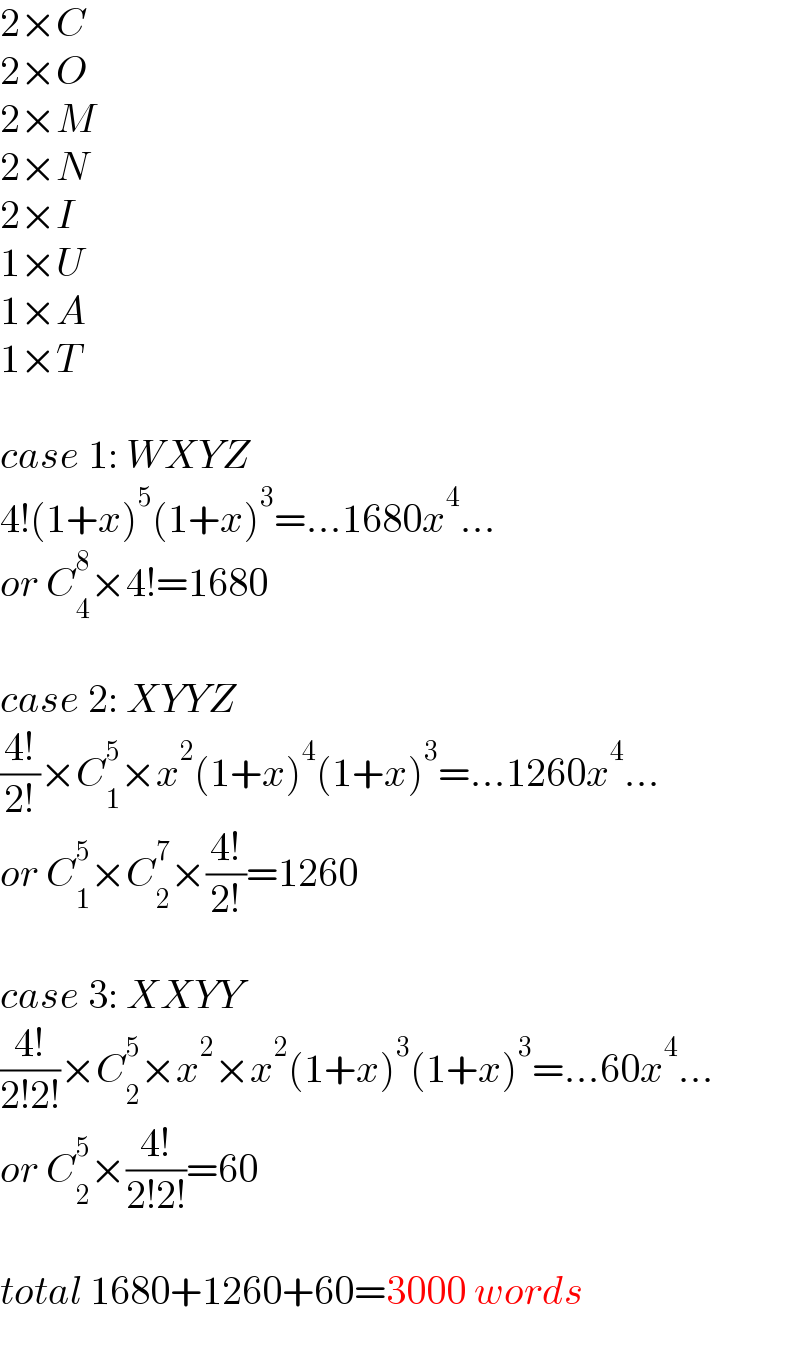
Commented by I want to learn more last updated on 22/Jan/21

Thanks sir, i appreciate .

Commented by I want to learn more last updated on 23/Jan/21

Sir that is coefficient of x^{4} in 4! {(1 + \frac{x}{1!} + \frac{x^{2}}{2!})}^{5} {(1 + x)}^{3}

Thanks sir

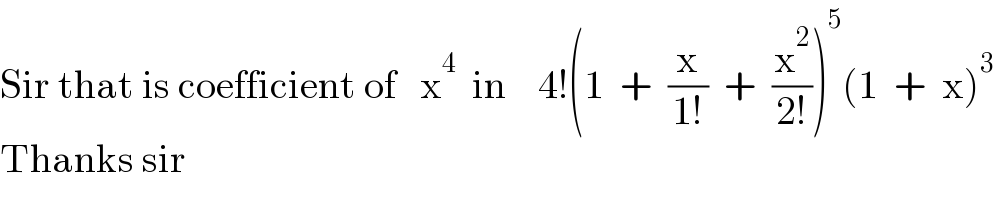
Commented by mr W last updated on 23/Jan/21

y e s .

Commented by I want to learn more last updated on 23/Jan/21

Thanks sir .

Commented by mr W last updated on 24/Jan/21

s e e a l s o Q 130193

Facebook Tweet Pin